Question

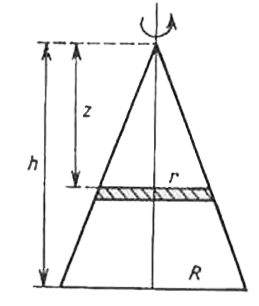
Derive, making use of an integral, the formula for the moment of inertia of a right circular cone about its height.

Answer

`0.3 MR^2` [ Hint . Divide the body into thin disks perpendicular to the axis of rotation]

Explanation

For a cone (Fig) `dm=(Mr^2dz)/(1//3hR^2), r = (Rz)/(h) , dI = (dm.r^2)/(2)=(3MR^2z^4dz)/(2h^5)`
`I=int_0^h(3MR^2z^4dz)/(2h^5)=(3MR^2)/(2h^5)int_0^hz^4dz.=(3MR^2h^2)/(2h^5xx5)=0.3MR^2`