There are many points on the circumference of a circle such that distance between each consecutive point is 11cm and time taken to move from one point to next is 45 minute find the radius of circle and no. of points.
The correct Answer is:480
To solve the problem, we need to find the radius of the circle and the number of points on its circumference given that the distance between each consecutive point is 11 cm and the time taken to move from one point to the next is 45 minutes.
Step 1: Understand the relationship between the circumference, radius, and number of points
The circumference C of a circle is given by the formula:
C=2πr
where r is the radius of the circle.
If there are n points on the circumference, and the distance between each consecutive point is 11 cm, then the total distance around the circle (the circumference) can also be expressed as:
C=n×11
Step 2: Set the two expressions for circumference equal to each other
From the two expressions for circumference, we can set them equal:
2πr=n×11
Step 3: Solve for n in terms of r
Rearranging the equation gives:
n=2πr11
Step 4: Substitute the value of π
Using π≈227, we substitute this value into the equation:
n=2×227×r11
Step 5: Simplify the equation
This simplifies to:
n=44r77
n=4r7
Step 6: Determine the relationship between n and r
From the equation n=4r7, we can express r in terms of n:
r=7n4
Step 7: Find integer values for n
Since n must be a whole number (you can't have a fraction of a point), n must be a multiple of 4. The smallest value for n that satisfies this is 4.
Step 8: Calculate the radius using n=4
Substituting n=4 back into the equation for r:
r=7×44=7 cm
Conclusion
Thus, the radius of the circle is 7 cm and the number of points on the circumference is 4.
Summary of the Solution
- Radius of the circle: 7 cm
- Number of points: 4
The circumference C of a circle is given by the formula:
C=2πr
where r is the radius of the circle.
C=n×11
From the two expressions for circumference, we can set them equal:
2πr=n×11
Rearranging the equation gives:
n=2πr11
Using π≈227, we substitute this value into the equation:
n=2×227×r11
This simplifies to:
n=44r77
n=4r7
From the equation n=4r7, we can express r in terms of n:
r=7n4
Since n must be a whole number (you can't have a fraction of a point), n must be a multiple of 4. The smallest value for n that satisfies this is 4.
Substituting n=4 back into the equation for r:
r=7×44=7 cm
Thus, the radius of the circle is 7 cm and the number of points on the circumference is 4.
- Radius of the circle: 7 cm
- Number of points: 4
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
The circumferences of two circles are touching externally. The distance between their centres is 12 cm. The radius of one circle is 7 cm. Find the diameter (in cm.) of the other circle.
A12B10C8D5 - Question 2 - Select One
The circumferences of two circles are touching externally. The distance between their centres is 12 cm. The radius of one circle is 7 cm. Find the diameter (in cm.) of the other circle.
A12B10C8D5
The difference between the circumference and radius of a circle is 37 cm. Find the area of the circle
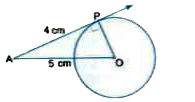
View SolutionThe length of a tangent from a point A at distance 5 cm from the centre of the circle is 4 cm. Find the radius of the circle.
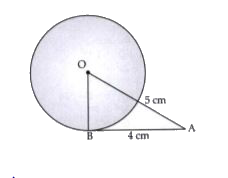
View SolutionFrom a point Q, the length of the tangent to a circle is 12 cm and distance of d from the centre is 13 cm. Find the radius of the circle.
View SolutionFrom a point Q, the length of the tangent to a circle is 24 cm and the distance of Q from the centre is 25 cm. Find the radius of the circle.
View SolutionThe difference between the circumference and radius of a circle is 37 cm. Find the area of the circle.
View SolutionA point moves quarter of a circle on the circumference of a circle of radius R. Find the distance travelled and displacement in terms of R.
View Solution