A ray of light suffers refraction through an equilateral prism. The deviation produced by the prism does not depend on the:
angle of incidence
colour of light
material of prism
size of prism
The correct Answer is:D
To solve the question regarding the factors affecting the deviation produced by a ray of light passing through an equilateral prism, we can follow these steps:
1. Understanding Refraction in Prisms:
- Refraction occurs when light passes from one medium to another, causing it to change direction. In the case of a prism, the light bends at the two surfaces it encounters.
2. Identifying Factors Affecting Deviation:
- The deviation produced by a prism is influenced by several factors:
- Angle of Incidence: The angle at which the light ray strikes the prism.
- Refractive Index: The property of the material of the prism that affects how much the light bends.
- Angle of the Prism: The angle between the two faces of the prism.
- Wavelength of Light: Different colors (or wavelengths) of light will refract differently due to their varying speeds in the material.
3. Analyzing the Options:
- We need to determine which factor does NOT affect the deviation. The options typically include:
- A) Angle of Incidence
- B) Refractive Index
- C) Wavelength of Light
- D) Size of the Prism
4. Evaluating Each Option:
- Angle of Incidence: This affects the deviation, as a larger angle can lead to more bending.
- Refractive Index: This is crucial as it determines how much the light will bend when entering and exiting the prism.
- Wavelength of Light: Different wavelengths will refract at different angles, which means this also affects deviation.
- Size of the Prism: The size of the prism (its dimensions) does not affect the angle of deviation. The deviation is determined by the angles and the material properties, not the physical size.
5. Conclusion:
- Based on the analysis, the deviation produced by the prism does not depend on the size of the prism. Therefore, the correct answer is D) Size of the Prism.
Final Answer:
The deviation produced by the prism does not depend on the size of the prism.
---
- Refraction occurs when light passes from one medium to another, causing it to change direction. In the case of a prism, the light bends at the two surfaces it encounters.
- The deviation produced by a prism is influenced by several factors:
- Angle of Incidence: The angle at which the light ray strikes the prism.
- Refractive Index: The property of the material of the prism that affects how much the light bends.
- Angle of the Prism: The angle between the two faces of the prism.
- Wavelength of Light: Different colors (or wavelengths) of light will refract differently due to their varying speeds in the material.
- We need to determine which factor does NOT affect the deviation. The options typically include:
- A) Angle of Incidence
- B) Refractive Index
- C) Wavelength of Light
- D) Size of the Prism
- Angle of Incidence: This affects the deviation, as a larger angle can lead to more bending.
- Refractive Index: This is crucial as it determines how much the light will bend when entering and exiting the prism.
- Wavelength of Light: Different wavelengths will refract at different angles, which means this also affects deviation.
- Size of the Prism: The size of the prism (its dimensions) does not affect the angle of deviation. The deviation is determined by the angles and the material properties, not the physical size.
- Based on the analysis, the deviation produced by the prism does not depend on the size of the prism. Therefore, the correct answer is D) Size of the Prism.
The deviation produced by the prism does not depend on the size of the prism.
Topper's Solved these Questions
- BOOK - ICSECHAPTER - REFRACTION OF LIGHT AT PLANE SURFACESEXERCISE - EXERCISE-4(B) NUMERICALS2videos
REFRACTION OF LIGHT AT PLANE SURFACES
- BOOK - ICSECHAPTER - REFRACTION OF LIGHT AT PLANE SURFACESEXERCISE - EXERCISE-4(C)8videos
REFRACTION OF LIGHT AT PLANE SURFACES
- BOOK - ICSECHAPTER - REFRACTION OF LIGHT AT PLANE SURFACESEXERCISE - EXERCISE-4(B)19videos
REFRACTION OF LIGHT AT PLANE SURFACES
- BOOK - ICSECHAPTER - REFRACTION OF LIGHTEXERCISE - DIAGRAM BASED MCQ:5videos
REFRACTION OF LIGHT
- BOOK - ICSECHAPTER - REFRACTION THROUGH A LENS EXERCISE - EXERCISE-5(D) (MULTIPLE CHOICE TYPE)2videos
REFRACTION THROUGH A LENS
Similar Questions
Knowledge Check
- Question 1 - Select One
In refraction of light through a prism the light ray:
Asuffers refraction only at one face of the prismBemergest out from the prism in a direction parallel to the incident rayCbends at both the surface of the prism towards its baseDbends at both the surfaces of the prism opposite to its base. - Question 2 - Select One
A ray of light passes through an equilateral prism (refractive index 1.5) such that angle of incidence is equal to angle of emergence and the latter is equal to 3/4th of the angle of prism. Calculate the angle of deviation.
A60∘B30∘C45∘D120∘
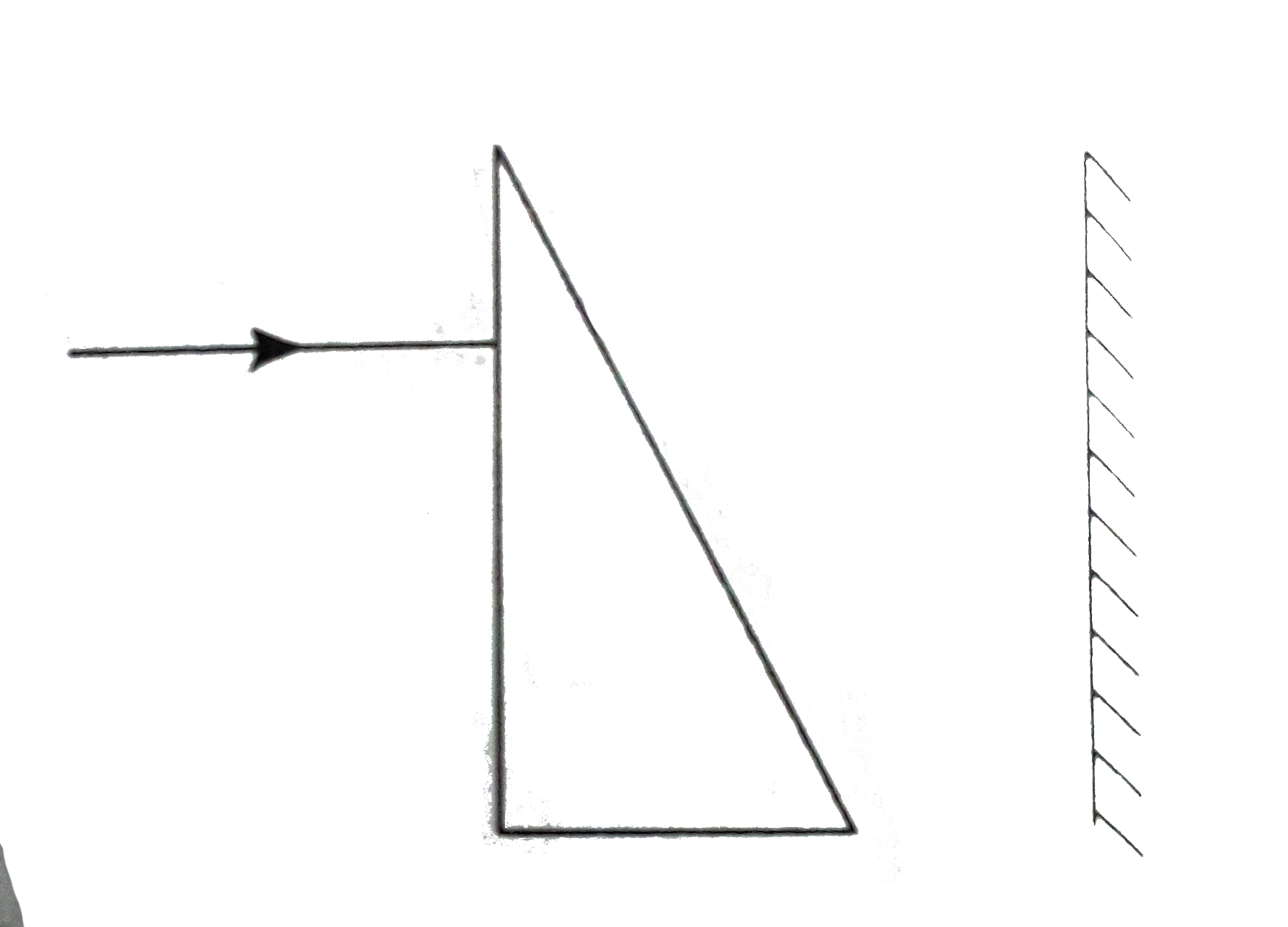
A right-angled prism of apex angle 4∘ and refractive index 1.5 is located in front of a vertical plane mirror as shown in figure. A horizontal ray of light is falling on the prism. Find the total deviation produced in the light ray as it emerges 2nd time from the prism.
 View Solution
View SolutionA ray of light passes through the equilateral prism such that angle of incidence is equal to the angle of emergence if the angle of incidence is 45∘. The angle of deviation will be
View SolutionA ray of light suffers a minimum deviation when incident on equilateral prism of refractive index √2 The angle of deviation is
View SolutionWhen a ray of light is incident normally on one refracting surface of an equilateral prism (Refractive index of the material of the prism =1.5)
View SolutionWhen a ray of light is incident normally on one refracting surface of an equilateral prism (Refractive index of the material of the prism =1.5
View SolutionA ray of light passes through an equilateral glass prism in such a manner that the angle of incidence is equal to the angle of emergence and each of these angles is equal to 3/4 of the angle of the prism. The angle of deviation is
View Solution