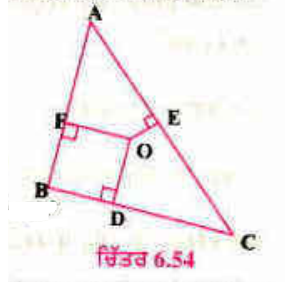
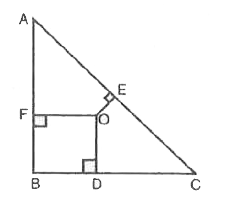
ਚਿੱਤਰ 6.54 ਵਿੱਚ, ΔABC ਦੇ ਵਿਚ ਸਥਿਤ ਕੋਈ ਬਿੰਦੂ O ਹੈ ਅਤੇ OD⊥BC,OE⊥AC ਅਤੇ OF⊥ABਹੈ।ਦਿਖਾਓ ਕਿ AF2+BD2+CE2=AE2+CD2+BF2
Step by step video & image solution for ਚਿੱਤਰ 6.54 ਵਿੱਚ, Delta ABC ਦੇ ਵਿਚ ਸਥਿਤ ਕੋਈ ਬਿੰਦੂ O ਹੈ ਅਤੇ OD bot BC,OE bot AC ਅਤੇ OF bot ABਹੈ।ਦਿਖਾਓ ਕਿ AF^2+BD^2+CE^2=AE^2+CD^2+BF^2 by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
ਚਿੱਤਰ 6.16 ਵਿੱਚ PSsQ=PTTR ਹੈ ਅਤੇ ∠PST=∠PRQ ਹੈ। ਸਿੱਧ ਕਰੋ ਕਿ ΔPQR ਇੱਕ ਸਮਦੋਭੁਜੀ ਤ੍ਰਿਭੁਜ ਹੈ।
View Solutionਜੇਕਰ tanA=43, ਤਾਂ ਕੋਣ A ਦੇ ਬਾਕੀ ਤਿਕੋਣਮਿਤਈ ਅਨੁਪਾਤ ਪਤਾ ਕਰੋ।
View Solutionਜਾਂਚ ਕਰੋ ਕਿ ਹੇਠਾਂ ਦਿੱਤੀਆਂ ਸਮੀਕਰਣਾਂ ਦੋ ਘਾਤੀ ਹਨ ਜਾਂ ਨਹੀ : (x+1)2=2(x−3)
View Solutionआकृति 6 . 54 में ΔABC के अभ्यन्तर में स्थित कोई बिंदु O है तथा OD⊥BC,OE⊥AC और OF⊥AB है दर्शाइए कि
(i) OA2+OB2+OC2−OD2−OE2−OF2=AF2+BD2+CE2
(ii) AF2+BD2+CE2=AE2+CD2+BF2

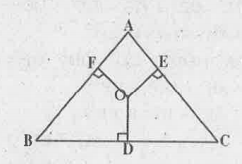
View SolutionO is a point in the interior of ΔABC,OD⊥BC,OE⊥ACandOF⊥AB, as shown in the figure,
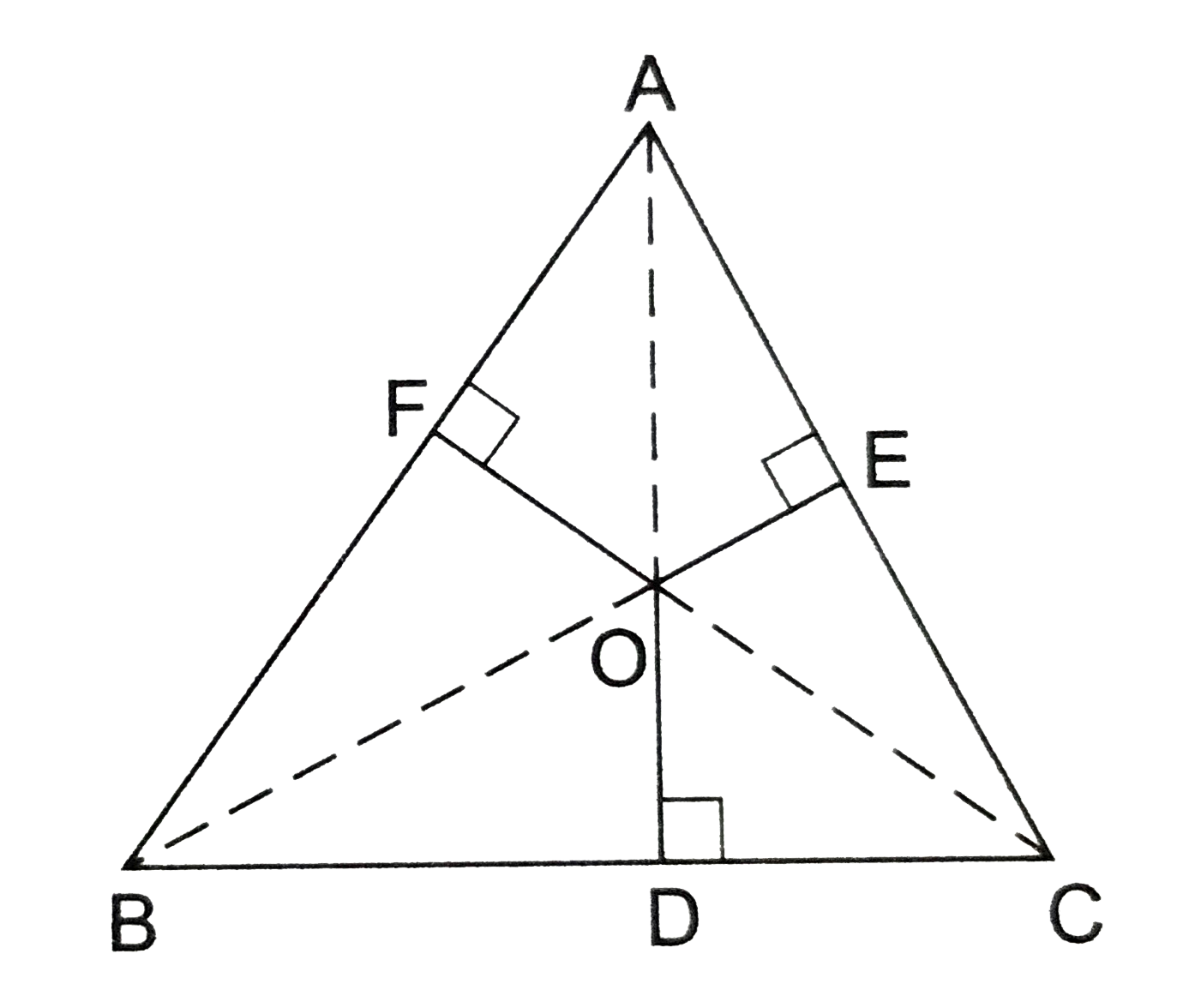
Prove that :
(i)OA2+OB2+OC2−OD2−OE2−OF2=AF2+BD2+CE2
(ii) AF2+BD2+CE2=AD2+BF2+CD2
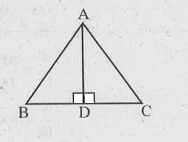
View Solutionसमद्विबाहु त्रिभुज ABC में AB=AC तथा BD⊥AC हो, तो सिद्ध कीजिए कि BD2−CD2=2CD.AD
View SolutionIn Δ ABC , AD ⊥BC and AD2=BD×CD. Prove that AB2+AC2= (BD+CD)2

View SolutionIn fig . O is a point in the interior of a triangle ABC , OD ⊥BC,OE⊥ACandOF⊥AB . Show that
(i) OA2+OB2+OC2−OD2−OE2−OF2=AF2+BD2+CE2
(ii) AF2+BD2+CE2+AE2+CD2+BF2 .
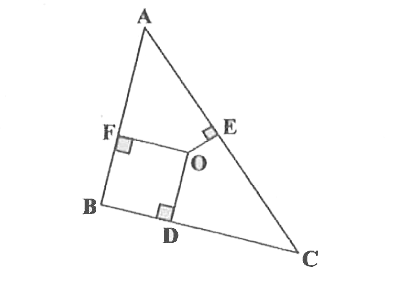
View Solutionआकृति में , ΔABC के अभ्यंतर में स्थित कोई बिंदु O है तथा OD⊥BC , OE⊥AC और OF⊥AB है । दर्शाइए कि
(i) OA2+OB2+OC2−OD2−OE2−OF2=AF2+BD2+CE2
(ii) AF2+BD2+CE2=AE2+CD2+BF2 .
View Solution△ABC ಯಲ್ಲಿ AD⊥BC ಮತ್ತು AD2=BDxCD ಆಗಿದೆ. ಹಾಗಾದರೆ AB2+AC2=(BD+CD)2 ಎಂದು ಸಾಧಿಸಿ.
View Solutionత్రిభుజము ABC అంతరంలో ఏదైనా బిందువు 'O' OD⊥BC,OE⊥AC మరియు OF⊥AB అయిన AE2+BF2+CD2=AF2+BD2+CE2 అని చూపండి.
View Solution‘O’ is any point in the interior of a triangle ABC. If OD⊥BC,OE⊥AC and OF ⊥ AB, show that
(i) OA2+OB2+OC2−OD2−OE2−OF2=AF2+BD2+CE2
(ii) AF2+BD2+CE2=AE2+CD2+BE2.
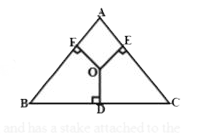
View Solution‘O’ is any point in the interior of a triangle ABC. If OD⊥BC,OE⊥AC and OF ⊥ AB, show that
(i) OA2+OB2+OC2−OD2−OE2−OF2=AF2+BD2+CE2
(ii) AF2+BD2+CE2=AE2+CD2+BE2.
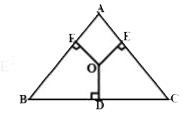
View SolutionIn the given figure, O is a point in the interior of a DeltaABC, OD⊥BC,OE⊥AC and OF⊥AB. Show that :
OA2+OB2+OC2−OD2−OE2−OF2=AF2+BD2+CE2.
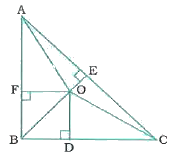
View SolutionIn the given figure, O is a point in the interior of a DeltaABC, OD⊥BC,OE⊥AC and OF⊥AB. Show that :
AF2+BD2+CE2=AE2+CD2+BF2.
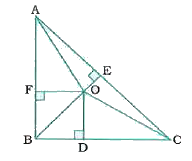
View Solution