A particle starts from rest in circular path of radius "R=2m" such that its angular velocity is ω=πt3rads .Find the magnitude of average velocity of particle when it has moved by angle 600 from its initial position
Step by step video solution for A particle starts from rest in circular path of radius "R=2m" such that its angular velocity is omega=(pi t)/(3)(rad)/s .Find the magnitude of average velocity of particle when it has moved by angle 60^(0) from its initial position by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
Starting from, rest, a particle rotates in a circle of radius R=√2m with an angular acceleration α=π4rads2.Calculate the magnitude of average velocity (in ms ) of the particle.over the time it rotates quarter circle.
View SolutionA particle starts from rest and its angular displacement (in rad) is given θ=t220+t5. Calculate the angular velocity at the end of t=4s.
View Solution
Knowledge Check
A particle starts from rest in circular path of radius R = 2 m such that its angular velocity is ω=πt3 rad/s. Find the magnitude of average velocity of particle when it has maoved by angle 60∘ from its initial position.
A√3m/sB2√3m/sC2√2m/sD√2m/sA particle is moving on a circular path of radius R with uniform angular speed ω. The magnitude of average velocityof particle during time t=0 to t=2π3ω:
A√32ωRπB32ωRπC3√32ωRπD23ωRπStarting from rest, a particle rotates in a circle of radius R 2 m with an angular acceleration = /4 rad/s2.The magnitude of average velocity of the particle over the time it rotates quarter circle is:
A1.5m/sB2m/sC1m/sD1.25m/s
A particle moves in a circular path of radius R with an angualr velocity ω=a−bt, where a and b are positive constants and t is time. The magnitude of the acceleration of the particle after time 2ab is
View SolutionA particle moves on the circumference of a circle of radius r. Starting from one point particle reaches to its diametric end in time t.The magnitude of average velocity of the particle is
View SolutionA particle describes uniform circular motion in a circle of radius 2 m, with the angular speed of 2 rad s−1. The magnitude of the change in its velocity in π2 s is
View SolutionA particle moving along a circule path of radius 'r' with uniform angular velocity ω. Its angular acceleration is
View SolutionA particle moves from A to B in a circular path of radius R covering an angle θ as shown in figure. Find the ratio of magnitude of distance and magnitude of displacement of the particle.
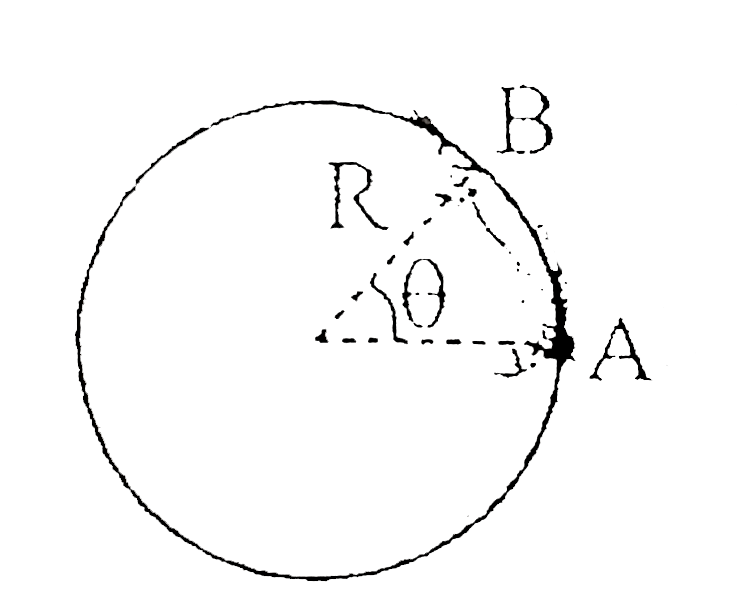
View Solution
