Which of the Maxwell distribution curves (obtained at different temperatures) shown in the following figure corresponds to the highest temperature of the gas ?
A. A
B. B
C. C
D. D
The correct Answer is:D
To determine which Maxwell distribution curve corresponds to the highest temperature of the gas, we can follow these steps:
1. Understand the Maxwell Distribution: The Maxwell distribution describes the distribution of speeds of particles in a gas. Each curve in the figure represents the speed distribution at a different temperature.
2. Recall the Relationship Between Temperature and Speed: The root mean square (RMS) speed of gas molecules is given by the formula:
vrms=√3RTM
where R is the gas constant, T is the temperature in Kelvin, and M is the molar mass of the gas. This indicates that the RMS speed is proportional to the square root of the temperature (vrms∝√T).
3. Analyze the Curves: In the given figure, each curve corresponds to a different temperature. The shape and position of the curves indicate the average speed of the gas molecules at those temperatures.
4. Identify the Highest Speed Curve: The curve that is furthest to the right on the x-axis (which represents speed) corresponds to the highest average speed of the gas molecules. Since speed is directly related to temperature, the curve with the highest average speed will correspond to the highest temperature.
5. Determine the Correct Curve: By comparing the curves, we can label them as follows:
- Curve A: UA
- Curve B: UB
- Curve C: UC
- Curve D: UD
From the analysis, we find that:
UD<UC<UB<UA
This indicates that Curve A has the highest speed and therefore corresponds to the highest temperature.
6. Conclusion: The curve that corresponds to the highest temperature of the gas is Curve A.
Final Answer:
The curve corresponding to the highest temperature of the gas is Curve A.
vrms=√3RTM
where R is the gas constant, T is the temperature in Kelvin, and M is the molar mass of the gas. This indicates that the RMS speed is proportional to the square root of the temperature (vrms∝√T).
- Curve A: UA
- Curve B: UB
- Curve C: UC
- Curve D: UD
UD<UC<UB<UA
This indicates that Curve A has the highest speed and therefore corresponds to the highest temperature.
The curve corresponding to the highest temperature of the gas is Curve A.
Topper's Solved these Questions
- STATES OF MATTER : GASES AND LIQUIDSBOOK - ICSECHAPTER - STATES OF MATTER : GASES AND LIQUIDSEXERCISE - TRUE OR FALSE TYPE QUESTION
15 Videos
- STATES OF MATTER : GASES AND LIQUIDSBOOK - ICSECHAPTER - STATES OF MATTER : GASES AND LIQUIDSEXERCISE - FILL IN THE BLANKS. TYPE QUESTIONS
10 Videos
- STATES OF MATTER : GASES AND LIQUIDSBOOK - ICSECHAPTER - STATES OF MATTER : GASES AND LIQUIDSEXERCISE - ESSAY (LONG ANSWER ) TYPE QUESTIONS
10 Videos
- SOME P-BLOCK ELEMENTS BOOK - ICSECHAPTER - SOME P-BLOCK ELEMENTS EXERCISE - NCERT TEXTBOOK EXERCISES (With Hints and Solutions)
63 Videos
- STRUCTURE OF ATOMBOOK - ICSECHAPTER - STRUCTURE OF ATOMEXERCISE - NCERT Textbook Exercises
67 Videos
Similar Questions
Which of the following decomposes at highest temperature?
View SolutionThe furnace which gives the highest temperature is
View SolutionWhich one of the following gases has the highest critical temperature ?
View SolutionWhich among the following will boil at highest temperature?
View SolutionWhich among the following will boil at highest temperature?
View SolutionState Stefan's law. Sketch graphs showing the distribution of energy in the spectrum of black body radiation at three temperatures, and indicate which curve corresponds to the highest temperature.
View SolutionWhich of the following aq. solutions will freeze at highest temperature?
View SolutionWhich of the following is a character of a gas at Boyle temperature?
View SolutionCompressibility factor (Z) is plotted against pressure at different temperature for same gas

Which of the following is the correct order of temperature shown in the above plot ?
View SolutionThe distribution of molecular kinetic energy at two temperature is as shown in the following graph:
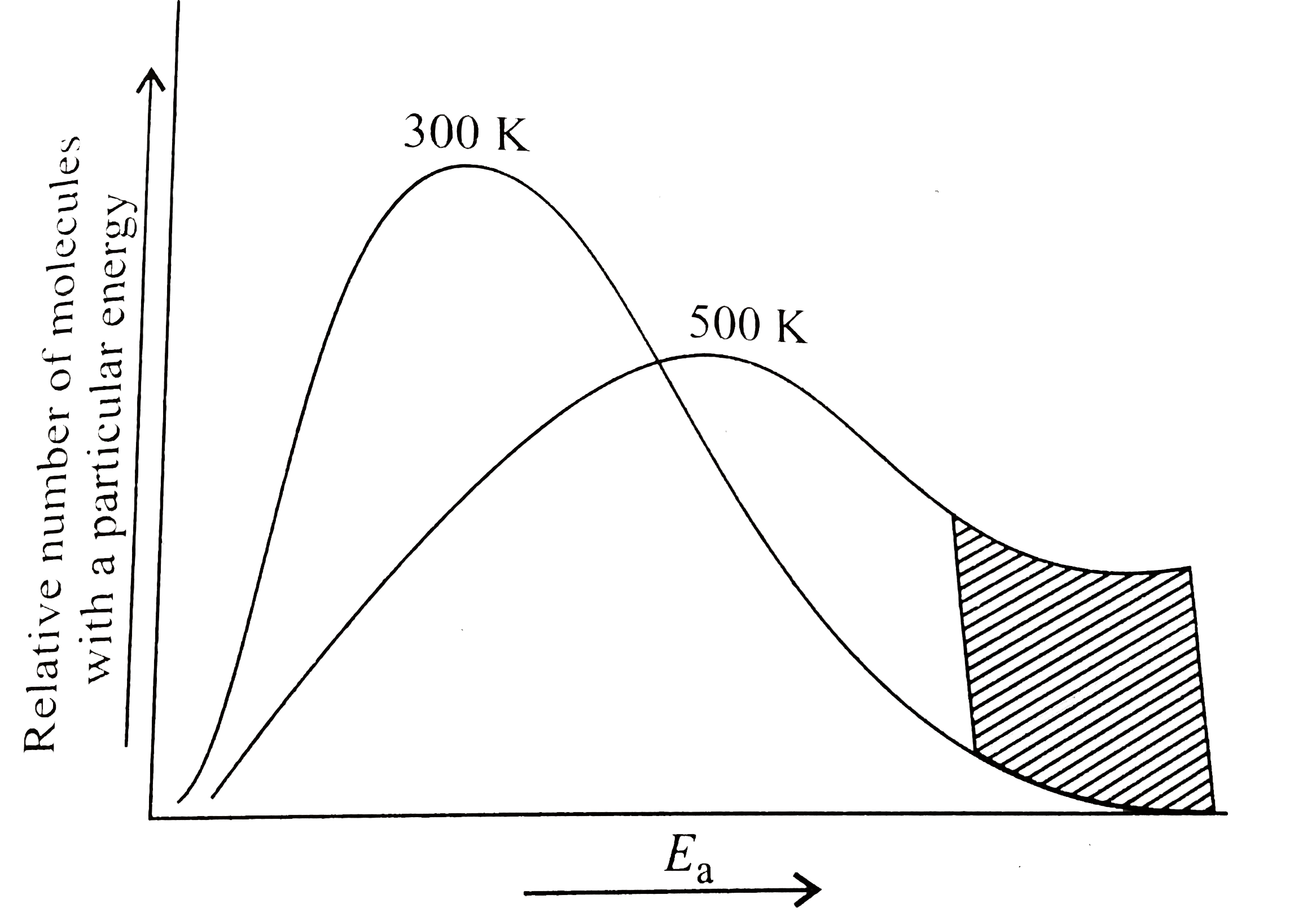
Which is the following concluison is/are correct?
View Solution
