Three insulated wires in a cable
Step-by-Step Solution:
1. Understanding the Question: The question refers to three insulated wires in a cable, which are commonly used in household wiring.
2. Identifying the Wires: In a typical cable, the three insulated wires are usually color-coded. The common colors are:
- Red (or sometimes Yellow): These wires are typically used as "hot" wires, which carry the current.
- Black: This is also a "hot" wire.
- White (or sometimes Green): This wire is used as the "neutral" wire.
3. Function of Each Wire:
- Hot Wires (Red and Black): These wires carry the electrical current from the power source to the electrical devices.
- Neutral Wire (White): This wire completes the electrical circuit by carrying the current back to the power source.
4. Application: The three-wire system is often used for various applications, including:
- Three-way switching: This allows control of a light from two different locations.
- Duplex receptacle wiring: This allows multiple devices to be connected to the same power source.
5. Conclusion: The three insulated wires in a cable serve essential functions in household wiring, with specific colors indicating their roles in the electrical system.
- Red (or sometimes Yellow): These wires are typically used as "hot" wires, which carry the current.
- Black: This is also a "hot" wire.
- White (or sometimes Green): This wire is used as the "neutral" wire.
- Hot Wires (Red and Black): These wires carry the electrical current from the power source to the electrical devices.
- Neutral Wire (White): This wire completes the electrical circuit by carrying the current back to the power source.
- Three-way switching: This allows control of a light from two different locations.
- Duplex receptacle wiring: This allows multiple devices to be connected to the same power source.
Topper's Solved these Questions
- ELECTRICITY BOOK - ICSECHAPTER - ELECTRICITY EXERCISE - Questions (Choose the correct option to fill in the blanks)
14 Videos
- ELECTRICITY BOOK - ICSECHAPTER - ELECTRICITY EXERCISE - Questions (Write T for true and F for False. Correct the false statements)
5 Videos
- ENERGYBOOK - ICSECHAPTER - ENERGYEXERCISE - THEME ASSIGNMENT
4 Videos
Similar Questions
Five very long, straight insulated wires are closely bound together to form a small cable. Currents carried by the wires are: I1=20A,I2=−6A,I3=12A,I4=−7A,I5=18A. (Negative currents are opposite in direction to the positve.) The magnetic field induction at a distance of 10cm from the cable is (current enters at A and leaves at B and C as shown)
View SolutionAssertion: The bending of an insulated wire increase the resistance of wire
Reason : The drift velocity of electron in this wire decreases
View Solution
Knowledge Check
Three elastic wires PQ,PR and PS support a body P of mass M, as shown in figure. The wires are of the some material and cross sectional area, the middle one being vertical. Find the loads by each wire.
 AMg/1+2cos2θBMg/1+2cos3θCMgcos2θ/1+2cos3θDMgcosθ/1+2cos3θ
AMg/1+2cos2θBMg/1+2cos3θCMgcos2θ/1+2cos3θDMgcosθ/1+2cos3θA long straight cable of length l is placed symmetrically along z-axis and has radius a(<<l). The cable consists of a thin wire and a co- axial conducting tube. An alternating current I(t)=I0 sin (2πvt). Flows down the central thin wire and returns along the co-axial conducting tube. the induced electric at a distance s from the wire inside the cable is
E(s,t)=μ0I0v cos (2πvt)In(sa)ˆk.
(i) Calculate the displacement current density inside the cable.
(ii) Integrate the displacement current density across the cross- section of the cable to find the total displacement current Id.
(iii) compare the conduction current I0 with the displacement current Id0.A2πλ2I0ln(as)sin(2πυt)ˆkB1λ2I0ln(as)sin(2πυt)ˆkCπλ2I0ln(as)sin(2πυt)ˆkDZero
How does the colour code of wires in a cable help in house wiring ?
View SolutionYou are given a U shaped soft iron piece, insulated copper wire and a battery. Draw a circuit diagram to make a horse shoe electromagnet.
View SolutionIn an electrical cable there is a single wire of radius 9mm of copper. Its resistance is 5Ω. The cable is replaced by 6 different insulated copper, wires the radius of each wire is 3mm. Now the total resistance of the cable will be
View SolutionThree elastic wires PQ,PR and PS support a body P of mass M, as shown in figure. The wires are of the some material and cross sectional area, the middle one being vertical. Find the loads by each wire.

View SolutionFig. below shows two bulbs with switches and fuse connected to the mains through a three pin socket by means of a three wires cable.
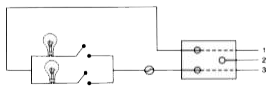
Name and state the colour of insulation of each wire 1, 2 and 3.
View SolutionAn elevator weighing 6000 kg is pulled upward by a cable with an acceleration of 5ms−2 . Taking g to be 10ms−2 , then the tension in the cable is
View Solution
