A rectangular water-tank measuring 80cm×60cm×60cm is filled from a pipe of cross-sectional area 1.5cm2, the water emerging at 3.2 m/s. How long does it take to fill the tank?
The correct Answer is:10 minutes
To solve the problem of how long it takes to fill a rectangular water tank with the given dimensions and flow rate, we will follow these steps:
Step 1: Calculate the Volume of the Tank
The volume V of a rectangular tank can be calculated using the formula:
V=length×breadth×height
Given dimensions are:
- Length = 80 cm
- Breadth = 60 cm
- Height = 60 cm
Substituting the values:
V=80cm×60cm×60cm
V=288000cm3
Step 2: Convert the Speed of Water to cm/s
The speed of water emerging from the pipe is given as 3.2 m/s. We need to convert this to cm/s:
3.2m/s=3.2×100cm/s=320cm/s
Step 3: Calculate the Flow Rate
The flow rate Q can be calculated using the formula:
Q=speed×cross-sectional area
Given the cross-sectional area of the pipe is 1.5cm2:
Q=320cm/s×1.5cm2
Q=480cm3/s
Step 4: Calculate the Time to Fill the Tank
The time t to fill the tank can be calculated using the formula:
t=Volume of the tankFlow rate
Substituting the values we found:
t=288000cm3480cm3/s
t=600s
Final Answer
The time required to fill the tank is 600 seconds.
---
The volume V of a rectangular tank can be calculated using the formula:
V=length×breadth×height
Given dimensions are:
- Length = 80 cm
- Breadth = 60 cm
- Height = 60 cm
V=80cm×60cm×60cm
V=288000cm3
The speed of water emerging from the pipe is given as 3.2 m/s. We need to convert this to cm/s:
3.2m/s=3.2×100cm/s=320cm/s
The flow rate Q can be calculated using the formula:
Q=speed×cross-sectional area
Given the cross-sectional area of the pipe is 1.5cm2:
Q=320cm/s×1.5cm2
Q=480cm3/s
The time t to fill the tank can be calculated using the formula:
t=Volume of the tankFlow rate
Substituting the values we found:
t=288000cm3480cm3/s
t=600s
The time required to fill the tank is 600 seconds.
Topper's Solved these Questions
- BOOK - ICSECHAPTER - SOLIDSEXERCISE - Exercise 21(C )10videos
SOLIDS
- BOOK - ICSECHAPTER - SOLIDSEXERCISE - Exercise 21(A)19videos
SOLIDS
- BOOK - ICSECHAPTER - SIMULTANEOUS LINEAR EQUATIONS IN TWO VARIABLESEXERCISE - Topic 2 (4 Marks questions)8videos
SIMULTANEOUS LINEAR EQUATIONS IN TWO VARIABLES
- BOOK - ICSECHAPTER - SOLUTION OF RIGHT TRIANGLESEXERCISE - EXERCISE 2435videos
SOLUTION OF RIGHT TRIANGLES
Similar Questions
Water is discharged from a pipe of cross-section area 3.2cm2 at the speed of 5m//s. Calculate the volume of water discharged:
in cm3 per secView SolutionWater is discharged from a pipe of cross-section area 3.2cm2 at the speed of 5m//s. Calculate the volume of water discharged:
in litres per minute.View SolutionA lawn sprinkler has 20 holes, each of cross-sectional area 2.0x10−2cm2, and is connected to a hose pipe of cross sectional area 2.4cm2. If the speed of water in the hose pipe is 1.5ms−1, the speed of water as it emerges from the holes is
View SolutionA rectangular tank is 80m long and 25m broad. Water flows into it through a pipe whose cross-section is 25cm2, at the rate of 16km per hour. How much the level of the water rises in the tank in 45 minutes.
View SolutionA rectangular tank 30cm×20cm×12cm contains water to a depth of 6cm. A metal cube of side 10cm is placed in the tank with its one face resting on the bottom of the tank. Find the volume of water, in litres, that must be poured in the tank so that the metal cube is just submerged in the water.
View SolutionA hose-pipe of cross-section area 2cm2 delivers 1500 litres of water in 5 minutes. What is the speed of water in m/s through the pipe?
View SolutionA rectangular tank having base 15cm×20cm is filled with water (density ρ=1000kg/m3) up to 20cm and force constant K=280N/m is fixed to the bottom of the tank so that the spring remains vertical.
This system is in an elevator moving downwards with acceleration a=2m/s2. A cubical block of side l=10cm and mass m=2kg is gently placed over the spring and released gradually, as shown in the figure.
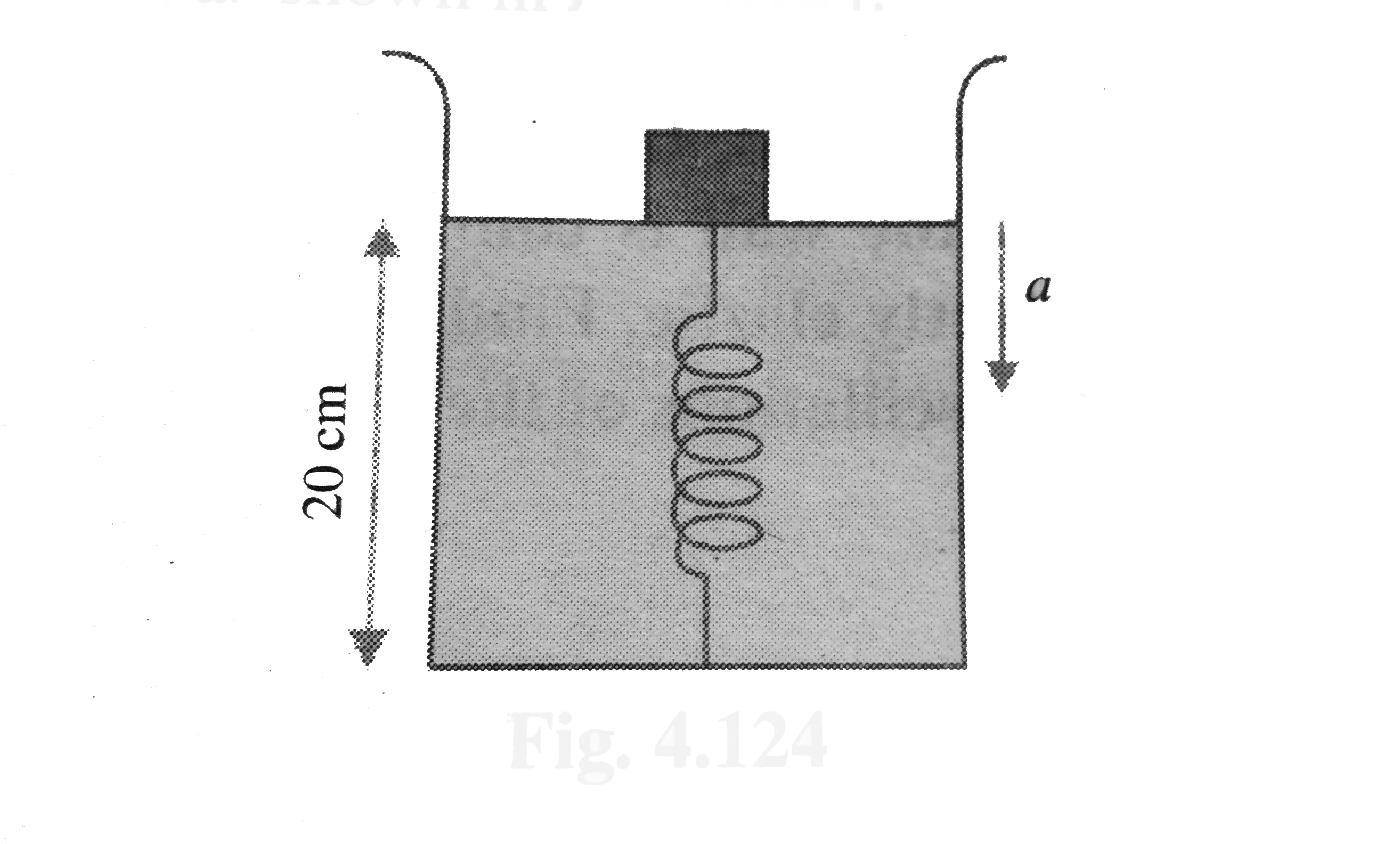
a. Calculate compression of the spring in equilibrium position.
b. If the block is slightly pushed down from equilibrium position and released, calculate frequency of its vertical oscillations.View SolutionA gardener holds a pipe of inside cross-sectional area 3.60cm2 at a height of 1.50m from ground. The opening at the nozzle of pipe has cross-sectional area 0.250cm2. Water velocity in the segment of the hose that lies on the ground is 50.0cm/s. a. What is the velocity of water leaving the nozzle? b. What is the thrust force experienced by the gardener due to flow of water through nozzle. c. What is the water pressure in the hose on the ground?
View SolutionA rectangular water tank of base 11m×6m contains water upto a height of 5 m. If the water in the tank is transferred to a cylindrical tank of radius 3.5 m, find the height of the water level in the tank.
View SolutionA water hose pipe of cross-sectional area 5cm2 is used to fill a tank of 120L. It has been observed that it takes 2 min to fill the tank. Now, a nozzel with an opening of cross-sectional area 1cm2 is attached to the hose. The nozzel is held so that water is projected horizontally from a point 1m above the ground. The horizontal distance over which the water can be projected is (Take g=10m/s2).
View Solution

