The coefficient of friction between the wedge and the table is μ, the friction between the rod and the wedge being negligible.
The correct Answer is:Q=m1m2gcos(α−φ)m1cos2αcosφ+m2sinαsin(α−φ);φ=arctan μ.
The equation of motion of the rod will remain unaltered, in the equation of motion of the wedge on should take into account the presence of the force of kinetic friction T=μ(Qcosα+m1g),
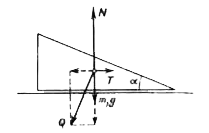
directed opposite to the acceleration (Fig.). Thus
−m2g+Qcosα=m2ay,−Qsinα+μ(Qcosα+m1g)=m1ax
After some transformations, noting that ay=axtanα, we obtain
Q=m1m2g(cosα+μsinα)m1cos2α+m2sin2α−μm2sinαcosα
ax=−m2g+μgcotα(m2+m1)m1cotα+m2tanα+μm2<0
ay=−m2gtanα+μg(m2+m1)m1cotα+m2tanα−μm2<0
The solution makes sense when
m1cotα+m2tanα>μm2,m2tanα>μ(m2+m1)
It follows from the second inequality that
tanα>μ(m2+m1)m2
If , on the other hand, tanα≤μ(m2+m1)m2 then ax=0,ay=0 and Q=m2gcosα.
Similar Questions
Knowledge Check
Wedge is fixed on horizontal surface. Block A is pulled upward by applying a force F as shown in figure and there is no friction between the wedge and the block A while coefficient of friction between A and B is μ. If there is no relative motion between the block A and B then frictional force developed between A and B is
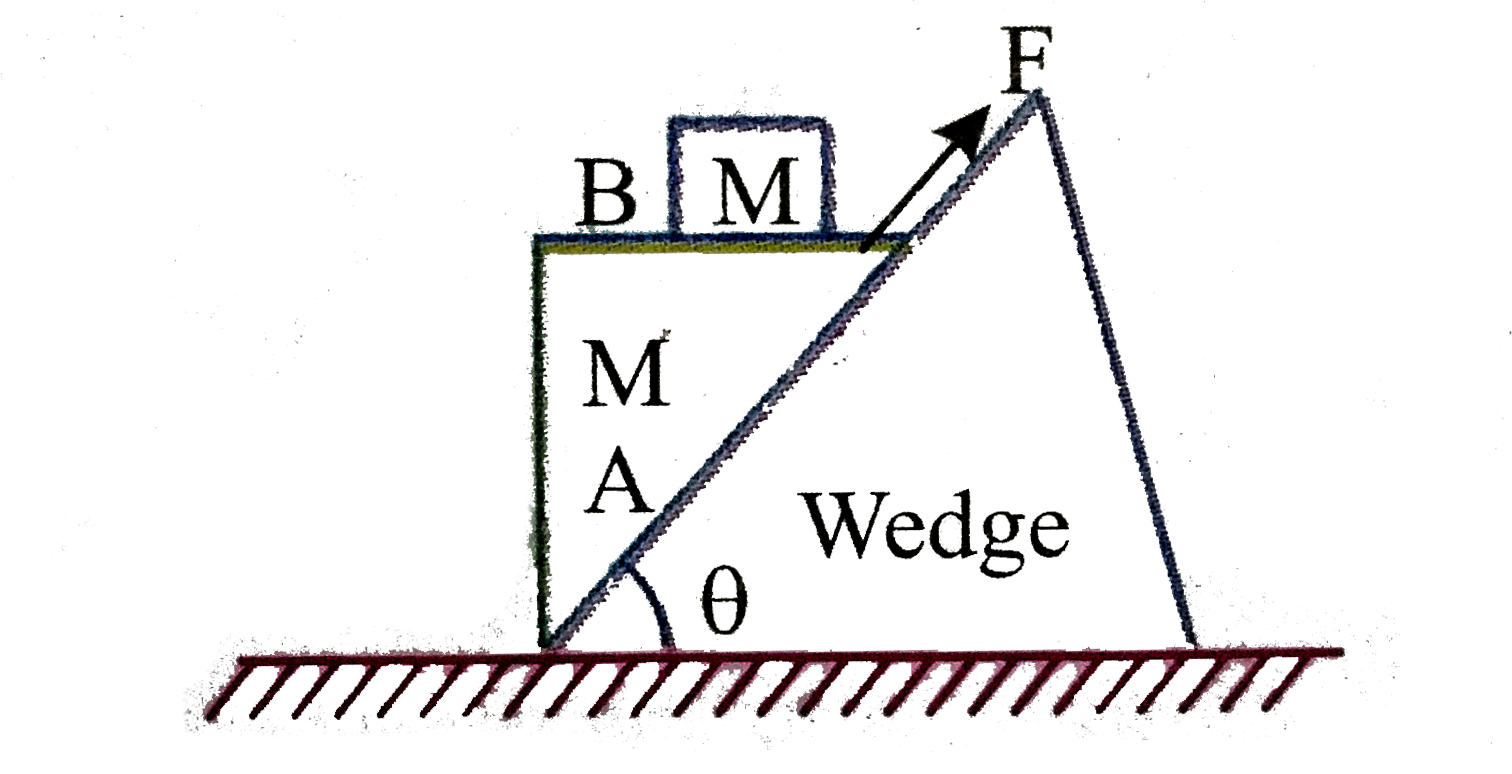 A[F+(m−M)gsinθ(m+M)]mcosθBμmgC[F−(m+M)gsinθ(m+M)]mcosθDμmg/2
A[F+(m−M)gsinθ(m+M)]mcosθBμmgC[F−(m+M)gsinθ(m+M)]mcosθDμmg/2A cube of mass m is placed on top of a wedge of mass 2m, as shown in figure. There is no friction between the cube and the wedge. The minimum co - efficient of friction between the wedge and the horizontal surface, so that the wedge does not move is
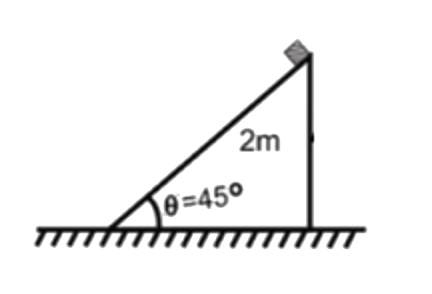 A0.1B0.2C0.3D1√2
A0.1B0.2C0.3D1√2
The coefficient of friction between the block and the wedge is μ. Friction between the wedge and the table is to be neglected.
View SolutionThe coefficient of friction between the block and the inclined plane is μ.
View SolutionA block A of massm which is placed a rough inclined face of a wedge of same mass being pulled through light string and with force F as shown in figure The coefficient of friction between inclined face and block A is μ while there is no friction between the ground and wedge it the whole system moves with same acceleration then find the value of F

View SolutionA block A of mass m which is placed a rough inclined face of a wedge of same mass being pulled through light string and with force F as shown in figure The coefficient of friction between inclined face and block A is μ while there is no friction between the ground and wedge it the whole system moves with same acceleration then find the value of F

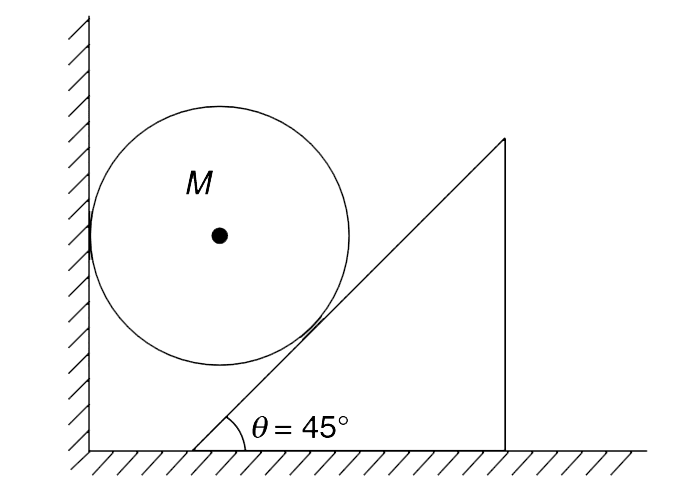
View SolutionA ball of mass M is in equilibrium between a vertical wall and the inclined surface of a wedge. The inclination of the wedge is θ=45∘ and its mass is very small compared to that of the ball. The coefficient of friction between the wedge and the floor is μ and there is no friction elsewhere. Find minimum value of μ for which this equilibrium is possible
View SolutionA cube of mass m is placed on top of a wedge of mass 2m, as shown in figure. There is no friction between the cube and the wedge. The minimum co - efficient of friction between the wedge and the horizontal surface, so that the wedge does not move is

View Solution
