Question

In Young's arrangement the distance between the slits is 1.5 mm and the distance of the screen from the slits is 2 m. The slits are illuminated by a source provided with a red filter $(\lambda =687nm)$. Find the distance between the interference fringes.
How will the distance between the fringes change, if the red filter is replaced by a greeen one $(\lambda =527nm)$ ?

Answer

0.9 mm; 0.7 mm

Explanation

Obviously, the principal (the zero-order) maximum will be observed in the centre of the interference pattern (Fig. 40.1). Let us find the coordinate of the m-th maximum, which we shall denote

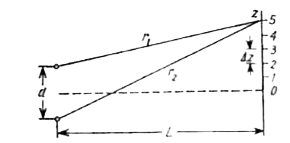
by `z_(m)`. This maximum will be observed when the propagation difference is `r_(2) - r_(1) 2m lambda//2`.
But `r_(1)^(2)=L^(2)+(z_(m)-(d)/(2))^(2)r_(2)^(2)=L^(2)+(z_(m)+(d)/(2))^(2)` Subtracting, we obtain `(r_(2)-r_(1))(r_(2)-r_(1))=2z_(m)d,`, Since `d lt L` and since in practice only the interference maxima of low order can be observed (i.e. `z_(m) lt L)`, we may put `r_(2) + r_(1) = 2L`. Hence
`2L(r_(2)-r_(1) = 2z_(m)d`, or `m lambda = z_(m)d`
Hence we obtain the coordinates of the maximum: `z_(m) = m lambda L//d.`
The separation between successive maxima (or minima) is
`Deltaz=z_(m+1)-z_(m)=lambdaL//d`