In parallelogram ABCD, E is the mid-point of side AB and CE bisect angle BCD. Prove that :
(i) AE=AD
(ii) DE bisect ∠ADC and
(iii) Angle DEC is a right angle.
To solve the problem step by step, we will prove the three statements regarding the parallelogram ABCD, where E is the midpoint of side AB and CE bisects angle BCD.
Step 1: Prove AE = AD
1. Identify the angles: Since ABCD is a parallelogram, we know that AB is parallel to DC. Therefore, angle DCE = angle CEB (alternate interior angles).
2. Use the angle bisector property: Since CE bisects angle BCD, we have angle DCE = angle ECB.
3. Set up the equations: From the above, we can conclude:
- angle CEB = angle ECB (from steps 1 and 2).
4. Apply the Isosceles Triangle Theorem: In triangle CEB, since angles CEB and ECB are equal, the sides opposite these angles must also be equal. Thus, EB = BC.
5. Use the midpoint property: Since E is the midpoint of AB, we have AE = EB.
6. Conclude with the properties of parallelograms: Since BC = AD (opposite sides of a parallelogram are equal), we can now conclude:
- AE = EB = BC = AD.
Thus, we have proved that AE = AD.
Step 2: Prove DE bisects angle ADC
1. Identify the angles: Since AB is parallel to DC, angle AEB = angle CDE (alternate interior angles).
2. Use the property of isosceles triangles: From the previous step, we know AE = AD. Therefore, in triangle ADE, angle AED = angle ADE (angles opposite equal sides).
3. Set up the equations: From the above, we have:
- angle AEB = angle CDE and angle AED = angle ADE.
4. Conclude: Since angle AED = angle ADE and angle AEB = angle CDE, we can conclude that DE bisects angle ADC.
Step 3: Prove angle DEC is a right angle
1. Use the properties of angles in a parallelogram: Since AD is parallel to BC, we know that angle ADC + angle DCB = 180° (consecutive interior angles).
2. Apply the angle bisector property: Since CE bisects angle BCD, we can express angle BCD as:
- angle BCD = angle DCB + angle DCE.
3. Set up the equation: From the previous step, we have:
- angle ADC + (angle DCB + angle DCE) = 180°.
4. Divide by 2: Since CE and DE are angle bisectors, we can express:
- (angle ADC/2) + (angle DCB/2) = 90°.
5. Identify the angles: The angles (angle ADC/2) and (angle DCB/2) correspond to angles EDC and ECD respectively.
6. Conclude: Since angle EDC + angle ECD = 90°, we can conclude that angle DEC must be 90°.
Thus, we have proved that angle DEC is a right angle.
Summary of Proofs
1. AE = AD.
2. DE bisects angle ADC.
3. Angle DEC is a right angle.
2. Use the angle bisector property: Since CE bisects angle BCD, we have angle DCE = angle ECB.
- angle CEB = angle ECB (from steps 1 and 2).
- AE = EB = BC = AD.
- angle AEB = angle CDE and angle AED = angle ADE.
- angle BCD = angle DCB + angle DCE.
- angle ADC + (angle DCB + angle DCE) = 180°.
- (angle ADC/2) + (angle DCB/2) = 90°.
1. AE = AD.
2. DE bisects angle ADC.
3. Angle DEC is a right angle.
Topper's Solved these Questions
Similar Questions
ABCD is a parallelogram E is mid-point of AB and DE bisects angle D. Prove that
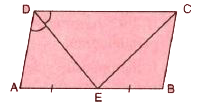
BC= BE
View SolutionABCD is a parallelogram E is mid-point of AB and DE bisects angle D. Prove that
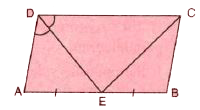
∠DEC=90∘
View SolutionABCD is a parallelogram E is mid-point of AB and DE bisects angle D. Prove that
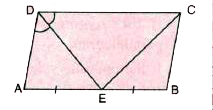
CE bisects angle C
View SolutionIn parallelogram ABCD, E is the mid-point of AD and F is the mid-point of BC. Prove that BFDE is a parallelogram.
View SolutionIn parallelogram ABCD, X and Y are mid-points of opposite sides AB and DC respectively. Prove that:
(i) AX=YC.
(ii) AX is parallel to YC
(iii) AXCY is a parallelogram.
View SolutionIn the following diagram, AD = AB and AE bisects angle A. Prove that :
(i) BE = DE (ii) ∠ABD>∠C
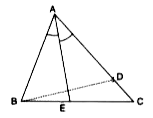
View SolutionIn a parallelogram ABCD, the bisector of ∠A bisects the line B at point X. Prove that AD = 2AB.
View SolutionIn ΔABC, AB = AC and the bisectors of angles B and C intersect at point O. Prove that :
(i) BO = CO
(ii) AO bisects angle BAC.
View SolutionIn ΔABC,D,E and F are mid-point of sides AB ,BC and AC respectively , Prove that AE and DF bisect each other.
View SolutionIn parallelogram ABCD, the bisector of angle A meets DC at P and AB= 2AD. Prove that:
Angle APB= 90∘
View Solution
