A pond appears to be 2.7 m deep. If the refractive index of water is 43, find the actual depth of the pond.
To find the actual depth of the pond, we can use the relationship between the refractive index, real depth, and apparent depth. Here’s a step-by-step solution:
Step 1: Understand the given information
- The apparent depth of the pond (the depth it appears to be) is given as 2.7 meters.
- The refractive index of water is given as 43.
Step 2: Use the formula for refractive index
The formula relating the refractive index (n), real depth (D), and apparent depth (d) is:
n=Dd
Where:
- n is the refractive index,
- D is the real depth,
- d is the apparent depth.
Step 3: Rearrange the formula to find the real depth
To find the real depth D, we can rearrange the formula:
D=n×d
Step 4: Substitute the values into the formula
Now, substitute the known values into the equation:
D=(43)×2.7
Step 5: Calculate the real depth
Now perform the multiplication:
D=4×2.73=10.83=3.6 meters
Final Answer
The actual depth of the pond is 3.6 meters.
---
- The apparent depth of the pond (the depth it appears to be) is given as 2.7 meters.
- The refractive index of water is given as 43.
The formula relating the refractive index (n), real depth (D), and apparent depth (d) is:
n=Dd
Where:
- n is the refractive index,
- D is the real depth,
- d is the apparent depth.
To find the real depth D, we can rearrange the formula:
D=n×d
Now, substitute the known values into the equation:
D=(43)×2.7
Now perform the multiplication:
D=4×2.73=10.83=3.6 meters
The actual depth of the pond is 3.6 meters.
Topper's Solved these Questions
Similar Questions
A water pond appears to be 2⋅7m dep. If the refractive index of water is 4/3, find the actual depth of the pond.
View SolutionA coin placed at the bottom of a beaker appears to be raised by 4.0 cm. If the refractive index of water is 4/3, find the depth of the water in the beaker.
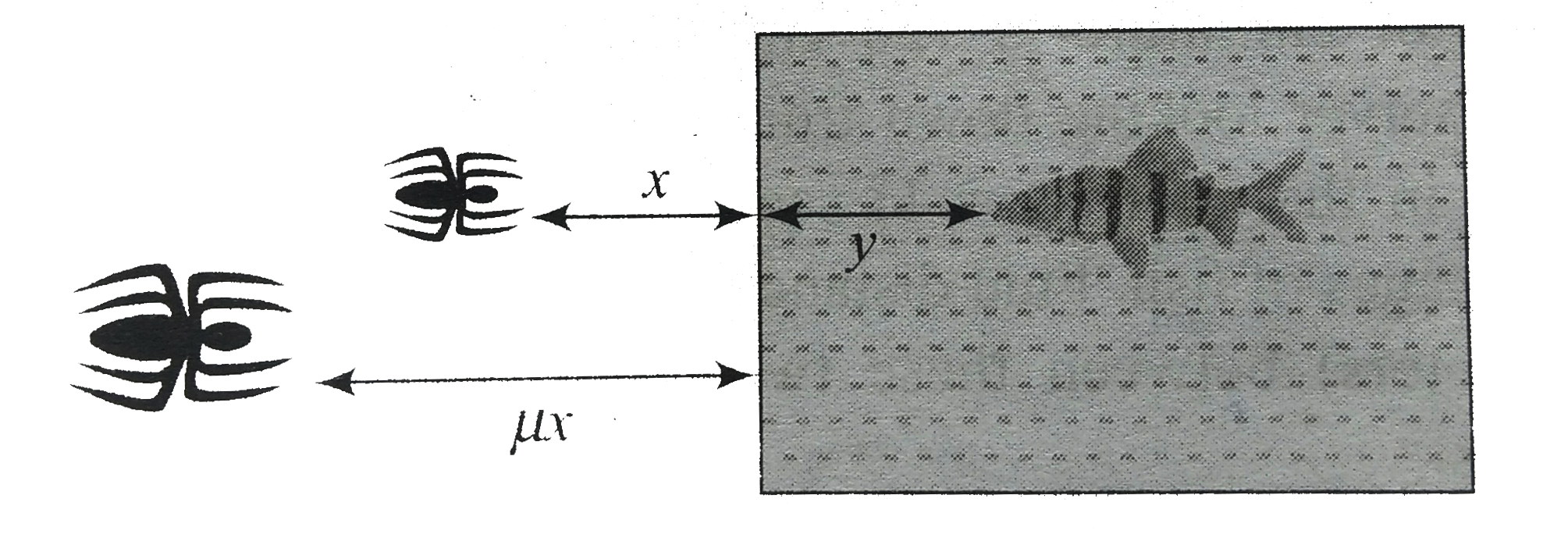
View SolutionA fish in an aquarium approaches the left wall at a rate of 3ms−1 observes a fly approaching it at 8ms−1. If the refractive index of water is (4/3), find the actual velocity of the fly.
 View Solution
View SolutionA fish in an aquarium approaches the left wall at a rate of 3ms−1 observes a fly approaching it at 8ms−1. If the refractive index of water is (4/3), find the actual velocity of the fly.
 View Solution
View SolutionThe refractive index of water is 4/3'. Explain the meaning of this statement.
View SolutionThe refractive index of water is 4/3. The speed of light in water is
View SolutionA layer of oil 3 cm thick is floating on a layer of coloured water 5 cm thick. Refractive index of coloured water is 5/3 and the apparent depth of the two liquids appears to be 36/7cm. Find the refractive index of oil.
View SolutionIf the speed of light in air is 3×108 m/s, and the refractive index of water is 1.33, find the speed of light in water.
View SolutionA glass slab of thickness 8 cms contains the same number of waves as 10 cms long path of water when both are traversed by the same monochromatic light. If the refractive index of water is 4/3, the refractive index of glass is
View SolutionAssertion A diverging lens (in air) cannot be made more diverging whatever be the medium we choose completely immerse the lens.
Reason The minimum refractive index of any medium is 1.View Solution

