A body of mass 10 kg is kept at a height of 5 m. It is allowed to fall and reach the ground.
What is the total mechanical energy possessed by the body at the height of 2 m assuming it is a frictionless medium ?
To solve the problem, we need to determine the total mechanical energy of the body at a height of 2 meters. The total mechanical energy is the sum of potential energy (PE) and kinetic energy (KE) of the body.
1. Identify the Given Values:
- Mass of the body (m) = 10 kg
- Height (h) = 5 m (initial height)
- Height at which we need to find the energy (h') = 2 m
- Acceleration due to gravity (g) = 10 m/s² (assuming standard value)
2. Calculate the Potential Energy at the Initial Height (5 m):
PE=m⋅g⋅h
PE=10kg⋅10m/s2⋅5m=500Joules
3. Understand the Conservation of Mechanical Energy:
- In a frictionless medium, the total mechanical energy (TME) is conserved. This means that the total mechanical energy at the initial height (5 m) will be equal to the total mechanical energy at any other height (including 2 m).
4. Determine the Total Mechanical Energy at Height 2 m:
- Since the total mechanical energy is conserved, the total mechanical energy at height 2 m will also be equal to the total mechanical energy at height 5 m.
TME=PE+KE
- At height 2 m, the total mechanical energy remains the same as at height 5 m, which is 500 Joules.
5. Conclusion:
- Therefore, the total mechanical energy possessed by the body at a height of 2 m is 500 Joules.
Final Answer:
The total mechanical energy possessed by the body at a height of 2 m is 500 Joules.
- Mass of the body (m) = 10 kg
- Height (h) = 5 m (initial height)
- Height at which we need to find the energy (h') = 2 m
- Acceleration due to gravity (g) = 10 m/s² (assuming standard value)
PE=m⋅g⋅h
PE=10kg⋅10m/s2⋅5m=500Joules
- In a frictionless medium, the total mechanical energy (TME) is conserved. This means that the total mechanical energy at the initial height (5 m) will be equal to the total mechanical energy at any other height (including 2 m).
- Since the total mechanical energy is conserved, the total mechanical energy at height 2 m will also be equal to the total mechanical energy at height 5 m.
TME=PE+KE
- At height 2 m, the total mechanical energy remains the same as at height 5 m, which is 500 Joules.
- Therefore, the total mechanical energy possessed by the body at a height of 2 m is 500 Joules.
The total mechanical energy possessed by the body at a height of 2 m is 500 Joules.
Topper's Solved these Questions
Similar Questions
A body of mass 10 kg is kept at a height of 5 m. It is allowed to fall and reach the ground.
What is the kinetic energy possessed by the body just before hitting the ground? (Take g = 10m/s2).
View SolutionThe potential energy possessed by a body at a height above the ground is called elastic potential energy.
View SolutionA body of mass 10 kg moving at a height of 2 m, with uniform speed of 2 m/s. Its total energy is
View SolutionA body of mass 0.2 kg falls from a height of 10 m to a height of 6 m above the ground. Find the loss in potential energy taking place in the body.
[g=10ms−2]
View SolutionA body of mass 5 kg falls from a height of 10 m to 4 m. Calculate : (i) the loss in potential energy of the body, and (ii) the total energy possessed by the body at any instant ? (Take -10 m s−2
View SolutionA body of mass 5 kg is raised vertically to a height of 10 m by a force 170 N. The velocity of the body at this height will be
View SolutionAn object of mass 40 kg is raised to a height of 5 m above the ground. What is its potential energy ? If the object is allowed to fall, find its kinetic energy when it is half - way down.
View SolutionA body of mass m is kept at a small height h above the ground. If the radius of the earth is R and its mass is M, the potential energy of the body and earth system (with h=∞ being the reference position) is
View SolutionAn object of mass 'm' is allowed to fall freely from a point A as shown in the figure.
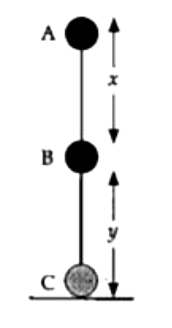
Calculate the total mechanical energy of object at:
Point A
View SolutionA body of mass m=2kg falls from a height h=10m from the ground level .
What is the magnitude of total change in momentum of the body before it strikes the ground?
View Solution