What is the moment of inertia of a ring about a tangent to the periphery of the ring?
12MR2
32MR2
MR2
MR2/9
To find the moment of inertia of a ring about a tangent to its periphery, we can use the parallel axis theorem. Here’s a step-by-step solution:
Step 1: Identify the moment of inertia of the ring about its center
The moment of inertia IC of a ring about an axis through its center and perpendicular to its plane is given by the formula:
IC=mr2
where m is the mass of the ring and r is the radius of the ring.
Step 2: Apply the Parallel Axis Theorem
The parallel axis theorem states that if you know the moment of inertia of a body about an axis through its center of mass, you can find the moment of inertia about any parallel axis by using the formula:
I=IC+md2
where:
- I is the moment of inertia about the new axis,
- IC is the moment of inertia about the center of mass,
- m is the mass of the object,
- d is the distance between the two axes.
Step 3: Determine the distance d
In this case, the distance d from the center of the ring to the tangent line at the periphery is equal to the radius r of the ring.
Step 4: Substitute the values into the parallel axis theorem
Now we can substitute IC and d into the parallel axis theorem:
I=IC+md2=mr2+mr2
This simplifies to:
I=2mr2
Final Answer
Thus, the moment of inertia of the ring about a tangent to the periphery of the ring is:
I=2mr2
---
The moment of inertia IC of a ring about an axis through its center and perpendicular to its plane is given by the formula:
IC=mr2
where m is the mass of the ring and r is the radius of the ring.
The parallel axis theorem states that if you know the moment of inertia of a body about an axis through its center of mass, you can find the moment of inertia about any parallel axis by using the formula:
I=IC+md2
where:
- I is the moment of inertia about the new axis,
- IC is the moment of inertia about the center of mass,
- m is the mass of the object,
- d is the distance between the two axes.
In this case, the distance d from the center of the ring to the tangent line at the periphery is equal to the radius r of the ring.
Now we can substitute IC and d into the parallel axis theorem:
I=IC+md2=mr2+mr2
This simplifies to:
I=2mr2
Thus, the moment of inertia of the ring about a tangent to the periphery of the ring is:
I=2mr2
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
Moment of inertia of a ring about a tangent to the circle of the ring is
A25MR2B23MR2C32MR2DNone of these - Question 2 - Select One
A ring and a disc have same mass and same radius. Ratio of moments of inertia of the ring about a tangent in its plane to that of the disc about its diameter is
A2:1B4:1C6:1D8:1 - Question 3 - Select One
The moment of inertia of semicircular ring about its centre is
AMR2BMR22CMR24DNone of these
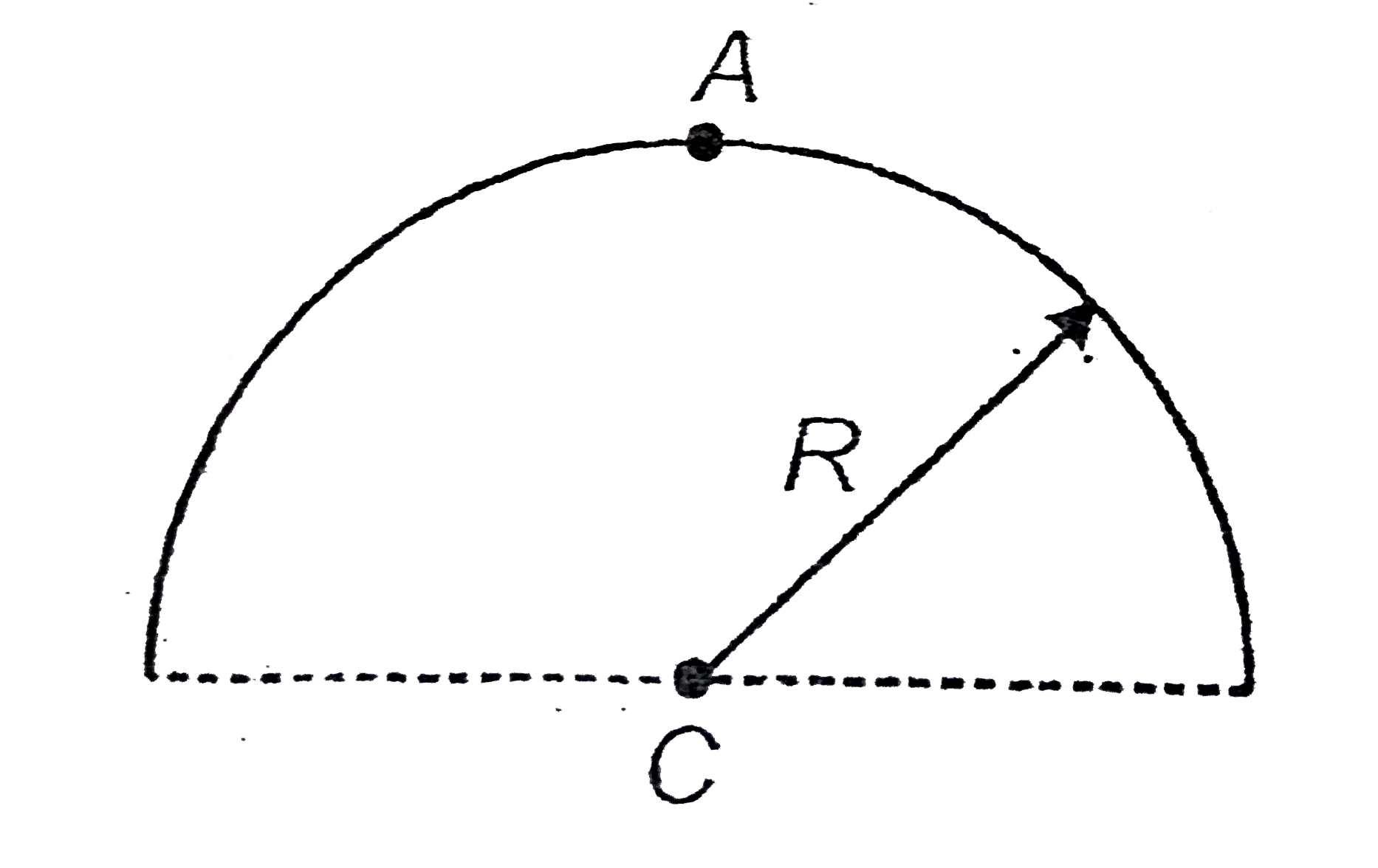
Moment of inertia of a half ring of mass m and radius R about an axis passing through point A perpendicular to the plane of the paper is IA. If IC is the moment of inertia of the ring about an axis perpendicular to the plane of paper and passing through point C, then
 View Solution
View SolutionThe moment of inertia of a circular ring about the tangent to the ring which is parallel to a diameter of the ring is 15gcm2. If the mass of the ring is 2g, what is its radius?
View SolutionTwo identical concentric rings each of mass m and radius R are placed perpendicularly. What is the moment of inertia of the system about the axis of one of the rings ?
View SolutionMoment of inertia of a disc about an axis which is tangent and parallel to its plane is I . Then the moment of inertia of disc about a tangent, but perpendicular to its plane will be
View SolutionM is mass and R is radius of a circular ring. The moment of inertia of same ring about an axis in the plane of ring at a perpendicular distance 2R3 from centre of ring is
View Solution

