What is the moment of inertia of a ring about a tangent to the circle of the ring ?
Video Solution
Text Solution
The tangent to the ring in the plane of the ring is parallel to one of the diameters of the ring. This distance between these two parallel axes is R, the radius of the ring. Using the parallel axes theorem.
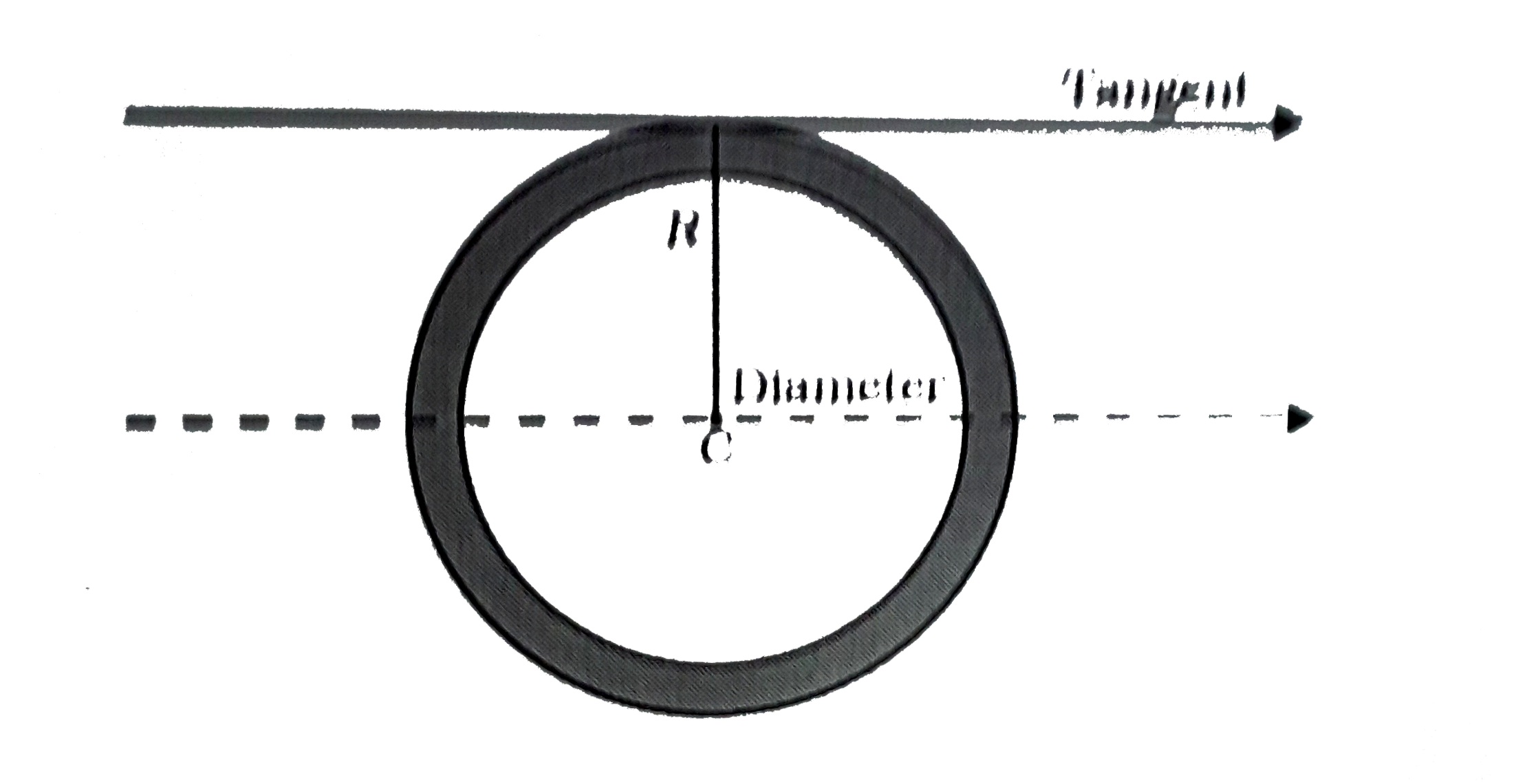
|
Topper's Solved these Questions
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBook:NCERTChapter:SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONExercise:EXERCISE
Explore 33 Videos
- PHYSICAL WORLD Book:NCERTChapter:PHYSICAL WORLD Exercise:EXERCISE
Explore 16 Videos
- THERMAL PROPERTIES OF MATTERBook:NCERTChapter:THERMAL PROPERTIES OF MATTERExercise:EXERCISE
Explore 22 Videos
Similar Questions
Knowledge Check
Moment of inertia of a ring about a tangent to the circle of the ring is
A25MR2B23MR2C32MR2DNone of theseTwo identical concentric rings each of mass m and radius R are placed perpendicularly. What is the moment of inertia of the system about the axis of one of the rings ?
A3MR2B32MR2C14MR2D2MR2A ring and a disc have same mass and same radius. Ratio of moments of inertia of the ring about a tangent in its plane to that of the disc about its diameter is
A2:1B4:1C6:1D8:1
The moment of inertia of a circular ring about the tangent to the ring which is parallel to a diameter of the ring is 15gcm2. If the mass of the ring is 2g, what is its radius?
View SolutionM is mass and R is radius of a circular ring. The moment of inertia of same ring about an axis in the plane of ring at a perpendicular distance 2R3 from centre of ring is
View SolutionMoment of inertia of a ring of mass M and radius R about a tangent to the circle of the ring is
View SolutionMoment of inertia of a ring of mass M and radius R about a tangent to the circle of the ring is
View SolutionThe moment of inertia of semicircular ring about its centre is
View Solution