A is a point on the x-axis and B is (-7, 9). Distance between the points A and B is 15 units. Find the coordinates of point A.
To find the coordinates of point A on the x-axis, given that point B is at (-7, 9) and the distance between A and B is 15 units, we can follow these steps:
Step 1: Define the coordinates of point A
Since point A lies on the x-axis, its coordinates can be represented as A(x,0).
Step 2: Use the distance formula
The distance d between two points (x1,y1) and (x2,y2) is given by the formula:
d=√(x2−x1)2+(y2−y1)2
In our case, the points are A(x,0) and B(−7,9), and the distance d is 15 units. Therefore, we can set up the equation:
15=√((−7)−x)2+(9−0)2
Step 3: Square both sides to eliminate the square root
Squaring both sides gives:
152=((−7)−x)2+(9−0)2
This simplifies to:
225=(−7−x)2+92
Step 4: Calculate 92
Calculating 92:
92=81
So, we can rewrite the equation as:
225=(−7−x)2+81
Step 5: Isolate the squared term
Subtract 81 from both sides:
225−81=(−7−x)2
This simplifies to:
144=(−7−x)2
Step 6: Take the square root of both sides
Taking the square root gives us two possible equations:
−7−x=12or−7−x=−12
Step 7: Solve for x in both cases
Case 1:
−7−x=12⟹−x=12+7⟹−x=19⟹x=−19
Case 2:
−7−x=−12⟹−x=−12+7⟹−x=−5⟹x=5
Step 8: Write the coordinates of point A
The coordinates of point A can be either:
1. A(−19,0)
2. A(5,0)
Final Answer
The coordinates of point A are (−19,0) and (5,0).
---
Since point A lies on the x-axis, its coordinates can be represented as A(x,0).
The distance d between two points (x1,y1) and (x2,y2) is given by the formula:
d=√(x2−x1)2+(y2−y1)2
In our case, the points are A(x,0) and B(−7,9), and the distance d is 15 units. Therefore, we can set up the equation:
15=√((−7)−x)2+(9−0)2
Squaring both sides gives:
152=((−7)−x)2+(9−0)2
This simplifies to:
225=(−7−x)2+92
Calculating 92:
92=81
So, we can rewrite the equation as:
225=(−7−x)2+81
Subtract 81 from both sides:
225−81=(−7−x)2
This simplifies to:
144=(−7−x)2
Taking the square root gives us two possible equations:
−7−x=12or−7−x=−12
Case 1:
−7−x=12⟹−x=12+7⟹−x=19⟹x=−19
−7−x=−12⟹−x=−12+7⟹−x=−5⟹x=5
The coordinates of point A can be either:
1. A(−19,0)
2. A(5,0)
The coordinates of point A are (−19,0) and (5,0).
Topper's Solved these Questions
- ICSE EXAMINATION PAPER 2020 BOOK - ICSECHAPTER - ICSE EXAMINATION PAPER 2020 EXERCISE - SECTION - B
21 Videos
- GRAPHICAL SOLUTION(SOLUTION OF SIMULTANEOUS LINEAR EQUATIONS, GRAPHICALLY)BOOK - ICSECHAPTER - GRAPHICAL SOLUTION(SOLUTION OF SIMULTANEOUS LINEAR EQUATIONS, GRAPHICALLY)EXERCISE - EXAMPLES
6 Videos
- INDICES (EXPONENTS)BOOK - ICSECHAPTER - INDICES (EXPONENTS)EXERCISE - 4 Marks Questions
9 Videos
Similar Questions
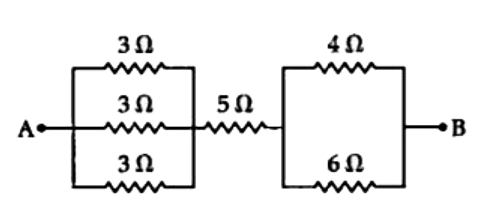
Find the resistance between points A and B.
View SolutionThe distance between the points (1,3) and (x,7) is 5, find x.
View SolutionFind distance between the points A (a,b) and B( - b,a)
View SolutionFind the distance between the points (0, 0) and (36, 15).
View SolutionFind the value of a , if the distance between the points A (-3,-14) and B (a,-5) is 9 units.
View SolutionFind the distance between the points (a,b)and(−b,a)
View SolutionA is a point on x- axis, and point B (5,-4) and AB = 5 units find the co-ordinates of A.
View SolutionA and B are two points on the x-axis and y-axis respectively. P(2, -3) is the mid point of AB. Find the
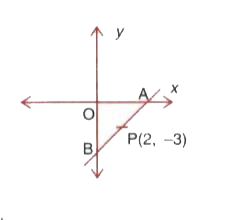
co-ordinates of A and B.
View SolutionFind the distance between points A(4,6) and B(4,−2) .
View SolutionFind the distances between the points
(2,-5) and (7,7)
View Solution
