From a disc of radius RandmassM, a circular hole of diameter R, whose rim passes through the centre is cut. What is the moment of inertia of remaining part of the disc about a perependicular axis, passing through the centre ?
13MR2/32
11MR2/32
9MR2/32
15MR2/32
The correct Answer is:a
To find the moment of inertia of the remaining part of the disc after cutting a circular hole, we can follow these steps:
Step 1: Calculate the Moment of Inertia of the Full Disc
The moment of inertia I of a full disc about an axis perpendicular to its plane and passing through its center is given by the formula:
Itotal=12MR2
where M is the mass of the disc and R is its radius.
Step 2: Determine the Mass of the Removed Portion
The diameter of the hole is R, which means the radius of the hole r is:
r=R2
The area of the full disc is:
Adisc=πR2
The area of the hole is:
Ahole=π(R2)2=πR24
The mass of the removed portion (the hole) can be calculated using the ratio of areas:
Mremoved=M⋅AholeAdisc=M⋅πR24πR2=M4
Step 3: Calculate the Moment of Inertia of the Removed Portion
Using the parallel axis theorem, the moment of inertia of the removed portion about the same axis can be calculated as:
Iremoved=ICM+Mremovedd2
where ICM is the moment of inertia of the hole about its own center of mass, and d is the distance from the center of the disc to the center of the hole.
The moment of inertia of the hole about its center is:
ICM=12Mremovedr2=12(M4)(R2)2=12⋅M4⋅R24=MR232
The distance d from the center of the disc to the center of the hole is R2. Therefore, we have:
Iremoved=MR232+(M4)(R2)2=MR232+M4⋅R24=MR232+MR216
Converting MR216 to a common denominator:
MR216=2MR232
Thus,
Iremoved=MR232+2MR232=3MR232
Step 4: Calculate the Moment of Inertia of the Remaining Part
Now, we can find the moment of inertia of the remaining part of the disc:
Iremaining=Itotal−Iremoved=12MR2−3MR232
Finding a common denominator (which is 32):
Iremaining=16MR232−3MR232=13MR232
Final Answer
The moment of inertia of the remaining part of the disc about a perpendicular axis passing through the center is:
13MR232
The moment of inertia I of a full disc about an axis perpendicular to its plane and passing through its center is given by the formula:
Itotal=12MR2
where M is the mass of the disc and R is its radius.
The diameter of the hole is R, which means the radius of the hole r is:
r=R2
The area of the full disc is:
Adisc=πR2
The area of the hole is:
Ahole=π(R2)2=πR24
The mass of the removed portion (the hole) can be calculated using the ratio of areas:
Mremoved=M⋅AholeAdisc=M⋅πR24πR2=M4
Using the parallel axis theorem, the moment of inertia of the removed portion about the same axis can be calculated as:
Iremoved=ICM+Mremovedd2
where ICM is the moment of inertia of the hole about its own center of mass, and d is the distance from the center of the disc to the center of the hole.
ICM=12Mremovedr2=12(M4)(R2)2=12⋅M4⋅R24=MR232
The distance d from the center of the disc to the center of the hole is R2. Therefore, we have:
Iremoved=MR232+(M4)(R2)2=MR232+M4⋅R24=MR232+MR216
Converting MR216 to a common denominator:
MR216=2MR232
Thus,
Iremoved=MR232+2MR232=3MR232
Now, we can find the moment of inertia of the remaining part of the disc:
Iremaining=Itotal−Iremoved=12MR2−3MR232
Finding a common denominator (which is 32):
Iremaining=16MR232−3MR232=13MR232
The moment of inertia of the remaining part of the disc about a perpendicular axis passing through the center is:
13MR232
Similar Questions
From a circular disc of radius R and mass 9 M , a small disc of radius R/3 is removed from the disc. The moment of inertia of the remaining disc about an axis perpendicular to the plane of the disc and passing through O is

View SolutionFrom a disc of radius R, a concentric circular portion of radius r is cut out so as to leave an annular disc of mass M. The moment of inertia of this annular disc about the axis perpendicular to its plane and passing through its centre of gravity is
View Solution
Knowledge Check
From a uniform circular disc of radius R and mass 9M, a small disc of radius R3 is removed as shown in the figure. The moment of inertia of the remaining disc about an axis perpendicular to the plane of the disc and passing through centre of disc is :
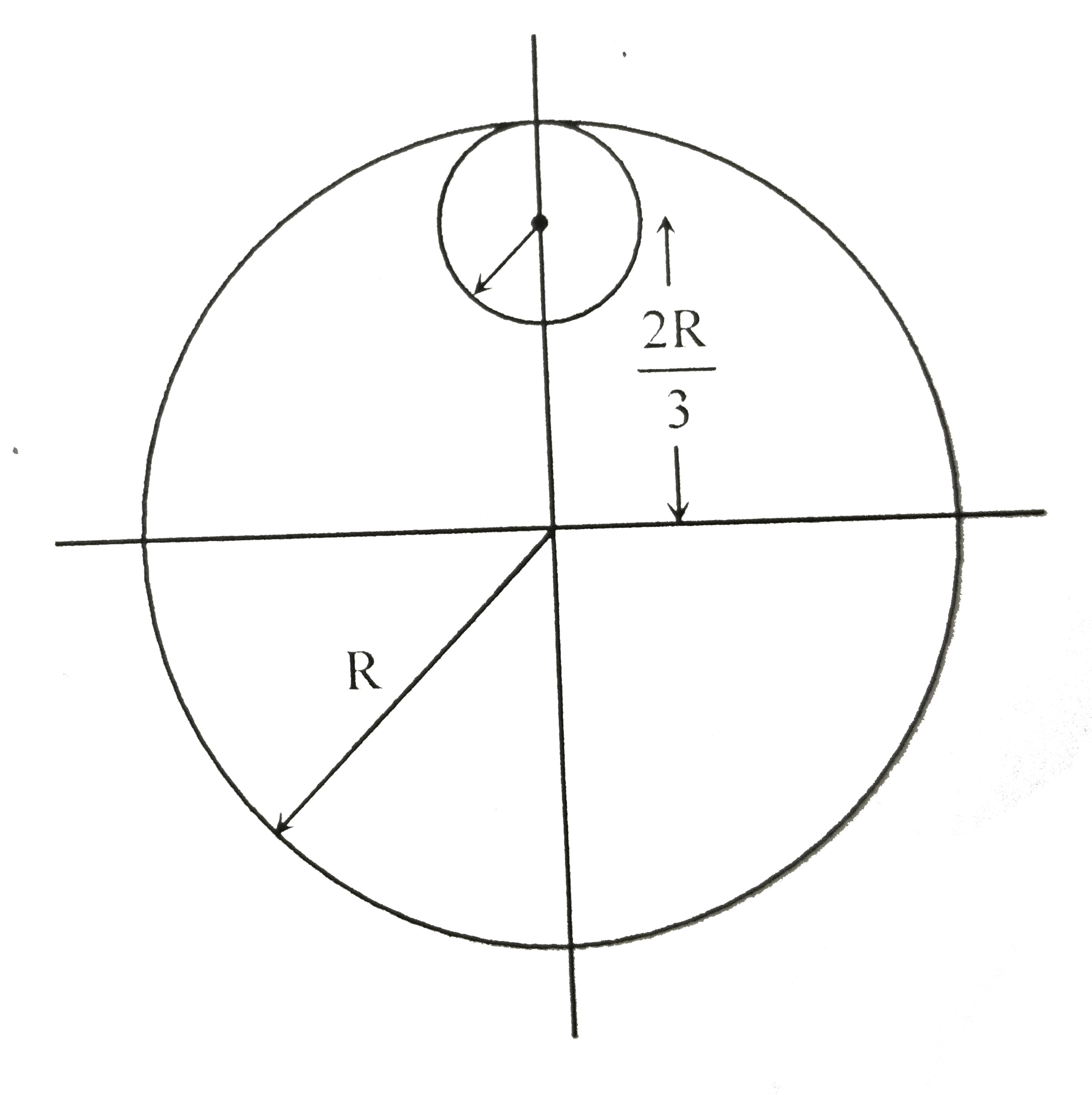 A4MR2B409MR2C40MR2D379MR2
A4MR2B409MR2C40MR2D379MR2
The moment of inertia of a uniform circular disc is maximum about an axis perpendicular to the disc and passing through -
View SolutionA uniform disc of mass m and radius R has an additional rim of mass m as well as four symmetrically placed masses, each of mass m/4 tied at positions R/2 from the centre as shown in Fig. What is the total moment of inertia of the disc about an axis perpendicular to the disc through its centre?

View SolutionA uniform disc (of mass M and radius a) has a hole (of radius b) drilled through it. The centre of the hole is at a distance c from the centre of the original disc. What is the moment of inertia of the disc about an axis through the centre of the disc and perpendicular to its plane ?
View SolutionThe moment of inertia of a copper disc, rotating about an axis passing through its centre and perpendicular to its plane
View Solution
A circular disc of radius b has a hole of radius a at its centre (see figure) if the mass per unit area of the disc varies as (σ0r) then the radius of gyration of the disc about its axis passing through the centre is
View SolutionMoment of inertia of a ring of mass M and radius R about an axis passing through the centre and perpendicular to the plane is
View SolutionA hole of radius R/2 is removed from a thin circular plate of radius R and mass M. The moment of inertia of the remaining plate about an axis through centre O and perpendicular to the plane of the plate is

View Solution
