A solid sphere of radius R is placed on a smooth horizontal surface. A horizontal force F is applied at height h from the lowest point. For the maximum acceleration of the centre of mass
h=R
h=2R
h=0
the acceleration will be same whatever h may be
The correct Answer is:d
To solve the problem of determining the maximum acceleration of the center of mass of a solid sphere when a horizontal force F is applied at a height h from the lowest point, we can follow these steps:
1. Understanding the System:
- We have a solid sphere of radius R resting on a smooth horizontal surface.
- A horizontal force F is applied at a height h from the lowest point of the sphere.
2. Identify the Forces:
- The only external horizontal force acting on the sphere is F.
- The sphere has a mass m.
3. Acceleration of the Center of Mass:
- The acceleration acm of the center of mass of the sphere can be calculated using Newton's second law:
acm=Fm
- This equation indicates that the acceleration of the center of mass depends solely on the net external force F and the mass m of the sphere.
4. Effect of Height h:
- The height h at which the force is applied affects the torque about the center of mass but does not affect the linear acceleration of the center of mass.
- The torque τ caused by the force F is given by:
τ=F⋅h
- However, since the surface is smooth, the sphere will not roll, and the force will only contribute to the linear acceleration.
5. Conclusion:
- Since the linear acceleration acm is independent of the height h, we can conclude that the acceleration of the center of mass remains the same regardless of the value of h.
- Therefore, the correct answer is that the acceleration will be the same for any height h.
Final Answer:
The correct option is D: The acceleration will be the same regardless of the height h.
- We have a solid sphere of radius R resting on a smooth horizontal surface.
- A horizontal force F is applied at a height h from the lowest point of the sphere.
- The only external horizontal force acting on the sphere is F.
- The sphere has a mass m.
- The acceleration acm of the center of mass of the sphere can be calculated using Newton's second law:
acm=Fm
- This equation indicates that the acceleration of the center of mass depends solely on the net external force F and the mass m of the sphere.
- The height h at which the force is applied affects the torque about the center of mass but does not affect the linear acceleration of the center of mass.
- The torque τ caused by the force F is given by:
τ=F⋅h
- However, since the surface is smooth, the sphere will not roll, and the force will only contribute to the linear acceleration.
- Since the linear acceleration acm is independent of the height h, we can conclude that the acceleration of the center of mass remains the same regardless of the value of h.
- Therefore, the correct answer is that the acceleration will be the same for any height h.
The correct option is D: The acceleration will be the same regardless of the height h.
Similar Questions
A solid sphere of radius R is resting on a smooth horizontal surface. A constant force F is applied at a height h from the bottom. Choose the correct alternative.
View SolutionA uniform sphere is place on a smooth horizontal surface and as horizontal force F is appied on it at a distance h above the surface. The acceleratioin of the centre
View Solution
A solid sphere of mass ma nd radis R is placed on a rough horizontal surface A horizontal force F is applied to sphere at a height h, (0≤h≤R) from centre. If sphere rolls slipping then,
View Solution
A uniform solid cylinder of mass m and radius R is placed on a rough horizontal surface. A horizontal constant force F is applied at the top point P of the cylinder so that it start pure rolling. The acceleration of the cylinder is
View SolutionA sphere of mass m and radius r is placed on a rough plank of mass M. The system is placed on a smooth horizontal surface. A constant force F is applied on the plank such that the sphere rolls purely on the plank. Find the acceleration of the sphere.

View SolutionUniform solid cylinder of mass m and radius R is placed on a rough horizontal surface. A horizontal constant force is applied at the top point P of the cylinder so that it start pure rolling .In the above question, the frictional force on the cylinder is
View SolutionA solid sphere rests on a horizontal surface. A horizontal impulse is applied at height h from centre. The sphere starts rolling just after the application of impulse. The ratio h/R will be
View SolutionA solid sphere of mass M and radius R is placed on a rough horizontal surface. It is stuck by a horizontal cue stick at a height h above the surface. The value of h so that the sphere performs pure rolling motion immediately after it has been stuck is
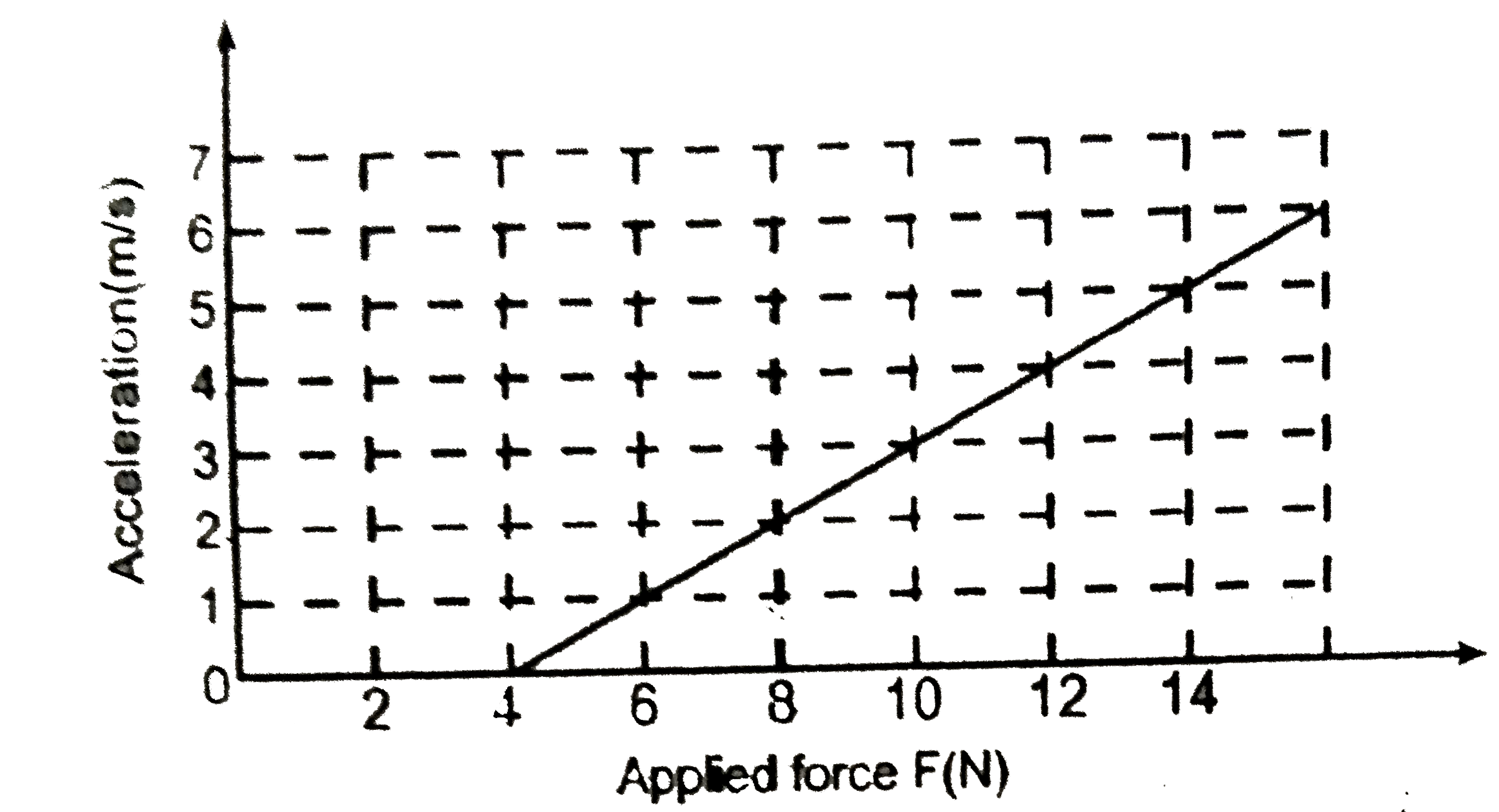
View SolutionA block of unknown mass is at rest on a rough, horizontal surface. A horizontal force F is applied to the block. The graph in the figure shows the acceleration of the block with respect to the applied force. The mass of the block is
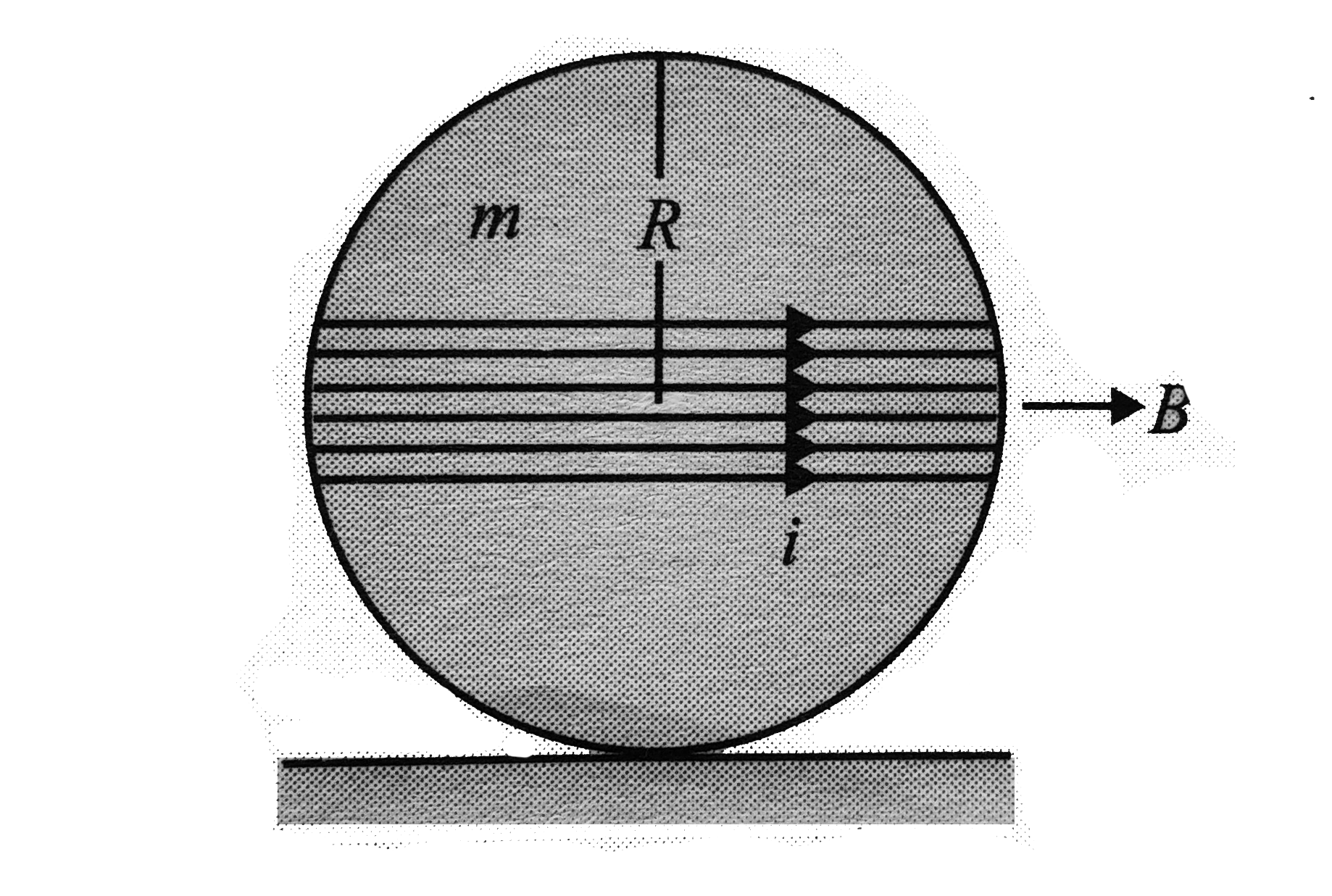
View SolutionA wire is wrapped N times over a solid sphere of mass m which is place on a smooth horizontal surface. A horizontal magnetic field of induction →B is present. Find the (a) torque (b) angular acceleration experienced by the sphere. Assume that the mass of the wire is negligible compared to the mass of the sphere.
View Solution
