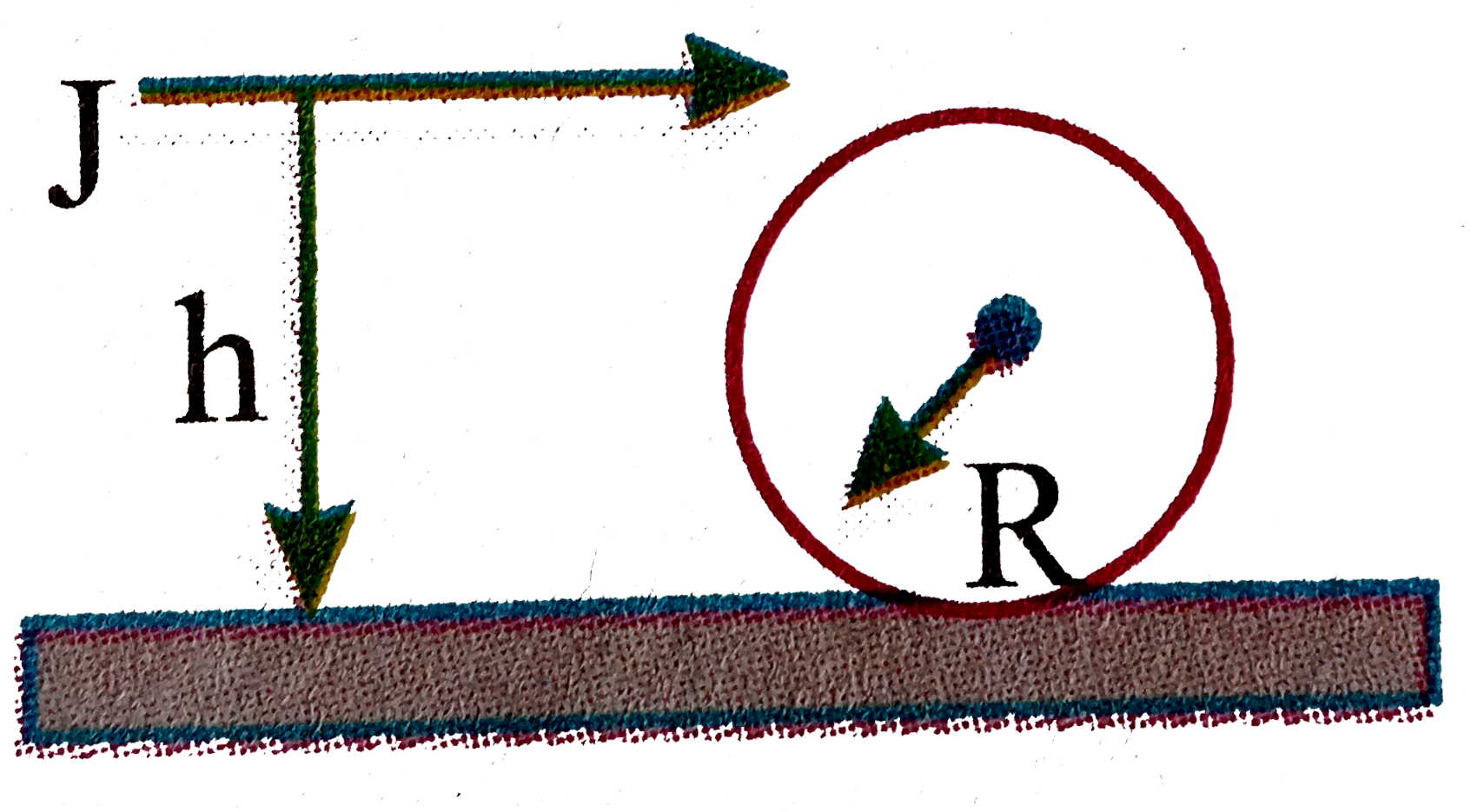
A uniform sphere is place on a smooth horizontal surface and as horizontal force F is appied on it at a distance h above the surface. The acceleratioin of the centre
is maximum when h=0
is maximum when h=R
is maximum when h=2R
is independent of h
The correct Answer is:D
To solve the problem, we need to analyze the situation where a uniform sphere is placed on a smooth horizontal surface and a horizontal force F is applied at a distance h above the surface. We want to determine the acceleration of the center of mass of the sphere.
1. Understand the Setup:
- We have a uniform sphere on a smooth horizontal surface.
- A horizontal force F is applied at a height h above the surface.
2. Identify the Forces:
- The only horizontal force acting on the sphere is the applied force F.
- There is no frictional force acting because the surface is smooth.
3. Analyze Motion:
- Since there is no friction, the sphere will not rotate; it will simply slide across the surface.
- The point of application of the force being at height h does not affect the horizontal motion because there is no friction to create a torque that would cause rotation.
4. Apply Newton's Second Law:
- According to Newton's second law, the acceleration a of the center of mass of the sphere is given by:
a=Fm
where m is the mass of the sphere.
5. Conclusion:
- The acceleration of the center of mass is independent of the height h at which the force is applied.
- Therefore, the final answer is:
a=Fm
- We have a uniform sphere on a smooth horizontal surface.
- A horizontal force F is applied at a height h above the surface.
- The only horizontal force acting on the sphere is the applied force F.
- There is no frictional force acting because the surface is smooth.
- Since there is no friction, the sphere will not rotate; it will simply slide across the surface.
- The point of application of the force being at height h does not affect the horizontal motion because there is no friction to create a torque that would cause rotation.
- According to Newton's second law, the acceleration a of the center of mass of the sphere is given by:
a=Fm
where m is the mass of the sphere.
- The acceleration of the center of mass is independent of the height h at which the force is applied.
- Therefore, the final answer is:
a=Fm
Topper's Solved these Questions
- CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONBOOK - HC VERMA ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONEXERCISE - Exercises
64 Videos
- CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONBOOK - HC VERMA ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONEXERCISE - Question For short Answer
25 Videos
- CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONBOOK - HC VERMA ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONEXERCISE - worked out Examples
23 Videos
- CALORIMETRYBOOK - HC VERMA ENGLISHCHAPTER - CALORIMETRYEXERCISE - Short answer
9 Videos
- CIRCULAR MOTIONBOOK - HC VERMA ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Question for short Answer
10 Videos
Similar Questions
A solid sphere of radius R is placed on a smooth horizontal surface. A horizontal force F is applied at height h from the lowest point. For the maximum acceleration of the centre of mass
View SolutionA solid sphere of radius R is placed on a smooth horizontal surface. A horizontal force F is applied at height h from the lowest point. For the maximum acceleration of the centre of mass
View SolutionA sphere of mass m and radius r is placed on a rough plank of mass M. The system is placed on a smooth horizontal surface. A constant force F is applied on the plank such that the sphere rolls purely on the plank. Find the acceleration of the sphere.

View SolutionA solid sphere of mass M and radius R is placed on a rough horizontal surface. It is stuck by a horizontal cue stick at a height h above the surface. The value of h so that the sphere performs pure rolling motion immediately after it has been stuck is
View SolutionA uniform cube of side and mass m rests on a rough horizontal surface. A horizontal force F is applied normal to one face at point that is directly above the centre of the face at a height a4 above the centre. The minimum value of F for which the cube begins to topple above an edge without sliding is
View Solution
A solid sphere of mass ma nd radis R is placed on a rough horizontal surface A horizontal force F is applied to sphere at a height h, (0≤h≤R) from centre. If sphere rolls slipping then,
View Solution
A uniform solid cylinder of mass m and radius R is placed on a rough horizontal surface. A horizontal constant force F is applied at the top point P of the cylinder so that it start pure rolling. The acceleration of the cylinder is
View SolutionA solid sphere of radius R is resting on a smooth horizontal surface. A constant force F is applied at a height h from the bottom. Choose the correct alternative.
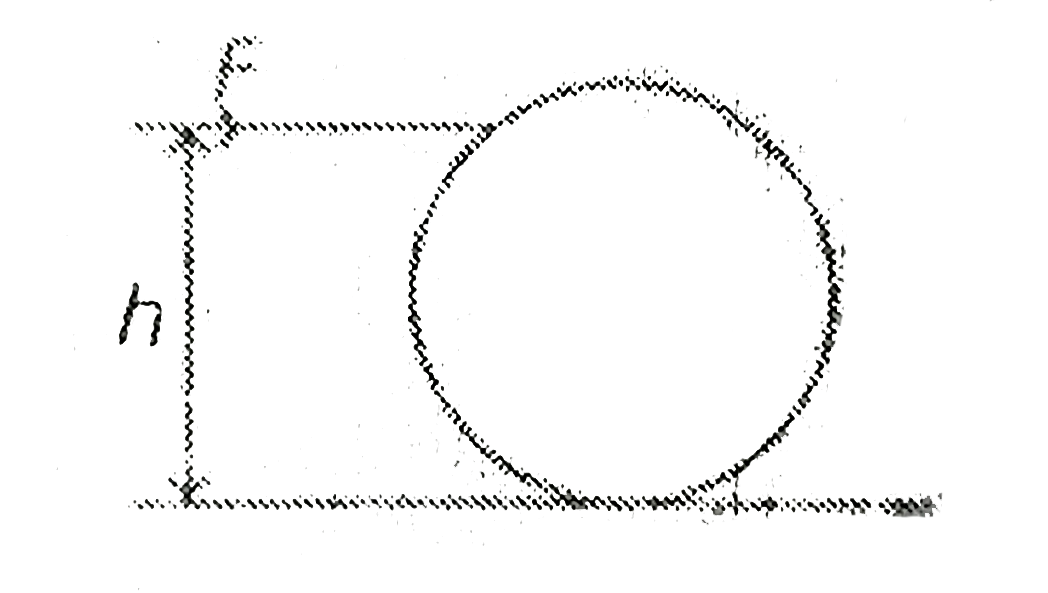
View SolutionThree identical cubes each of mass m are place on a smooth horizontal surface.A constant force F is applied on A as shown in the figure.The force on B applied by C is
View SolutionA hollow sphere of mass 'm' and radius 'R' are placed on horizontal surface and a horizontal force 'F' starts acting at a distance 'x' from its top most as shown in the figure.The value of 'x' such that hollow sphere starts pure rolling without use of friction is
_PHY_E05_157_Q01.png)
View Solution
