A pendulum is displaced to an angle θ from its equilibrium position, then it will pass through its mean position with a velocity v equal to
√2gl
√2glsinθ
√2glcosθ
√2gl(1−cosθ)
The correct Answer is:D
To solve the problem of finding the velocity v of a pendulum as it passes through its mean position after being displaced to an angle θ, we can use the principle of conservation of mechanical energy. Here’s a step-by-step solution:
Step 1: Understand the System
- A pendulum is displaced to an angle θ from its equilibrium position (mean position).
- The pendulum swings back to the mean position where we want to find the velocity v.
Step 2: Identify the Points
- Let point P be the maximum displacement (at angle θ).
- Let point B be the mean position (equilibrium position).
Step 3: Calculate the Height h
- The height h of the pendulum at point P can be calculated using the length L of the pendulum and the angle θ:
h=L−Lcos(θ)=L(1−cos(θ))
Step 4: Apply Conservation of Energy
- At point P (maximum displacement):
- Potential Energy (PE) = mgh
- Kinetic Energy (KE) = 0 (since it momentarily stops)
- At point B (mean position):
- Potential Energy (PE) = 0 (minimum)
- Kinetic Energy (KE) = 12mv2
Using conservation of energy:
PE at P=KE at B
mgh=12mv2
Step 5: Simplify the Equation
- The mass m cancels out from both sides:
gh=12v2
- Rearranging gives:
v2=2gh
Step 6: Substitute for h
- Substitute h=L(1−cos(θ)) into the equation:
v2=2gL(1−cos(θ))
- Taking the square root to find v:
v=√2gL(1−cos(θ))
Final Result
- Thus, the velocity v of the pendulum as it passes through the mean position is:
v=√2gL(1−cos(θ))
- A pendulum is displaced to an angle θ from its equilibrium position (mean position).
- The pendulum swings back to the mean position where we want to find the velocity v.
- Let point P be the maximum displacement (at angle θ).
- Let point B be the mean position (equilibrium position).
- The height h of the pendulum at point P can be calculated using the length L of the pendulum and the angle θ:
h=L−Lcos(θ)=L(1−cos(θ))
- At point P (maximum displacement):
- Potential Energy (PE) = mgh
- Kinetic Energy (KE) = 0 (since it momentarily stops)
- At point B (mean position):
- Potential Energy (PE) = 0 (minimum)
- Kinetic Energy (KE) = 12mv2
PE at P=KE at B
mgh=12mv2
- The mass m cancels out from both sides:
gh=12v2
- Rearranging gives:
v2=2gh
- Substitute h=L(1−cos(θ)) into the equation:
v2=2gL(1−cos(θ))
- Taking the square root to find v:
v=√2gL(1−cos(θ))
- Thus, the velocity v of the pendulum as it passes through the mean position is:
v=√2gL(1−cos(θ))
Similar Questions
A small circular coil with a mass of m consists of N turns of fine wire and carries a current of I. The coil is located in a uniform magnetic field of B with the coil axis orginally parallel to the field direction.
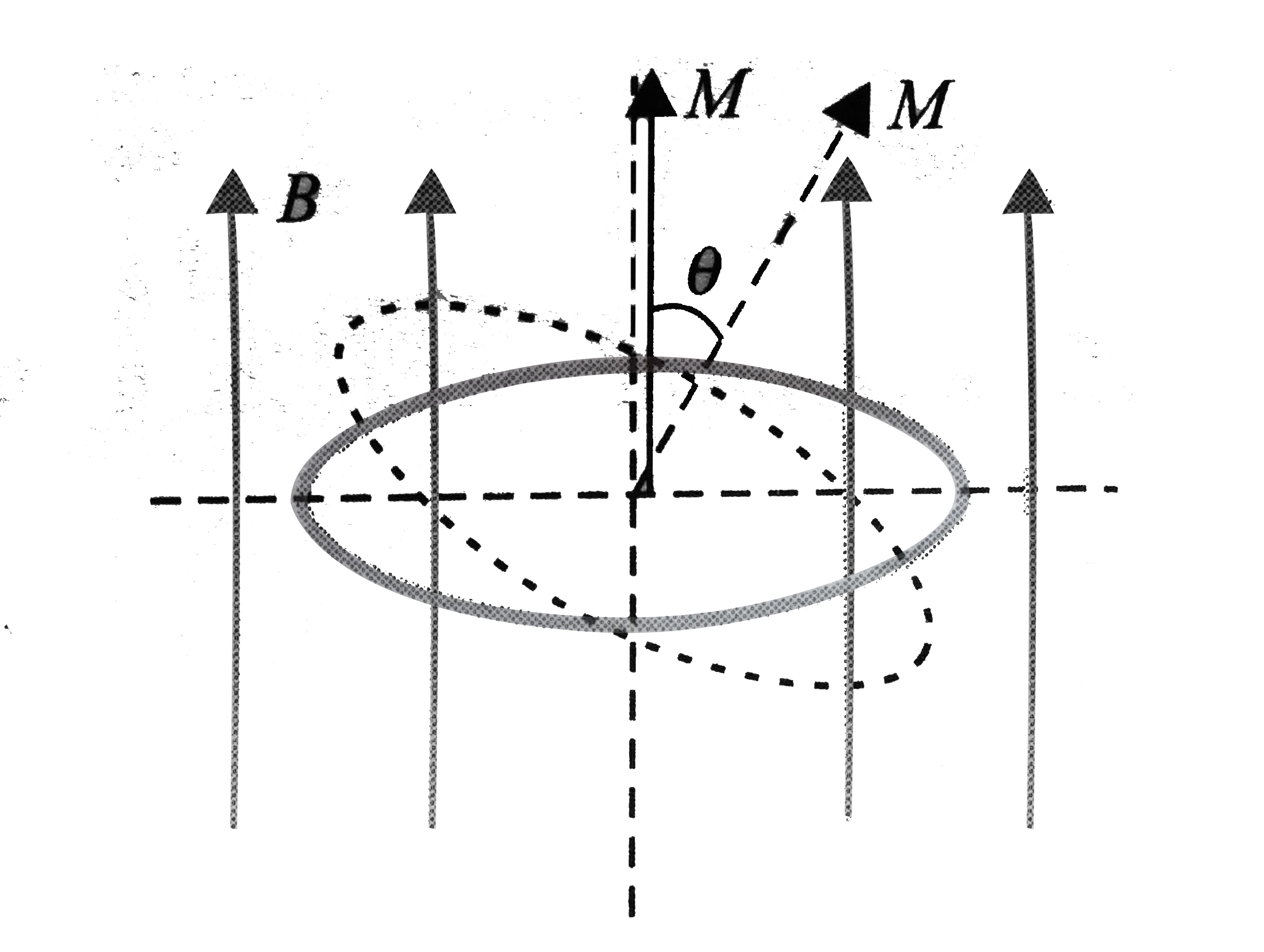
a. What is the period of small angle oscilations of the coil axis around its equilibrium position, assuming no friction and no mechanical force?
b. If the coil is rotated through an angle of θ from its equilibrium position and then released, what will be its angular speed when it passes through equilibrium position?View SolutionA small ball B of mass m is suspended with light inelastic string of length L from a block A of same mass in which can move on smooth horizontal surface as shown in the figure. The ball is displaced by angle θ from equilibrium position and then released.
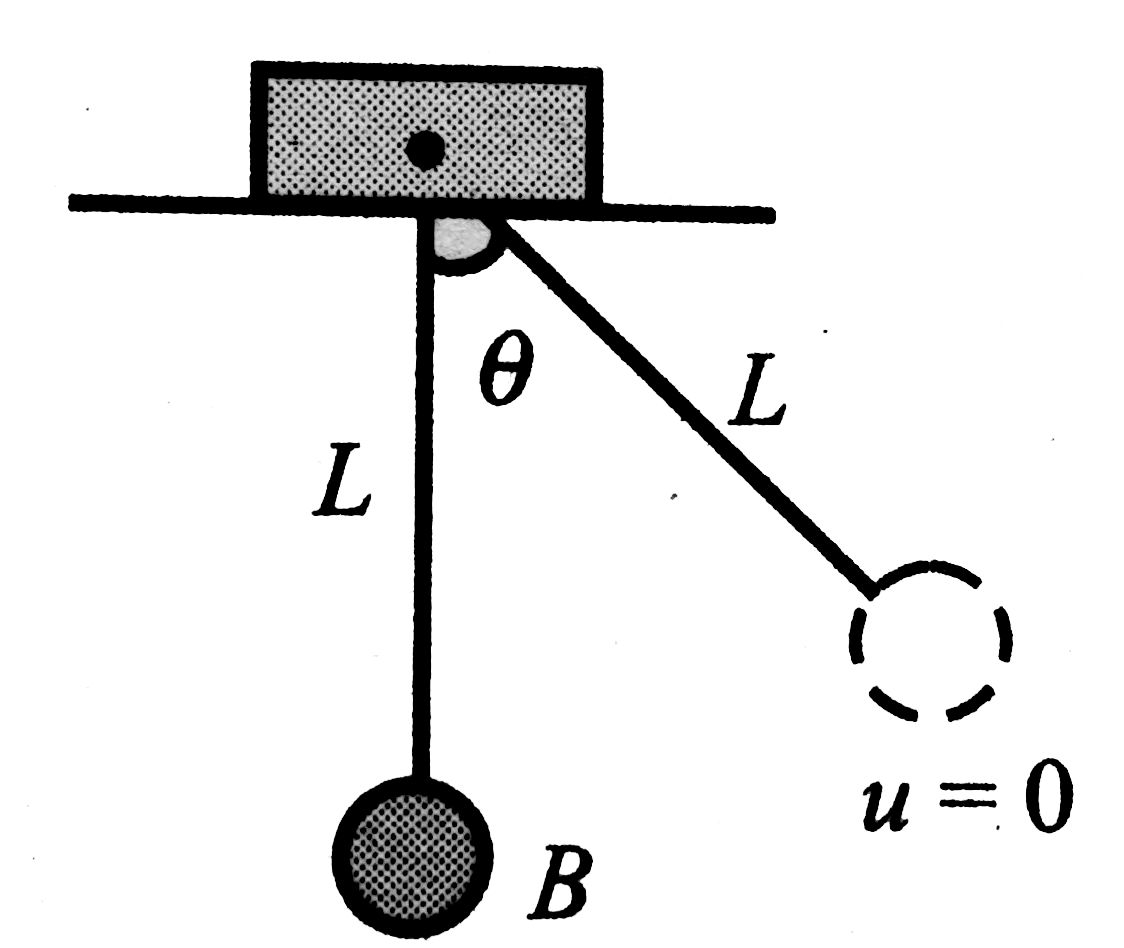
The displacement of block when equilibrium position isView SolutionA particle simple harmonic motion completes 1200 oscillations per minute and passes through the mean position with a velocity 3.14ms−1. Determine displacement of the particle from its mean position. Also obtain the displacement equation of the particle if its displacement be zero at the instant t=0.
View SolutionThe bob of a simple pendulum is displaced from its equilibrium position 'O' to a position 'Q' which is at a height 'h' above 'O' and the bob is then released. Assuming the mass of the bob to be 'm' and time period of oscillation to be 2.0s, the tension in the string when the bob passes through 'O' is
View SolutionThe bob of a simple pendulum is displaced from its equilibrium position 'O' to a position 'Q' which is at a height 'h' above 'O' and the bob is then released. Assuming the mass of the bob to be 'm' and time period of oscillation to be 2.0s, the tension in the string when the bob passes through 'O' is
View SolutionA particle executes shm in a line 10 cm long. When it passes through the mean position its velocity is 15cms−1 . Find the period.
View SolutionA wagon of mass M can move without friction along horizontal rails. A simple pendulum consisting of a sphere of mass m is suspended from the ceiling of the wagon by a string of length l. At the initial moment the wagon and the pendulum are at rest and the string is deflected through an angle α from the vertical. Find the velocity of the wagon when the pendulum passes through its mean position.
View SolutionA simple pendulum ,while oscillating ,rises to a maximum vertical height of 5 cm from its rest position when it reaches to its extreme position on one side.If mass of the bob of the simple pendulum is 500 g and g-10 m s−2 find :
(i)the total energy of simple pendulum at any instant while oscillating ,and
(ii)the velocity of bob at its mean position State the assumption made in your calculation .View SolutionIf the second pendulum bob is thrown at velocity v2 at an angle β from mean position, but on other side of mean position , find the phase diffrence in the two SHMs now as show in the figure.
 View Solution
View SolutionFind the time period of mass M when displaced from its equilibrium position and then released for the system shown in figure.
 View Solution
View Solution

