A conducting sphere of radius R is given a charge Q. The electric potential and the electric field at the centre of the sphere respectively are
zero and Q4πε0R2
Q4πε0R and zero
Q4πε0R and Q4πε0R2
Both are zero
The correct Answer is:B
To solve the problem, we need to determine the electric potential and the electric field at the center of a conducting sphere of radius R that has been given a charge Q.
1. Understanding Charge Distribution:
- When a conducting sphere is charged, the charge Q distributes itself uniformly on the outer surface of the sphere due to mutual repulsion between the charges.
- Inside the conducting material and at the center, there are no free charges.
2. Electric Field Inside the Sphere:
- According to Gauss's law, the electric field E inside a conductor in electrostatic equilibrium is zero.
- Since there are no charges inside the sphere, we can conclude that the electric field at the center of the sphere is:
E=0(inside the conducting sphere)
3. Electric Potential Inside the Sphere:
- The electric potential V inside a conductor is constant throughout its volume. This means that the potential at any point inside the conducting sphere, including the center, is the same as the potential at the surface of the sphere.
- The potential at the surface of a charged sphere is given by the formula:
V=KQR
where K=14πϵ0.
- Therefore, the potential at the center of the sphere is:
V=KQR=14πϵ0⋅QR
4. Final Results:
- The electric field at the center of the conducting sphere is:
E=0
- The electric potential at the center of the conducting sphere is:
V=14πϵ0⋅QR
Summary:
- Electric Field at the center: E=0
- Electric Potential at the center: V=14πϵ0⋅QR
- When a conducting sphere is charged, the charge Q distributes itself uniformly on the outer surface of the sphere due to mutual repulsion between the charges.
- Inside the conducting material and at the center, there are no free charges.
- According to Gauss's law, the electric field E inside a conductor in electrostatic equilibrium is zero.
- Since there are no charges inside the sphere, we can conclude that the electric field at the center of the sphere is:
E=0(inside the conducting sphere)
- The electric potential V inside a conductor is constant throughout its volume. This means that the potential at any point inside the conducting sphere, including the center, is the same as the potential at the surface of the sphere.
- The potential at the surface of a charged sphere is given by the formula:
V=KQR
where K=14πϵ0.
- Therefore, the potential at the center of the sphere is:
V=KQR=14πϵ0⋅QR
- The electric field at the center of the conducting sphere is:
E=0
- The electric potential at the center of the conducting sphere is:
V=14πϵ0⋅QR
- Electric Field at the center: E=0
- Electric Potential at the center: V=14πϵ0⋅QR
Similar Questions
A conducting sphere of radius R is charged to a potential of V volts. Then the electric field at a distance r(>R) from the centre of the sphere would be
View SolutionA hollow insulated conducting sphere is given a positive charge of 10μC . What will be the electric field at the centre of the sphere it is radius is 2 metres ?
View SolutionA thin conducting ring of radius R is given a charge +Q, Fig. The electric field at the center O of the ring due to the charge on the part AKB of the ring is E. The electric field at the center due to the charge on part ACDB of the ring is

View SolutionBoth the ring and the conducting sphere are given the same charge Q. Determine the potential of the sphere. Assume that the centre of the sphere lies on the axis of th ring. Is it necessary that the charge on the ring be uniformly distributed to answer the above question ?
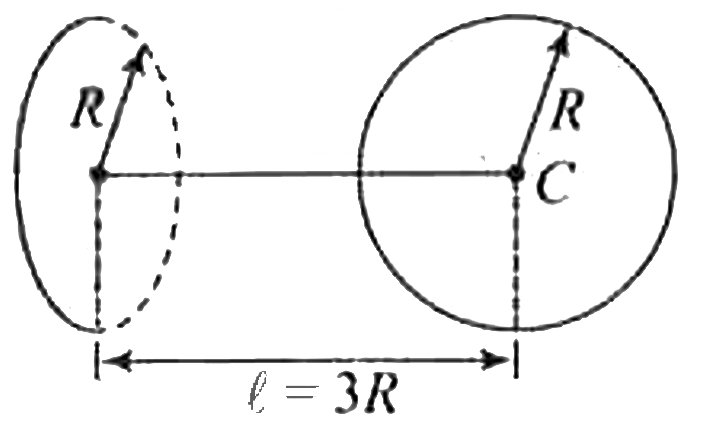
View Solutionn charged drops, each of radius r and charge q, coalesce to from a big drop of radius R and charge Q. If V is the electric potential and E is the electric field at the surface of a drop, then.
View SolutionA non-conducting sphere of radius R is given a charge Q. Charge is uniformly distributed in its volume. The electric potential at centre and at the surface of sphere respectively are
View SolutionAn isolated solid metal sphere of radius R is given an electric charge. The variation of the intensity of the electric field with the distance r from the centre of the sphere is best shown by
View SolutionAt a point 20cm from the centre of a uniformly charged dielectric sphere of radius 10cm, the electric field is 100V/m. The electric field at 3cm from the centre of the sphere will be
View SolutionA non-conducting solid sphere of radius R is uniformly charged. The magnitude of the electric filed due to the sphere at a distance r from its centre
View SolutionA solid insulating sphere of radius R is given a charge Q. If at a point inside the sphere the potential is 1.5 times the potential at the surface, this point will be
View Solution