Four point charges −Q,−q,2q and 2Q are placed, one at each corner of the square. The relation between Q and q for which the potential at the centre of the square is zero is
Q=−q
Q=−1q
Q=q
Q=1q
The correct Answer is:A
To find the relation between the charges Q and q such that the potential at the center of the square formed by the charges is zero, we can follow these steps:
Step 1: Identify the Configuration
We have four point charges placed at the corners of a square:
- Charge at corner 1: −Q
- Charge at corner 2: −q
- Charge at corner 3: 2q
- Charge at corner 4: 2Q
Step 2: Determine the Distance from the Center
The distance from the center of the square to each corner can be calculated using the diagonal of the square. If the side length of the square is a, the diagonal d is given by:
d=a√2
The distance from the center to a corner (which is half the diagonal) is:
r=d2=a√22=a√2
Step 3: Calculate the Potential at the Center
The electric potential V at a point due to a point charge is given by:
V=kqr
where k is Coulomb's constant. The total potential at the center due to all four charges is the sum of the potentials due to each charge.
1. Potential due to −Q:
V−Q=k−Qa√2=−kQ√2a
2. Potential due to −q:
V−q=k−qa√2=−kq√2a
3. Potential due to 2q:
V2q=k2qa√2=k2q√2a
4. Potential due to 2Q:
V2Q=k2Qa√2=k2Q√2a
Step 4: Sum the Potentials
Now, we sum the potentials at the center:
Vtotal=V−Q+V−q+V2q+V2Q
Substituting the expressions we derived:
Vtotal=−kQ√2a−kq√2a+k2q√2a+k2Q√2a
Step 5: Factor Out Common Terms
Factoring out k√2a:
Vtotal=k√2a(−Q−q+2q+2Q)
This simplifies to:
Vtotal=k√2a(Q+q)
Step 6: Set the Total Potential to Zero
For the potential at the center to be zero:
k√2a(Q+q)=0
Since k√2a is not zero, we have:
Q+q=0
Thus, we find:
Q=−q
Final Relation
The relation between Q and q for which the potential at the center of the square is zero is:
Q=−q
We have four point charges placed at the corners of a square:
- Charge at corner 1: −Q
- Charge at corner 2: −q
- Charge at corner 3: 2q
- Charge at corner 4: 2Q
The distance from the center of the square to each corner can be calculated using the diagonal of the square. If the side length of the square is a, the diagonal d is given by:
d=a√2
The distance from the center to a corner (which is half the diagonal) is:
r=d2=a√22=a√2
The electric potential V at a point due to a point charge is given by:
V=kqr
where k is Coulomb's constant. The total potential at the center due to all four charges is the sum of the potentials due to each charge.
V−Q=k−Qa√2=−kQ√2a
V−q=k−qa√2=−kq√2a
V2q=k2qa√2=k2q√2a
V2Q=k2Qa√2=k2Q√2a
Now, we sum the potentials at the center:
Vtotal=V−Q+V−q+V2q+V2Q
Substituting the expressions we derived:
Vtotal=−kQ√2a−kq√2a+k2q√2a+k2Q√2a
Factoring out k√2a:
Vtotal=k√2a(−Q−q+2q+2Q)
This simplifies to:
Vtotal=k√2a(Q+q)
For the potential at the center to be zero:
k√2a(Q+q)=0
Since k√2a is not zero, we have:
Q+q=0
Thus, we find:
Q=−q
The relation between Q and q for which the potential at the center of the square is zero is:
Q=−q
Similar Questions
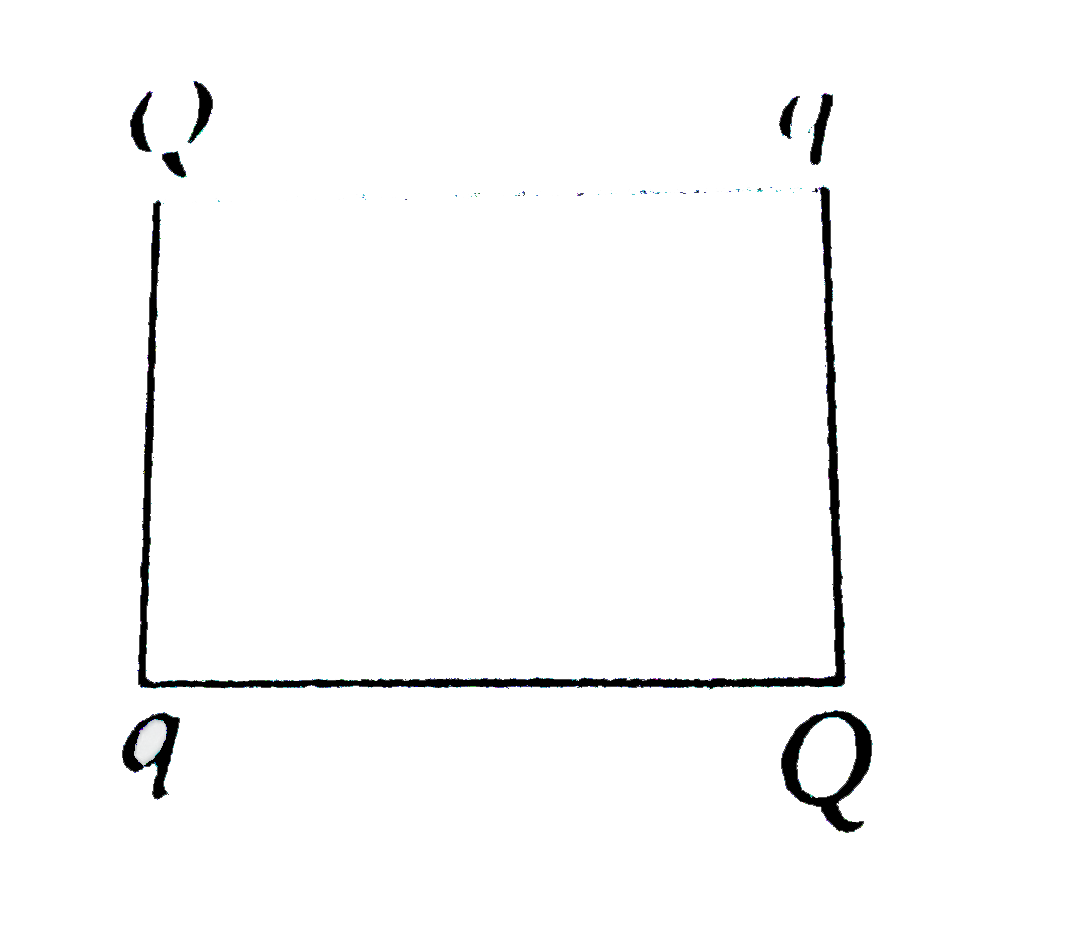
Four charge Q,q,Q and q are kept at the four corners of a square as shown below. What is the relation between Q and q, so that the net force on a charge q is zero ?
View SolutionCharges q,2q,3q and 4q are placed at the corners A,B,C and D of a square as shown in the following figure. The directon of electric field at the centre of the square is along

View SolutionCharges q,2q,3q and 4q are placed at the corners A,B,C and D of a square as shown in the following figure. The directon of electric field at the centre of the square is along

View SolutionFour charges of +q, +q +q and +q are placed at the corners A, B, C and D of s square. The resultant force on the charge at D
View SolutionA charge Q is placed at each corner of a cube of side a. The potential at the centre of the cube is
View SolutionFour point charge q,−q,2Q and Q are placed in order at the corners A, B, C and D of a square. If the field at the midpoint of CD is zero then the value of q/Q is 5√5x. Find the value of x.
View SolutionFour charges of +q, +q +q and +q are placed at the corners A, B, C and D of s square. The resultant force on the charge at D
View SolutionIf identical charges (−q) are placed at each corner of a cube of side b, then electric potential energy of charge (+q) which is placed at centre of the cube will be
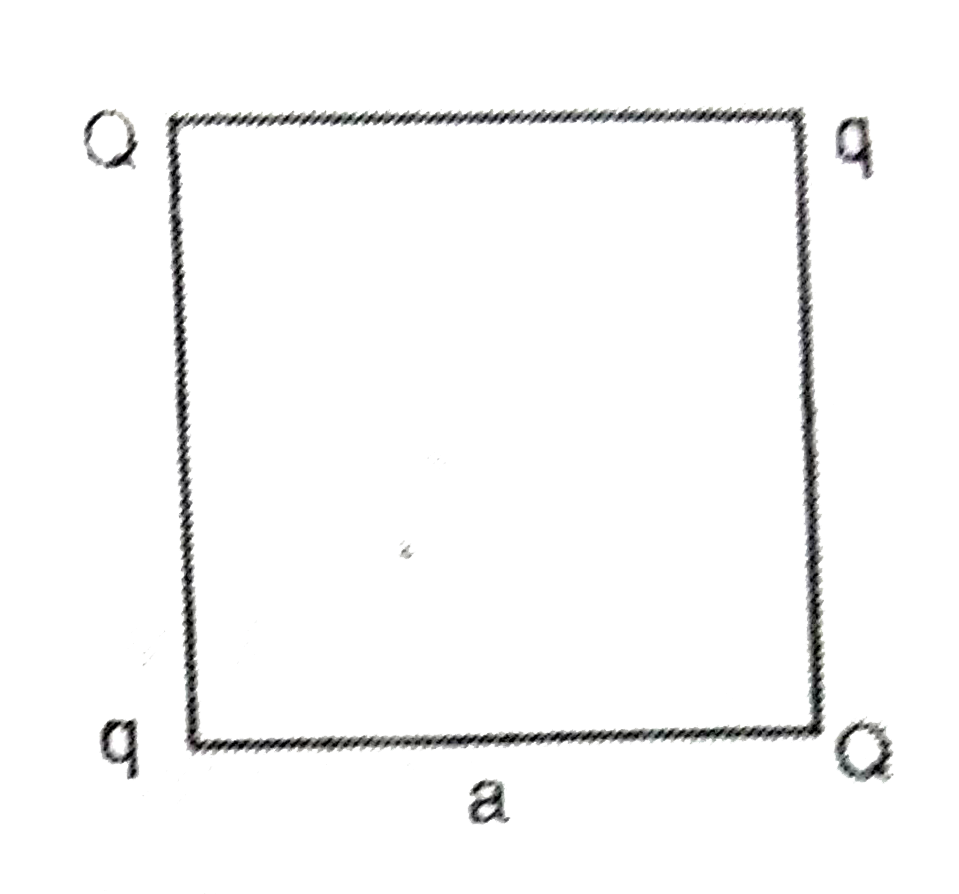
View SolutionFour point charges Q, q, Q and q are placed at the corners of a square of side' a' as shown in the figure.
Find the .
(a) resultnat electric force on a charge Q and .
(b) potential energy of this system.
View SolutionFour identical charges each .q. are placed at four corners of a square of side .a.. Find the charge to be placed at the centre of the square so that the system of charges is in equilibrium
View Solution
