A parallel plate condenser with oil (dielectric constant 2) between the plates has capacitance C. If oil is removed, the capacitance of capacitor becomes
√2C
2c
C√2
C2
The correct Answer is:D
To solve the problem, we need to understand how the capacitance of a parallel plate capacitor changes when a dielectric material is removed.
1. Understand the formula for capacitance with a dielectric:
The capacitance C of a parallel plate capacitor with a dielectric material is given by the formula:
C=k⋅ϵ0⋅Ad
where:
- k is the dielectric constant,
- ϵ0 is the permittivity of free space,
- A is the area of the plates,
- d is the separation between the plates.
2. Identify the given values:
We are given that the capacitance with oil (dielectric constant k=2) is C. Therefore, we can express this as:
C=2⋅ϵ0⋅Ad
3. Calculate the capacitance without the dielectric:
When the oil is removed, the dielectric constant k becomes 1 (since air or vacuum has a dielectric constant of 1). The new capacitance C′ can be expressed as:
C′=1⋅ϵ0⋅Ad=ϵ0⋅Ad
4. Relate the new capacitance to the original capacitance:
From the first equation, we know:
C=2⋅ϵ0⋅Ad
Thus, we can express ϵ0⋅Ad in terms of C:
ϵ0⋅Ad=C2
5. Substituting back to find the new capacitance:
Now substituting this back into the equation for C′:
C′=ϵ0⋅Ad=C2
6. Conclusion:
Therefore, the capacitance of the capacitor after the oil is removed becomes:
C′=C2
Final Answer:
The capacitance of the capacitor after the oil is removed is C2.
---
The capacitance C of a parallel plate capacitor with a dielectric material is given by the formula:
C=k⋅ϵ0⋅Ad
where:
- k is the dielectric constant,
- ϵ0 is the permittivity of free space,
- A is the area of the plates,
- d is the separation between the plates.
We are given that the capacitance with oil (dielectric constant k=2) is C. Therefore, we can express this as:
C=2⋅ϵ0⋅Ad
When the oil is removed, the dielectric constant k becomes 1 (since air or vacuum has a dielectric constant of 1). The new capacitance C′ can be expressed as:
C′=1⋅ϵ0⋅Ad=ϵ0⋅Ad
From the first equation, we know:
C=2⋅ϵ0⋅Ad
Thus, we can express ϵ0⋅Ad in terms of C:
ϵ0⋅Ad=C2
Now substituting this back into the equation for C′:
C′=ϵ0⋅Ad=C2
Therefore, the capacitance of the capacitor after the oil is removed becomes:
C′=C2
The capacitance of the capacitor after the oil is removed is C2.
Similar Questions
A parallel plate capacitor with liquid filled between the plates (dielectric constant of liquid, k=4)has capacitance 20μF. If the liquid is removed then capacitance of capacitor becomes
View SolutionA parallel plate capacitor with air between the plates has a capacitance C. If the distance between the plates is doubled and the space between the plates is filled with a dielectric of dielectric constant 6, then the capacitance will become.
View Solution
Knowledge Check
A parallel plate condenser with a dielectric of dielectric constant K between the plates has a capacity C and is charged to a potential V volt. The dielectric slab is slowly removed from between the plates and then reinserted. The net work done by the system in this process is
AzeroB12(K−1)CV2CCV2(K−1)KD(K−1)CV2A parallel plate capacitor with air between the plates has a capacitance of 10 pF. The capacitance, if the distance bgetween the plates is reduced by half and the space between tehm is filled with a substance of dielectric constant 4 is
A80pFB96pFC100pFD120pF
A parallel plate capacitor with air between the plates has a capacitance of 8 pF.(1pF=10−12F) What will be the capacitance if the distance between the plates is reduced by half and the space between them is filled with a substance of dielectric constant 6 ?
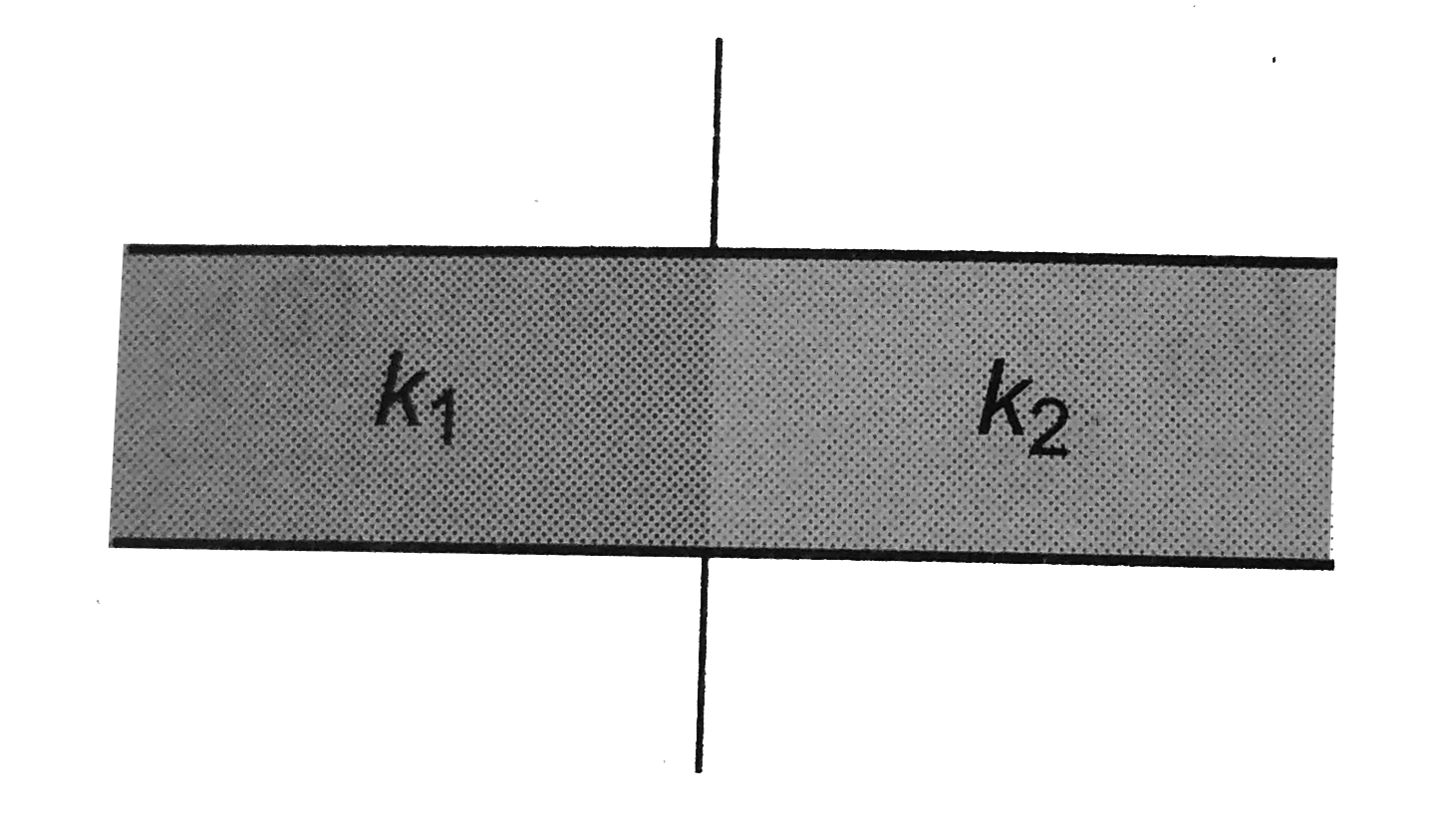
View SolutionA parallel plate capacitor with air as medium between the plates has a capacitance of 10μF. The area of capacitor is divided into two equal halves and filled with two media as shown in the figure having dielectric constnt k1=2 and k2=4. the capacitance of the system will now be
View SolutionA parallel plate capacitor has a dielectric (of dielectric constant k) between the plates. The plates are charged to a surface charge density σ0. What is the density of the polarized charge that appear on the surface of the dielectric.
View SolutionThe capacitor shown has square plates of side L and has gap d between the photos. In the dielectric constant of the materiale of the slab is k , the capacitance of the capacitor is

View SolutionAssertion : If the distance between parallel plates of a capacitor is halved and dielectric constant is made three times, then the capacitance becomes 6 times.
Reason : Capacity of the capacitor depends upon the nature of the material between the plates.
View SolutionA parallel plate capacitor with air between the plates has capacitance of 9pF. The separation between its plates is 'd'. The space between the plates is now filled with two dielectrics. One of the dielectrics has dielectric constant k1=3 and thickness d3 while the other one has dielectric constant k2=6 and thickness 2d3. Capacitance of the capacitor is now
View Solution
