A charge q is placed at the centre of the line joining two equal charges Q. The system of the three charges will be in equilibrium if q is equal to:
−Q4
+Q
−Q
Q2
The correct Answer is:A
To find the value of charge q that will keep the system of three charges in equilibrium, we can follow these steps:
Step 1: Understand the arrangement of charges
We have two equal charges Q placed at a distance L apart, and a charge q is placed at the midpoint of the line joining these two charges. The distances from q to each Q are L2.
Step 2: Determine the forces acting on charge q
- The charge q will experience a repulsive force F1 due to one of the charges Q and an attractive force F2 due to the other charge Q if q is negative.
Step 3: Calculate the forces
1. Repulsive force F1 (from one Q):
F1=kQ⋅q(L2)2=kQ⋅qL24=4kQqL2
2. Attractive force F2 (from the other Q):
F2=kQ⋅q(L2)2=kQ⋅qL24=4kQqL2
Step 4: Set the forces equal for equilibrium
For the system to be in equilibrium, the magnitudes of the forces must be equal:
F1=F2
Since both forces are equal in magnitude but opposite in direction, we can set them equal:
4kQqL2=4kQ(−q)L2
Step 5: Solve for q
Since both sides are equal, we can simplify:
4kQq=−4kQq
This implies:
4kQq+4kQq=0⟹8kQq=0
Since k and Q are not zero, we can divide both sides by 4kQ:
q=−Q4
Conclusion
Thus, the charge q must be equal to:
q=−Q4
We have two equal charges Q placed at a distance L apart, and a charge q is placed at the midpoint of the line joining these two charges. The distances from q to each Q are L2.
- The charge q will experience a repulsive force F1 due to one of the charges Q and an attractive force F2 due to the other charge Q if q is negative.
1. Repulsive force F1 (from one Q):
F1=kQ⋅q(L2)2=kQ⋅qL24=4kQqL2
F2=kQ⋅q(L2)2=kQ⋅qL24=4kQqL2
For the system to be in equilibrium, the magnitudes of the forces must be equal:
F1=F2
Since both forces are equal in magnitude but opposite in direction, we can set them equal:
4kQqL2=4kQ(−q)L2
Since both sides are equal, we can simplify:
4kQq=−4kQq
This implies:
4kQq+4kQq=0⟹8kQq=0
Since k and Q are not zero, we can divide both sides by 4kQ:
q=−Q4
Thus, the charge q must be equal to:
q=−Q4
Similar Questions
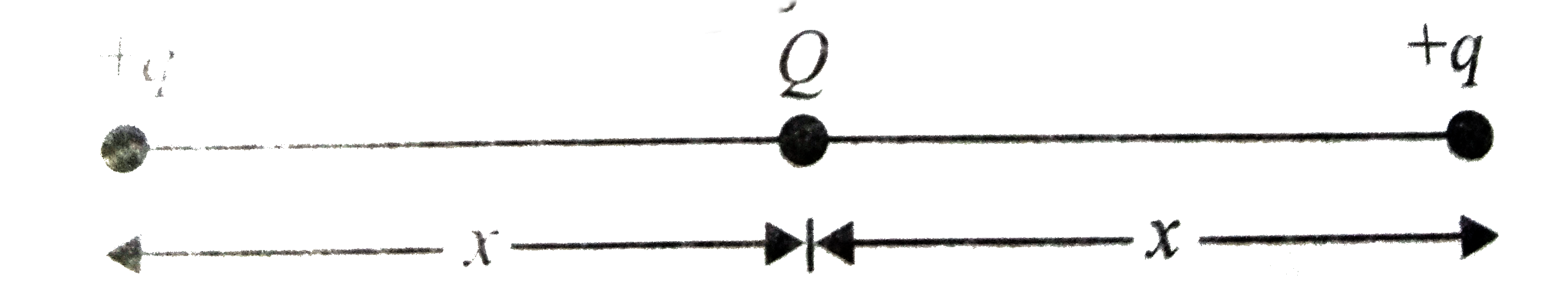
A charge Q is placed at the centre of the line joining two point charges +q and +q as shown in figure. The ratio of charges Q and q is
View SolutionTwo idential point charges Q are kept at a distance r from each other. A third point charge is placed on the line joing the above two charges such that all the three charges are in equilibrium. The third charge
(a) should be of magnitude q=………..
(b) should be of sign .......
(c ) should be placed ........ .
View SolutionIf Two charges +qand+4q are separated by a distance d and a point charge Q is placed on the line joining the above two charges and in between them such that all charges are in equilibrium. Then the charge Q and it's position are
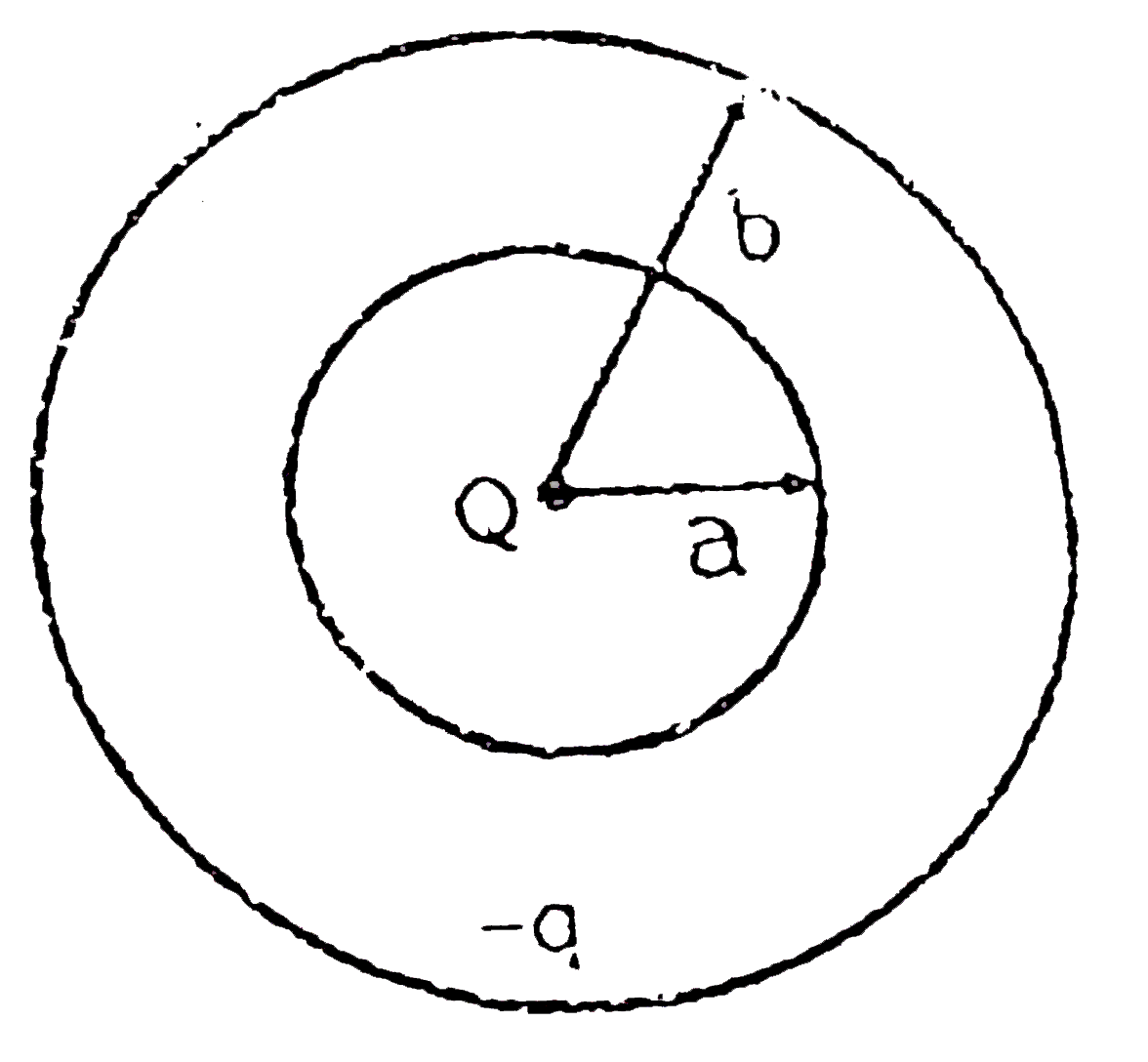
View SolutionShown in the figure a spherical shell with an inner radius 'a' and an outer radius 'b' is made of conducting metarial. A charge +Q is placed at the centre of the spherical shell and a total charge -q s placed on the shell. Charge -q is districuted on the surfaces as :
View SolutionA thin metallic spherical shell contains a charge Q on it. A point charge q is placed at the center of the shell and another charge q1 is placed outside it as shown in the figure. All the three charges are positive. Find the force on the charge

a. at centre due to all charges.
b. at center due to shell.
View SolutionTwo point charges +q and −q are placed a distance x apart. A third charge is so placed that at the three charges are in equilibrium. Then
View SolutionThree positive charges of equal magnitude q are placed at the vertics of and equilatral triangle of side l. How can the system of charges be palced in equilibrium?
View SolutionAssertion At the centre of the line joining two equal and opposite charges, E=0
Reason At the centre of the line joining two equal and similar charge, E≠0.
View SolutionA charge Q is place at each of the opposite corners of a square. A charge q is placed at each of the other two corners. If the net electrical force on Q is zero, then Q/q equals:
View SolutionA thin. Metallic spherical shell contains charge Q on it. A point charge q is placed at the centre of the shell and another charge q1 is placed outside it as shown in figure . All the three charges are positive. The force on the charge at the centre is

View Solution
