Assertion At the centre of the line joining two equal and opposite charges, E=0
Reason At the centre of the line joining two equal and similar charge, E≠0.
If both Assertion and Reason are correct and Reason is the correct explanation of Assertion
If both Assertion and Reason are correct but Reason is not the correct explanation of Assertion
If Assertion is true but Reason is false
If both Assertion and Reason are false
To solve the assertion and reason question, we will analyze both statements step by step.
Step 1: Analyze the Assertion
Assertion: At the center of the line joining two equal and opposite charges, E=0.
1. Define the Charges: Let’s denote the two charges as +Q and −Q.
2. Positioning the Charges: Place +Q at point A and −Q at point B, with a distance R between them.
3. Midpoint Calculation: The midpoint P is located at a distance R2 from both charges.
4. Electric Field Contribution:
- The electric field E1 due to the positive charge +Q at point P will be directed away from +Q (to the right).
- The electric field E2 due to the negative charge −Q at point P will be directed towards −Q (also to the right).
5. Net Electric Field Calculation: Since both electric fields at point P are in the same direction, the net electric field E at point P is given by:
E=E1+E2≠0
6. Conclusion for Assertion: Therefore, the assertion is false since E is not equal to zero.
Step 2: Analyze the Reason
Reason: At the center of the line joining two equal and similar charges, E≠0.
1. Define the Similar Charges: Let’s denote the two similar charges as +Q and +Q.
2. Positioning the Charges: Place both charges at points A and B, with a distance R between them.
3. Midpoint Calculation: The midpoint P is again located at a distance R2 from both charges.
4. Electric Field Contribution:
- The electric field E1 due to the first charge +Q at point P will be directed away from +Q (to the right).
- The electric field E2 due to the second charge +Q at point P will also be directed away from +Q (to the left).
5. Net Electric Field Calculation: Since the electric fields are equal in magnitude but opposite in direction, the net electric field E at point P is given by:
E=E1−E2=0
6. Conclusion for Reason: Therefore, the reason is also false since E is equal to zero.
Final Conclusion
Both the assertion and the reason are false.
Assertion: At the center of the line joining two equal and opposite charges, E=0.
2. Positioning the Charges: Place +Q at point A and −Q at point B, with a distance R between them.
3. Midpoint Calculation: The midpoint P is located at a distance R2 from both charges.
4. Electric Field Contribution:
- The electric field E1 due to the positive charge +Q at point P will be directed away from +Q (to the right).
- The electric field E2 due to the negative charge −Q at point P will be directed towards −Q (also to the right).
5. Net Electric Field Calculation: Since both electric fields at point P are in the same direction, the net electric field E at point P is given by:
E=E1+E2≠0
6. Conclusion for Assertion: Therefore, the assertion is false since E is not equal to zero.
Reason: At the center of the line joining two equal and similar charges, E≠0.
2. Positioning the Charges: Place both charges at points A and B, with a distance R between them.
3. Midpoint Calculation: The midpoint P is again located at a distance R2 from both charges.
4. Electric Field Contribution:
- The electric field E1 due to the first charge +Q at point P will be directed away from +Q (to the right).
- The electric field E2 due to the second charge +Q at point P will also be directed away from +Q (to the left).
5. Net Electric Field Calculation: Since the electric fields are equal in magnitude but opposite in direction, the net electric field E at point P is given by:
E=E1−E2=0
6. Conclusion for Reason: Therefore, the reason is also false since E is equal to zero.
Both the assertion and the reason are false.
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Match the columns
5 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Medical entrances gallery
37 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Taking it together
105 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions
135 Videos
Similar Questions
A charge q is placed at the centre of the line joining two equal charges Q. The system of the three charges will be in equilibrium if q is equal to:
View SolutionA charge q is placed at the centre of the line joining two equal charges Q. The system of the three charges will be in equilibrium if q is equal to:
View SolutionA charge q is placed at the centre of the line joining two equal charges Q. The system of the three charges will be in equilibrium if q is equal to:
View SolutionA charge Q is placed at the centre of the line joining two point charges +q and +q as shown in figure. The ratio of charges Q and q is
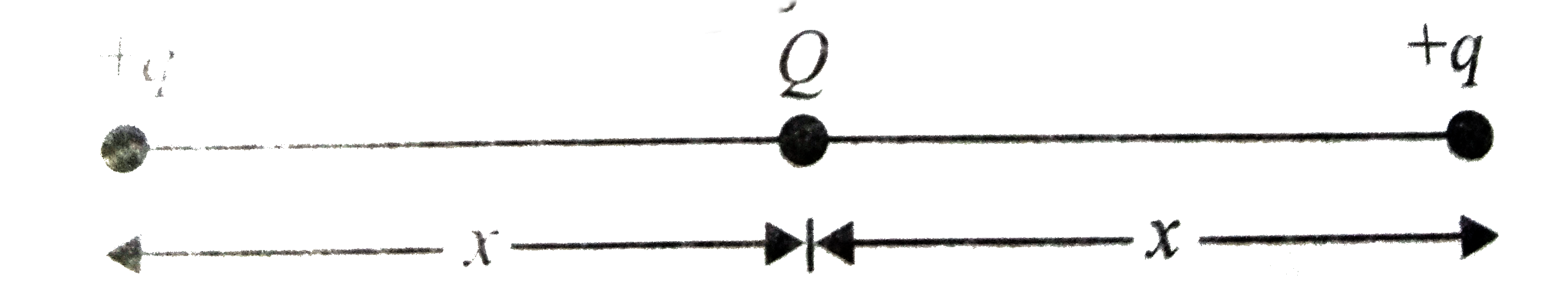
View Solution(A ): A charge is lying at the centre of the line joining two similar charges each which are fixed. The system will be in equilibrium if that charge is one fourth of the similar charges.
(R ): For charge to be in equilibrium, sum of the forces on charge due to rest of the two charges must be zero.
View SolutionTwo charges −10C and +10C are placed 10 cm apart. Potential at the centre of th eline joining the two charges is
View SolutionProve that the line joining the mid-points of two parallel chords of a circle passes through the centre.
View SolutionProve that the line joining the mid-points of two parallel chords of a circle passes through the centre.
View SolutionProve that the line joining the mid-points of two parallel chords of a circle passes through the centre.
View SolutionShow that the line joining the points (4,−3) and (0,7) is perpendicular to the line joining the points (5,2) and (0,0).
View Solution
