There are three copper wires of length and cross-sectional area (L,A),(2L,A/2)(L/2,2A). In which case in the resistance minimum?
it is the same in all three cases
Wire of cross-sectional are 2A
Wire of cross-sectional area A
Wire of cross-sectional are 12A
The correct Answer is:B
To find out in which case the resistance is minimum among the three copper wires, we can use the formula for resistance:
R=ρLA
where:
- R is the resistance,
- ρ is the resistivity of the material (which is constant for copper),
- L is the length of the wire,
- A is the cross-sectional area of the wire.
1. Identify the parameters for each wire:
- Wire 1: Length L, Area A
- Wire 2: Length 2L, Area A2
- Wire 3: Length L2, Area 2A
2. Calculate the resistance for each wire:
- Resistance of Wire 1:
R1=ρLA
- Resistance of Wire 2:
R2=ρ2LA2=ρ2L⋅2A=ρ4LA
- Resistance of Wire 3:
R3=ρL22A=ρL4A
3. Compare the resistances:
- From the calculations:
- R1=ρLA
- R2=ρ4LA
- R3=ρL4A
4. Determine which resistance is the smallest:
- Comparing R1,R2, and R3:
- R1 is greater than R3 because R1=ρLA and R3=ρL4A.
- R2 is the largest because R2=ρ4LA.
- Therefore, R3<R1<R2.
5. Conclusion:
- The resistance is minimum in Wire 3.
Final Answer:
The wire with minimum resistance is the third wire (length L2, area 2A).
- R is the resistance,
- ρ is the resistivity of the material (which is constant for copper),
- L is the length of the wire,
- A is the cross-sectional area of the wire.
- Wire 1: Length L, Area A
- Wire 2: Length 2L, Area A2
- Wire 3: Length L2, Area 2A
- Resistance of Wire 1:
R1=ρLA
- Resistance of Wire 2:
R2=ρ2LA2=ρ2L⋅2A=ρ4LA
- Resistance of Wire 3:
R3=ρL22A=ρL4A
- From the calculations:
- R1=ρLA
- R2=ρ4LA
- R3=ρL4A
- Comparing R1,R2, and R3:
- R1 is greater than R3 because R1=ρLA and R3=ρL4A.
- R2 is the largest because R2=ρ4LA.
- Therefore, R3<R1<R2.
- The resistance is minimum in Wire 3.
The wire with minimum resistance is the third wire (length L2, area 2A).
Similar Questions
One end of a Nichrome wire of length 2L and cross-sectional area A is attached to an end of another Nichrome wire of length L and cross-sectional area 2A. If the free end of the longer wire is at an electric potential of 8.0 volts, and the free end of the shorter wire is at an electric potential 1.0 volts, the potential at the junction of the two wires is equal to
View SolutionCalculate the resistance of an aluminium wire of length 50 cmand cross sectional area 2.0mm2. The resistivity of aluminium is (ρ)=2.6×10−8(Ω)m.
View Solution
Knowledge Check
A wire of length L and cross-sectional area A is made of a material of Young's modulus Y. IF the wire is stretched by an amount x, the workdone is
AYAx22LBYAx2L2CYAx2LDYAx2LA metal wire of length L1 and area of cross section A is attached to a rigid support. Another metal wire of length L2 and of the same cross sectional area is attached to the free end of the first wire. A body of mass M is then suspended from the free end of the second wire, if Y1 and Y2 are the Young's moduli of the wires respectively the effective force constant of the system of two wires is
A[(Y1Y2)A][2(Y1L2+Y2L1)]B[(Y1Y2)A](L1L2)2C[(Y1Y2)A](Y1L2+Y2L1)D[(Y1Y2)1/2A](L2+L1)1/2
The V – I graph for a wire of copper of length L and cross -section area A is shown in the figure below . The slope of the graph will be
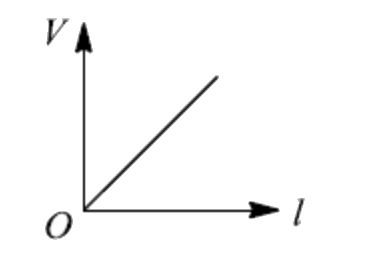
View SolutionThe V – I graph for a wire of copper of length L and cross -section area A is shown in the figure below . The slope of the graph will be

View SolutionWhat length of a copper wire of cross-sectional area 0.01mm2 will be needed to prepare a resistance of 1kΩ? Resistivity of copper =1.7×10−8Ωm.
View SolutionFind the resistance of a copper coil of total wire-length 10m and area of cross section 1.0mm^(2). What would be the resistance of a similar coil of aluminium? The resistivity of copper =1.7×10−8(Ω)m and that of aluminium2.6×10−8(Ω).
View SolutionI−V characterstic of a copper wire of length L and area fo cross-section A is shown in Fig. The slope of the curve becomes
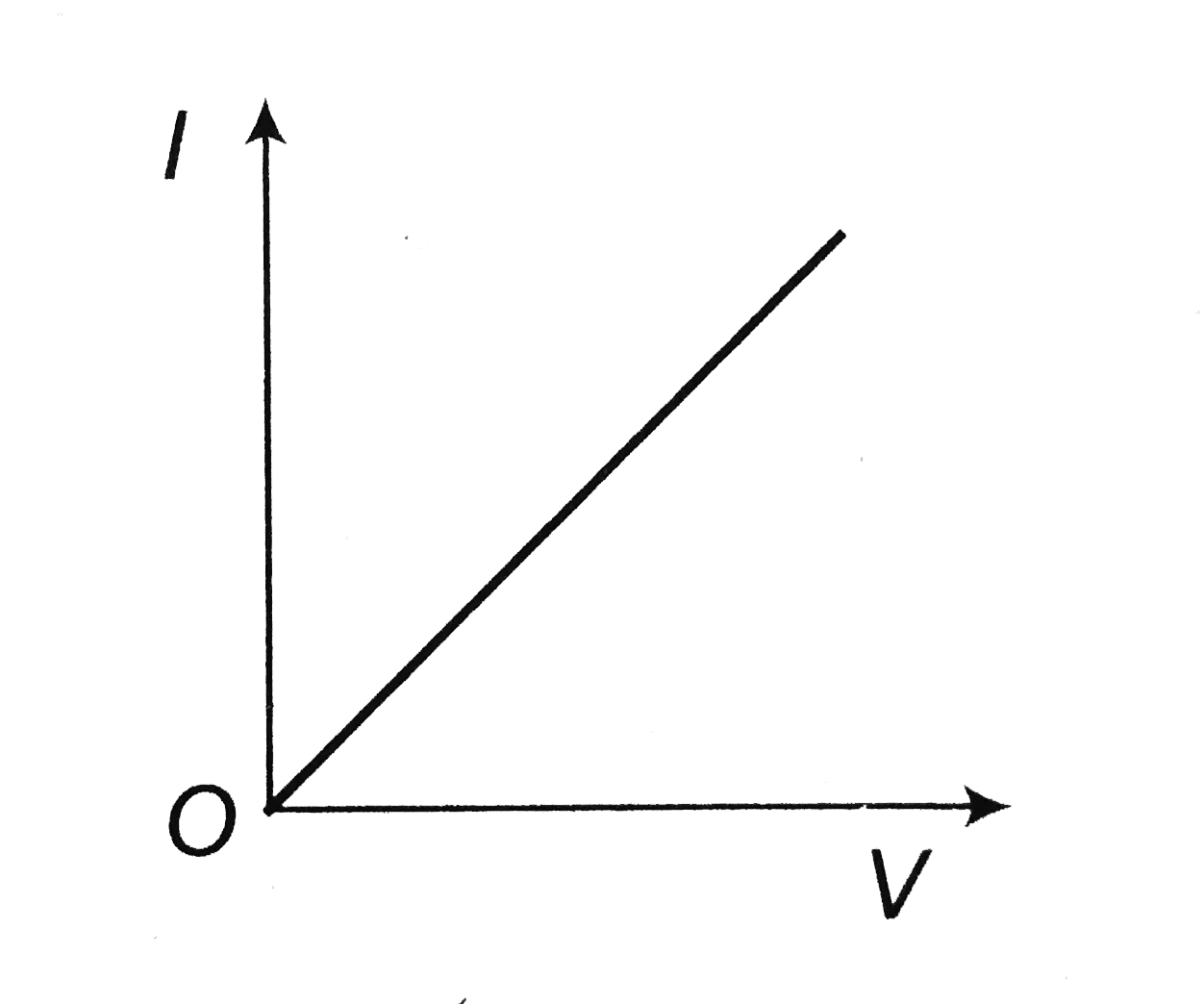
View SolutionCalculate the electric field in a copper wire of cross-sectional area2.0mm2 carrying a current of 1A .The resistivity of copper =1.7×10−8(Ω)m.
View Solution
