An electron moves in a circular orbit with a uniform speed v.It produces a magnetic field B at the centre of the circle. The radius of the circle is proportional to
Bv
vB
√vB
√Bv
The correct Answer is:c
To solve the problem, we need to analyze the relationship between the radius of the circular orbit of the electron and the magnetic field produced at the center of the circle. Here’s a step-by-step breakdown of the solution:
Step 1: Define the Current due to the Electron
The electron moving in a circular orbit can be treated as a current loop. The current I produced by the moving electron can be calculated as:
I=qT
where q is the charge of the electron (denoted as e), and T is the time period of the electron's motion.
Step 2: Calculate the Time Period T
The time period T is the time taken for the electron to complete one full circular orbit. The circumference of the circle is 2πr, and since the electron moves with a uniform speed v, we have:
T=2πrv
Step 3: Substitute T into the Current Formula
Substituting the expression for T into the current formula gives:
I=e2πrv=ev2πr
Step 4: Calculate the Magnetic Field B at the Center
The magnetic field B at the center of a circular loop carrying current I is given by the formula:
B=μ0I2r
Substituting the expression for I from Step 3 into this equation:
B=μ02r⋅ev2πr=μ0ev4πr2
Step 5: Rearranging the Equation to Find r
From the equation derived in Step 4, we can express r in terms of B:
B=μ0ev4πr2
Rearranging this gives:
r2=μ0ev4πB
Taking the square root of both sides:
r=√μ0ev4πB
Step 6: Identify the Proportional Relationship
From the equation r=√μ0ev4πB, we can see that the radius r is proportional to the square root of the ratio of v to B:
r∝√vB
Conclusion
Thus, the radius r of the circular orbit of the electron is proportional to the square root of the ratio of the speed v to the magnetic field B.
The electron moving in a circular orbit can be treated as a current loop. The current I produced by the moving electron can be calculated as:
I=qT
where q is the charge of the electron (denoted as e), and T is the time period of the electron's motion.
The time period T is the time taken for the electron to complete one full circular orbit. The circumference of the circle is 2πr, and since the electron moves with a uniform speed v, we have:
T=2πrv
Substituting the expression for T into the current formula gives:
I=e2πrv=ev2πr
The magnetic field B at the center of a circular loop carrying current I is given by the formula:
B=μ0I2r
Substituting the expression for I from Step 3 into this equation:
B=μ02r⋅ev2πr=μ0ev4πr2
From the equation derived in Step 4, we can express r in terms of B:
B=μ0ev4πr2
Rearranging this gives:
r2=μ0ev4πB
Taking the square root of both sides:
r=√μ0ev4πB
From the equation r=√μ0ev4πB, we can see that the radius r is proportional to the square root of the ratio of v to B:
r∝√vB
Thus, the radius r of the circular orbit of the electron is proportional to the square root of the ratio of the speed v to the magnetic field B.
Similar Questions
When a particle moves in a circle with a uniform speed
View SolutionAn electron moving in a circular orbit of radius R with frequency f. The magnetic field at the centre of the orbit is
View SolutionThe magnetic field at the centre of dotted circle in the arrangement shown is
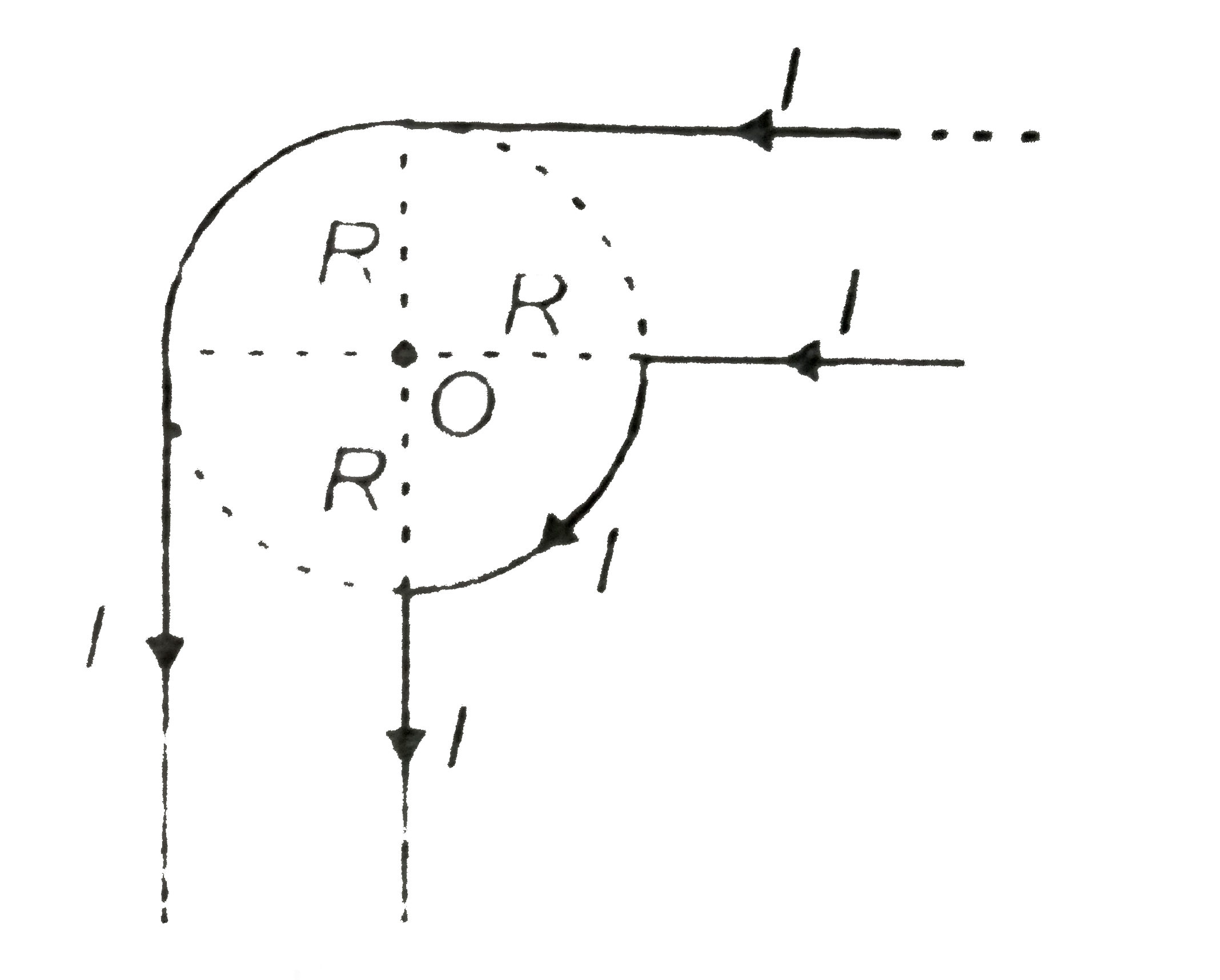
View SolutionAn electron moves in circular orbit of radius r with constant speed v.The force acting in it is
View SolutionAn electron moving in a circular orbit of radius makes n rotations per second. The magnetic field produced at the centre has magnitude :
View SolutionAn electron moving in a circular orbit of radius r makes n rotation per second. The magnetic field produced at the centre has magnitude
View SolutionAn electron is moving on a circular path of radius r with speed v in a transverse magnetic field B. e/m for it will be
View SolutionA proton of mass m and charge q enters a region of uniform magnetic field of a magnitude B with a velocity v directed perpendicular to the magnetic field. It moves in a circular path and leaves the magnetic field after completing a quarter of a circle. The time spent by the proton inside the magnetic field is proportional to:
View SolutionA particle is moving in a circle with uniform speed its motion is
View SolutionAn electron makes (3×105) revolutions per second in a circle of radius 0.5 angstrom. Find the magnetic field B at the centre of the circle.
View Solution