A proton of mass m and charge q enters a region of uniform magnetic field of a magnitude B with a velocity v directed perpendicular to the magnetic field. It moves in a circular path and leaves the magnetic field after completing a quarter of a circle. The time spent by the proton inside the magnetic field is proportional to:
(a)v−1/2
(b)v1/2
(c)v0
(d)v
The correct Answer is:C
To solve the problem, we need to analyze the motion of a proton moving in a magnetic field. Here’s a step-by-step breakdown of the solution:
Step 1: Understand the Motion of the Proton
When a charged particle like a proton enters a magnetic field perpendicularly, it experiences a magnetic force that causes it to move in a circular path. The radius of this circular path can be determined using the formula:
r=mvqB
where:
- r is the radius of the circular path,
- m is the mass of the proton,
- v is the velocity of the proton,
- q is the charge of the proton,
- B is the magnetic field strength.
Step 2: Determine the Distance Traveled
Since the proton completes a quarter of a circle, the distance d it travels while in the magnetic field is:
d=14×2πr=πr2
Step 3: Substitute for Radius
Substituting the expression for r into the distance formula gives:
d=π2(mvqB)
Step 4: Calculate the Time Spent in the Magnetic Field
The time t spent by the proton in the magnetic field can be calculated using the formula:
t=dv
Substituting the expression for d:
t=π2(mvqB)v
Step 5: Simplify the Expression
Now, simplifying the expression for t:
t=πm2qB
Step 6: Analyze Proportionality
From the final expression, we can see that the time t is proportional to m (mass of the proton), 1/q (inverse of charge), and 1/B (inverse of the magnetic field strength). Importantly, it does not depend on the velocity v.
Conclusion
Thus, we conclude that the time spent by the proton inside the magnetic field is proportional to:
t∝mqB
Since it does not depend on v, we can say that it is proportional to v0 (which equals 1).
Final Answer
The correct option is C.
---
When a charged particle like a proton enters a magnetic field perpendicularly, it experiences a magnetic force that causes it to move in a circular path. The radius of this circular path can be determined using the formula:
- r is the radius of the circular path,
- m is the mass of the proton,
- v is the velocity of the proton,
- q is the charge of the proton,
- B is the magnetic field strength.
Since the proton completes a quarter of a circle, the distance d it travels while in the magnetic field is:
Substituting the expression for r into the distance formula gives:
The time t spent by the proton in the magnetic field can be calculated using the formula:
Now, simplifying the expression for t:
From the final expression, we can see that the time t is proportional to m (mass of the proton), 1/q (inverse of charge), and 1/B (inverse of the magnetic field strength). Importantly, it does not depend on the velocity v.
Thus, we conclude that the time spent by the proton inside the magnetic field is proportional to:
The correct option is C.
Topper's Solved these Questions
- MOCK TEST 1BOOK - VMC MODULES ENGLISHCHAPTER - MOCK TEST 1EXERCISE - PART I : PHYSICS (SECTION-2)
10 Videos
- MAGNETIC EFFECTS OF CURRENT BOOK - VMC MODULES ENGLISHCHAPTER - MAGNETIC EFFECTS OF CURRENT EXERCISE - JEE Advanced (Archive)
78 Videos
- MOCK TEST 10BOOK - VMC MODULES ENGLISHCHAPTER - MOCK TEST 10EXERCISE - PHYSICS (SECTION 2)
5 Videos
Similar Questions
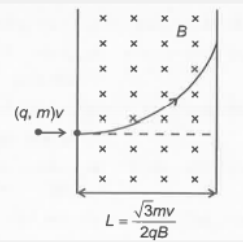
A charge particle of mass m and charge q enters in a region of uniform magnetic field as shown in figure.The magnitude of change in linear momentum due to magnetic field will be
View SolutionA proton and an electron are projected into a region of uniform magnetic field in a direction perpendicular to the field. If they have the same initial velocities then
View SolutionIf a charged particle enters perpendicular in the uniform magnetic field then
View SolutionA proton of mass m and charge q enters a magnetic field B with a velocity v at an angle θ with the direction of B.The radius of curvature of the resulting path is
View SolutionThe path of a charged particle in a uniform magnetic field, when the velocity and the magnetic field are perpendicular to each other is a
View SolutionA charged particle is moving in a circular path in uniform magnetic field. Match the following.

View SolutionA charged particle enters a uniform magnetic field perpendicular to it. The magnetic field
View SolutionA proton of mass m and charge q is moving in a plane with kinetic energy E. if there exists a uniform magnetic field B, perpendicular to the plane motion. The proton will move in a circular path of radius
View SolutionA charge q enters into a magnetic field (B) perpendicularly with velocity V. The time rate of work done by the magnetic force on the charge is
View SolutionAn electron moves in a circular orbit with a uniform speed v.It produces a magnetic field B at the centre of the circle. The radius of the circle is proportional to
View Solution
