Current is flowing in a coil of area A and number of turns N, then magnetic moment of the coil ,M is equal to
NiA
NiA
Ni√A
N2Ai
The correct Answer is:a
To find the magnetic moment M of a coil with area A and number of turns N carrying a current I, we can follow these steps:
1. Understanding Magnetic Moment: The magnetic moment M of a coil is a measure of the strength and orientation of its magnetic field. It depends on the number of turns in the coil, the current flowing through it, and the area of the coil.
2. Formula for Magnetic Moment: The magnetic moment M can be expressed with the formula:
M=N⋅I⋅A
where:
- N is the number of turns in the coil,
- I is the current flowing through the coil,
- A is the area of the coil.
3. Substituting Values: If we have the values for N, I, and A, we can substitute them into the formula to calculate the magnetic moment.
4. Final Expression: Thus, the magnetic moment M of the coil can be expressed as:
M=N⋅I⋅A
Conclusion:
The magnetic moment M of a coil with area A and number of turns N carrying a current I is given by the formula:
M=N⋅I⋅A
M=N⋅I⋅A
where:
- N is the number of turns in the coil,
- I is the current flowing through the coil,
- A is the area of the coil.
M=N⋅I⋅A
The magnetic moment M of a coil with area A and number of turns N carrying a current I is given by the formula:
M=N⋅I⋅A
Similar Questions
Current 'i' is flowing in heaxagonal coil of side a. The magnetic induction at the centre of the coil will be
View SolutionA moving coil galvanometer coil has a coil of area A, number of turns N. The radial magnetic field present is B. the moment of inertia of the coil is I about its rotation axis. The torque is applied by the magnetic field on the coil of the galvanometer when current I0 passes through it and produces a deflection of π/2 of the pointer. Then answer the following questions based on the paragraph.
Then the value of torsionl constant of the spring is
View Solution
Knowledge Check
A 200 turn closely wound circular coil of radius 15 cm carries a current of 4 A. The magnetic moment of this coil is
A36.5Am2B56.5Am2C66.5Am2D108Am2
A square 12-turn coil with sides of length 40 cm carries a current of 3 A. It lies in the x-y plane as shown in Fig. in a uniform magnetic field.
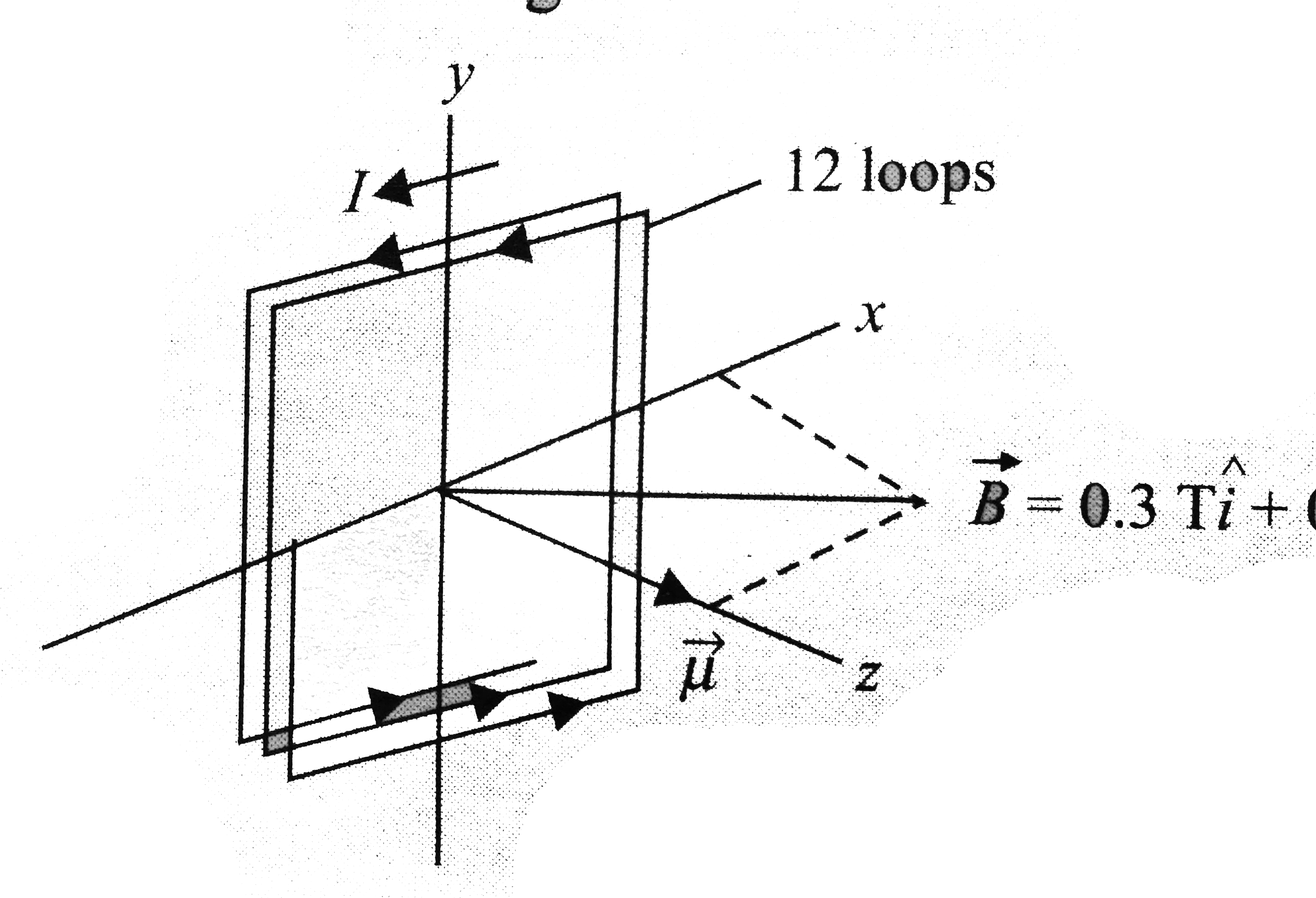
(a) Find the magnetic moment of the coil.
(b) Find the torque exerted on the coil.
(c) Find the potential energy of the coil.
View SolutionCurrent I A flowing in a circular coil of radius r. then the magnetic induction at the centre is B. If the current is doubled then the magnetic induction will be
View SolutionMagnetic field due to 0.1A current flowing through a circular coil of radius 0.1m and 1000 turns at the centre of the coil is
View SolutionA long wire carrying a steady current is bent into a circular loop of one turn. The magnetic field at the centre of the loop is B. it is then bent into a circular coil of n turns. The magnetic field at the centre of this coil of n turns will be
View SolutionA circular coil of N turns and radius R carries a current I. It is unwound and rewound to make another coil of radius R/2. Current I remaining the same. Calculate the ratio of the magnetic moments of the new coil and the original coil.
View SolutionIn a moving coil galvanometer, torque on the coil can be experessed as τ=ki, where i is current through the wire and k is constant . The rectangular coil of the galvanometer having number of turns N , area A and moment of interia I is placed in magnetic field B. Find
(a) k in terms of given parameters N,I,AandB
(b) the torsion constant of the spring , if a current i0 produces a deflection of (π)/(2) in the coil .
(c) the maximum angle through which the coil is deflected, if charge Q is passed through the coil almost instaneously. ( ignore the daming in mechinal oscillations).
View SolutionIn a moving coil galvanometer, torque on the coil can be experessed as τ=ki, where i is current through the wire and k is constant . The rectangular coil of the galvanometer having number of turns N , area A and moment of interia I is placed in magnetic field B. Find
(a) k in terms of given parameters N,I,AandB
(b) the torsion constant of the spring , if a current i0 produces a deflection of (π)/(2) in the coil .
(c) the maximum angle through which the coil is deflected, if charge Q is passed through the coil almost instaneously. ( ignore the daming in mechinal oscillations).
View Solution
