For a series L C R circuit, the power loss at resonance is
V2ωL−1ωC
i2Cω
i2R
V2ωC
The correct Answer is:C
To find the power loss at resonance in a series LCR circuit, we can follow these steps:
Step 1: Understand the Impedance at Resonance
In a series LCR circuit, the total impedance (Z) is given by:
Z=√R2+(XL−XC)2
Where:
- R is the resistance,
- XL is the inductive reactance,
- XC is the capacitive reactance.
At resonance, the inductive reactance equals the capacitive reactance:
XL=XC
Thus, at resonance:
Z=R
Step 2: Determine the Current at Resonance
The current (I) in the circuit can be calculated using Ohm's law:
I=VZ
Substituting the impedance at resonance:
I=VR
Where V is the supply voltage.
Step 3: Calculate the Power Loss
The power (P) in an AC circuit is given by:
P=V⋅I⋅cos(θ)
At resonance, the current and voltage are in phase, which means the phase angle θ=0 and cos(0)=1. Thus, the power simplifies to:
P=V⋅I
Substituting the expression for current:
P=V⋅(VR)=V2R
Step 4: Express Power in Terms of Current
We can also express power in terms of current:
P=I2R
Using the current we found earlier:
P=(VR)2R=V2R
Conclusion
Thus, the power loss at resonance in a series LCR circuit is:
P=V2R
In a series LCR circuit, the total impedance (Z) is given by:
Z=√R2+(XL−XC)2
Where:
- R is the resistance,
- XL is the inductive reactance,
- XC is the capacitive reactance.
XL=XC
Thus, at resonance:
Z=R
The current (I) in the circuit can be calculated using Ohm's law:
I=VZ
Substituting the impedance at resonance:
I=VR
Where V is the supply voltage.
The power (P) in an AC circuit is given by:
P=V⋅I⋅cos(θ)
At resonance, the current and voltage are in phase, which means the phase angle θ=0 and cos(0)=1. Thus, the power simplifies to:
P=V⋅I
Substituting the expression for current:
P=V⋅(VR)=V2R
We can also express power in terms of current:
P=I2R
Using the current we found earlier:
P=(VR)2R=V2R
Thus, the power loss at resonance in a series LCR circuit is:
P=V2R
Similar Questions
A series L-C-R circuit is operated at resonance . Then
View SolutionFor a series L - C - R circuit at resonance, the statement which is not true is
View SolutionIn series LCR circuit at resonance
View SolutionIn L−C−R series AC circuit,
View SolutionAssertion: At resonance, power factor of series L-C-R circuit is zero.
Reason: At resonance, current function and voltage functions are in same phase.
View SolutionIf V0 and I0 are the peak current and voltge across the resistor in a series L−C−R circuit, then the power dissipated in the circuit is (powerfac→r=cosθ)
View SolutionThe value of power factor coso in series LCR circuit at resonance is
View SolutionIn a series LCR circuit, at resonance, power factor is …….. .
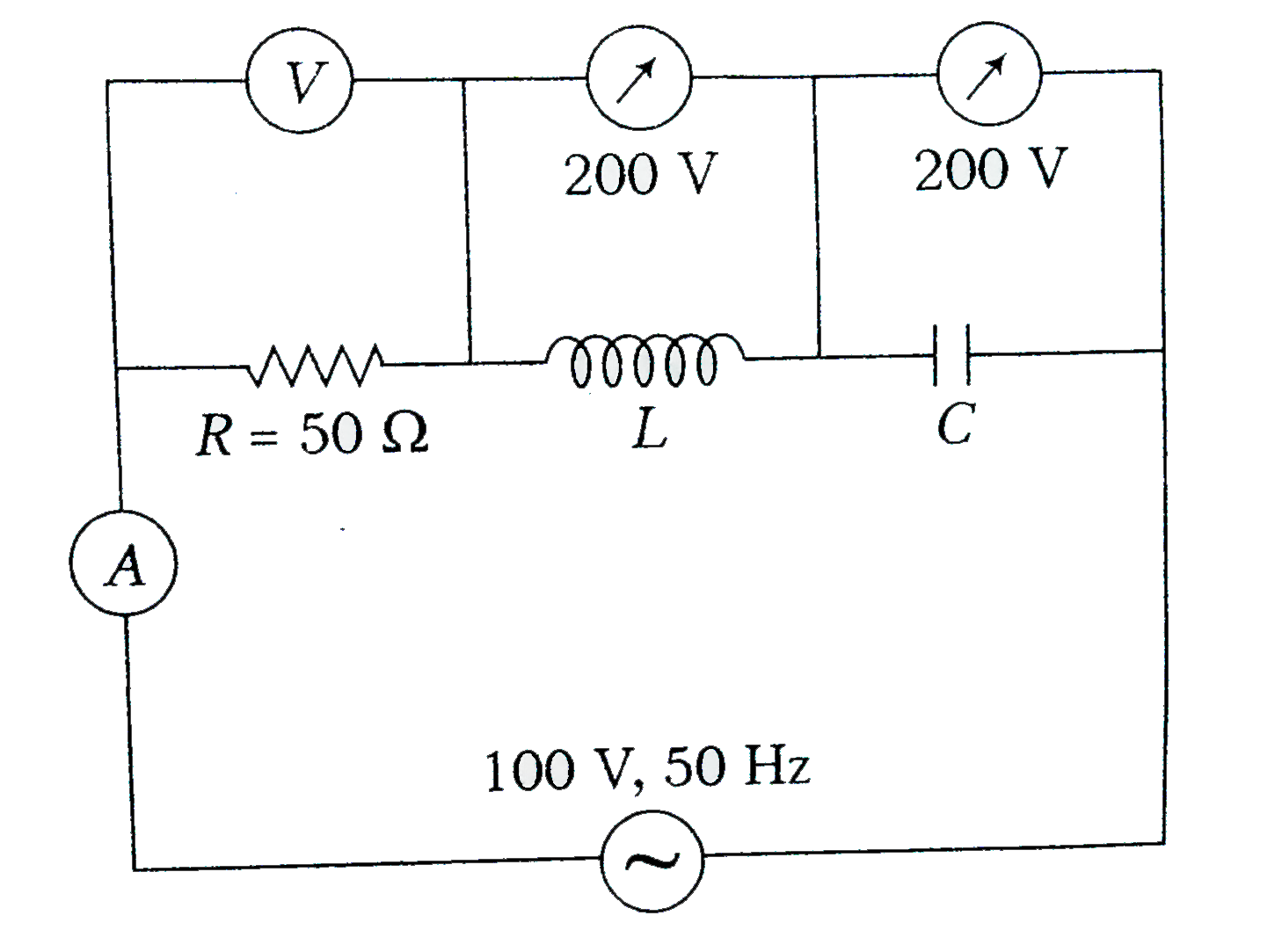
View SolutionIn the series L-C-R circuit , the voltmeter and ammeter readings are respectively
View SolutionIn the series L-C-R circuit , the voltmeter and ammeter readings are
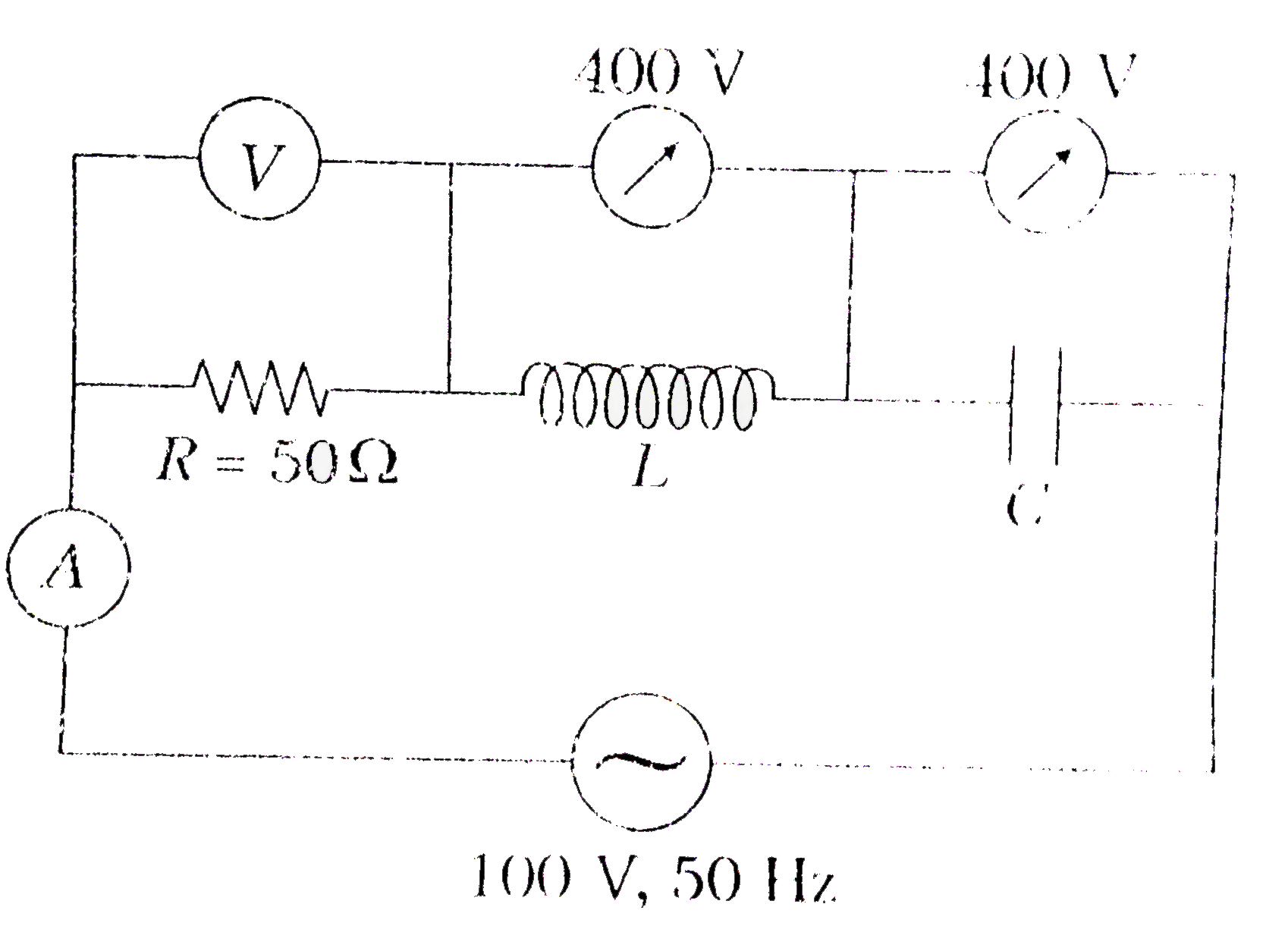
View Solution
