The count rate of a Geiger Muller counter for the radiation of a radioactive material of half-life 30 min decreases to 5s−1 after 2h. The initial count rate was
20s−1
25s−1
80s−1
625s−1
The correct Answer is:C
To solve the problem, we need to determine the initial count rate of a Geiger Muller counter for a radioactive material, given its half-life and the count rate after a certain time period.
1. Identify Given Values:
- Half-life of the radioactive material, t1/2=30 minutes.
- Time elapsed, t=2 hours = 120 minutes.
- Count rate after 2 hours, N=5 counts per second.
2. Calculate Number of Half-Lives:
- The number of half-lives n can be calculated using the formula:
n=tt1/2
- Substituting the values:
n=120 min30 min=4
3. Use the Radioactive Decay Law:
- The relationship between the initial count rate N0 and the count rate after n half-lives N is given by:
N=N0(12)n
- Rearranging this gives:
N0=N⋅2n
4. Substituting Known Values:
- Now substitute N=5 counts per second and n=4:
N0=5⋅24
- Calculate 24=16:
N0=5⋅16=80
5. Final Result:
- The initial count rate N0 is 80 counts per second.
Conclusion:
The initial count rate was 80 counts per second.
---
- Half-life of the radioactive material, t1/2=30 minutes.
- Time elapsed, t=2 hours = 120 minutes.
- Count rate after 2 hours, N=5 counts per second.
- The number of half-lives n can be calculated using the formula:
n=tt1/2
- Substituting the values:
n=120 min30 min=4
- The relationship between the initial count rate N0 and the count rate after n half-lives N is given by:
N=N0(12)n
- Rearranging this gives:
N0=N⋅2n
- Now substitute N=5 counts per second and n=4:
N0=5⋅24
- Calculate 24=16:
N0=5⋅16=80
- The initial count rate N0 is 80 counts per second.
The initial count rate was 80 counts per second.
Similar Questions
Knowledge Check
Suppose we consider a large number of containers each containing initially 10000 atoms of a radioactive material with a half life of 1 yr. After 1 yr
Aall the containers will have 5000 atoms of the material.Ball the containers will contain the same number of atoms of the material but that number will only be approximately 5000Cthe containers will in general have different number of the atoms of the material but their average will be close to 5000Dnone of containers can have more than 5000 atoms.
Find the half-life period of a radioactive material if its activity drops of (116)th of its initial value in 40 years.
View SolutionAfter an interval of one day , 1/16th initial amount of a radioactive material remains in a sample. Then, its half-life is .
View SolutionIf T is the half-life of a radioactive material, then the fraction that would remain after a time T2 is
View SolutionHalf−life of a radioactive disintegration (A→B) having rate constat 231s−1 is
View SolutionSuppose we consider a large number of continers each containing initially 10000 atoms of a radioactive material with a half life of 1 year. After 1 year.
View SolutionSuppose we consider a large number of continers each containing initially 10000 atoms of a radioactive material with a half life of 1 year. After 1 year.
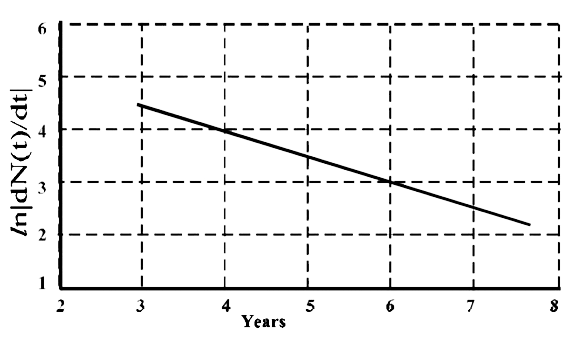
View SolutionTo determine the half life of a radioactive element , a student plot a graph of in ∣∣∣dN(t)dt∣∣∣ versus t , Here ∣∣∣dN(t)dt∣∣∣ is the rate of radiation decay at time t , if the number of radioactive nuclei of this element decreases by a factor of p after 4.16year the value of p is
View Solution
