A particle is thrown vertically upward. Its velocity at half of the height is 10 m/s. Then the maximum height attained by it : -
(g=10m/s2)
8 m
20 m
10 m
16 m
The correct Answer is:C
To solve the problem step by step, we will use the kinematic equations of motion.
Step 1: Understand the problem
A particle is thrown vertically upward, and we know its velocity at half of the maximum height is 10 m/s. We need to find the maximum height attained by the particle.
Step 2: Define the variables
- Let the maximum height be H.
- Therefore, the height at half of the maximum height is H2.
- The velocity at half the height is given as v=10m/s.
- The acceleration due to gravity is g=10m/s2.
Step 3: Apply the kinematic equation
We can use the kinematic equation:
v2=u2+2as
Where:
- v is the final velocity (0 m/s at the maximum height),
- u is the initial velocity (10 m/s at half the height),
- a is the acceleration (which is -g, or -10 m/s², since gravity acts downward),
- s is the displacement (which is H2).
Step 4: Substitute the known values
Substituting the known values into the equation:
0=(10)2+2(−10)(H2)
This simplifies to:
0=100−10H
Step 5: Solve for H
Rearranging the equation gives:
10H=100
H=10010=10m
Conclusion
The maximum height attained by the particle is H=10m.
A particle is thrown vertically upward, and we know its velocity at half of the maximum height is 10 m/s. We need to find the maximum height attained by the particle.
- Let the maximum height be H.
- Therefore, the height at half of the maximum height is H2.
- The velocity at half the height is given as v=10m/s.
- The acceleration due to gravity is g=10m/s2.
We can use the kinematic equation:
v2=u2+2as
Where:
- v is the final velocity (0 m/s at the maximum height),
- u is the initial velocity (10 m/s at half the height),
- a is the acceleration (which is -g, or -10 m/s², since gravity acts downward),
- s is the displacement (which is H2).
Substituting the known values into the equation:
0=(10)2+2(−10)(H2)
This simplifies to:
0=100−10H
Rearranging the equation gives:
10H=100
H=10010=10m
The maximum height attained by the particle is H=10m.
Similar Questions
A particle is thrown vertically upwards. If its velocity is half of the maximum height is 20m/s, then maximum height attained by it is
View SolutionWhen a particle is thrown vertically upwards, its velocity at one third of its maximum height is 10√2m/s. The maximum height attained by it is
View SolutionA ball is thrown vertically upward with a velocity of 20 m/s. Calculate the maximum height attain by the ball.
View SolutionA stone is thrown vertically upwards. When stone is at a height half of its maximum height, its speed is 10ms−1 , then the maximum height attained by the stone is (g=10ms−2)
View SolutionA stone is thrown vertically upwards. When stone is at a height half of its maximum height, its speed is 10ms−1 , then the maximum height attained by the stone is (g=10ms−2)
View SolutionA body is thrown vertically upwards with an initial velocity u. Write an expression for the maximum height attained by the body.
View SolutionThe time of flight of a projectile is 10 s and range is 500m. Maximum height attained by it is [g=10m/s2]
View SolutionThe time of flight of a projectile is 10 s and range is 500m. Maximum height attained by it is [g=10m/s2]
View SolutionTwo bodies are thrown vertically upwards with their initial velocity in the ratio 2:3. Then the ratio of the maximum height attained by them is
View SolutionA rocket is projected vertically upwards and its time velocity graph is shown in the figure-1.115. The maximum height attained by the rocket is :
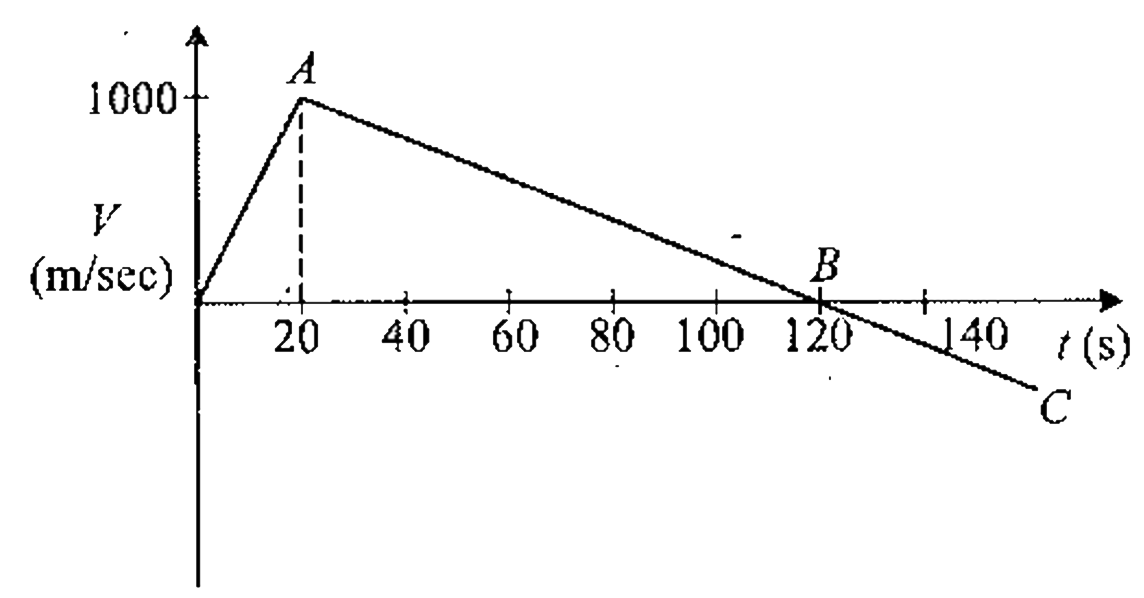
View Solution
