A ray of light is incident on a 60∘ prism at the minimum deviation position. The angle of refraction at the first face (i.e. incident face) of the prism is-
30∘
45∘
60∘
Zero
The correct Answer is:A
To solve the problem, we need to find the angle of refraction at the first face of a prism when light is incident at the minimum deviation position. Here's the step-by-step solution:
Step 1: Understand the Prism and Minimum Deviation
We are given a prism with an angle A=60∘. At the minimum deviation position, the light ray experiences equal angles of refraction at both faces of the prism.
Step 2: Use the Relation for Minimum Deviation
At minimum deviation, the angles of refraction r1 and r2 at the two faces of the prism are equal. Therefore, we can denote both angles as r.
Step 3: Apply the Formula for Prism
The relation for the angles in a prism at minimum deviation is given by:
A=r1+r2
Since r1=r2=r, we can rewrite this as:
A=2r
Step 4: Substitute the Given Angle
Substituting the value of the angle of the prism A:
60∘=2r
Step 5: Solve for r
Now, we can solve for r:
r=60∘2=30∘
Conclusion
Thus, the angle of refraction at the first face of the prism is 30∘.
Final Answer
The angle of refraction at the first face of the prism is 30∘.
---
We are given a prism with an angle A=60∘. At the minimum deviation position, the light ray experiences equal angles of refraction at both faces of the prism.
At minimum deviation, the angles of refraction r1 and r2 at the two faces of the prism are equal. Therefore, we can denote both angles as r.
The relation for the angles in a prism at minimum deviation is given by:
A=r1+r2
Since r1=r2=r, we can rewrite this as:
A=2r
Substituting the value of the angle of the prism A:
60∘=2r
Now, we can solve for r:
r=60∘2=30∘
Thus, the angle of refraction at the first face of the prism is 30∘.
The angle of refraction at the first face of the prism is 30∘.
Similar Questions
When light of wavelength λ is incident on an equilateral prism, kept on its minimum deviation position, it is found that the angle of deviation equals the angle of the prism itself. The refractive index of the material of the prism for the wavelength λ is
View SolutionA ray passes through a prism of angle 60∘ in minimum deviation position and suffers a deviation of 30∘. What is the angle of incidence on the prism
View SolutionA light ray of angles of incidence 40∘ emerged from the prism in minimum deviation position. Then what is the angle of incidence on the second surface ?
View SolutionA light ray is incident upon a prism in minimum deviation position and suffers a deviation of 34∘. If the shaded half of the prism is knocked off, the ray will
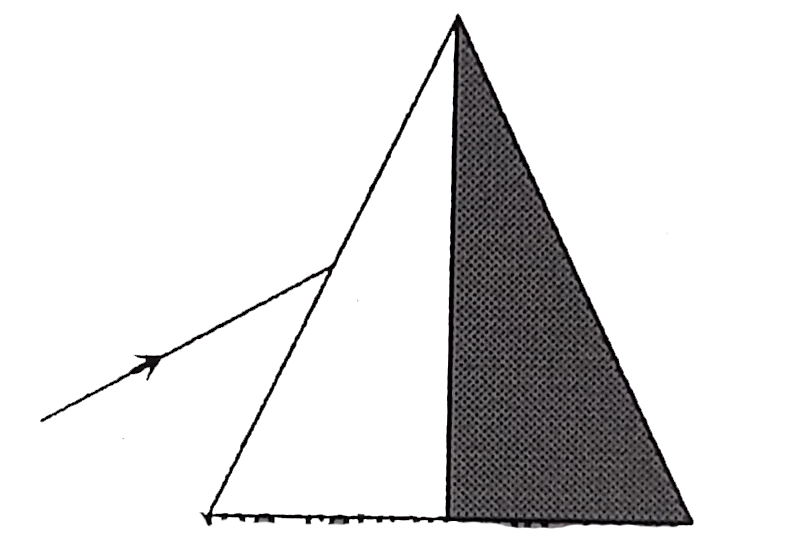
View Solution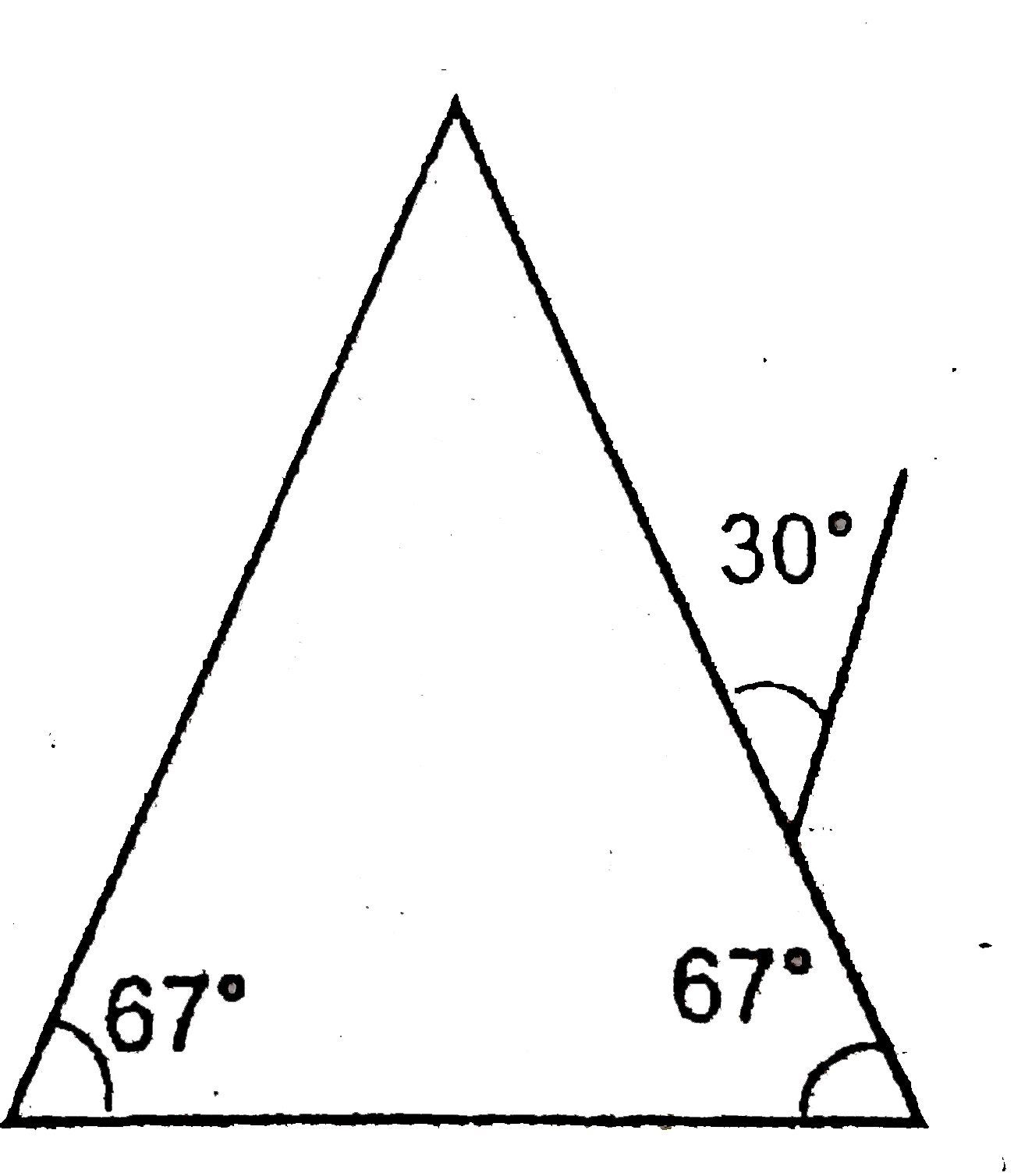
A ray of light incident on one of the face of a prism as shown. After refraction through this surface ray is incident on base of the prism. The refractive index of the prism is √3. The net deviation of the ray due to prism is
View SolutionIf the refracting angle of a prism is 60∘ and the minimum deviation is 30∘, then the angle of incidence is
View SolutionA ray of light is normally incident on one face of an equilateral glass prism. Answer the following :
(a) What is the angle of incidence on the first face of the prism?
(b) What is the angle of refraction from the first face of the prism ?
(c) What will be the angle of incidence at the second face of the prism ?
(d) Will the light ray suffer minimum deviation by the prism ?
View SolutionThe angle of incidence for a ray of light at a refracting surface of a prism is 45∘. The angle of prism is 60∘. If the ray suffers minimum deviation through the prism, the angle of minimum deviation and refractive index of the material of the prism respectively, are :
View SolutionA prism is made of glass of unknown refractive index. A parallel beam of light is incident on a face of the prism. By rotating the prism, the minimum angle of deviation is measured to be 40∘. What is the refractive index of the prism ? If the prism is placed in water (μ=1.33), predict the new angle of minimum deviation of the parallel beam. The refracting angle of prism is 60∘.
View SolutionA ray of light is incident on a prism ABC of μ=√3 as shown in Fig. Find the angle of incidence for which the deviation of light by the prism ABC is minimum.
By what angle should the second prism be rotated so that final ray suffers net minimum deviation ?
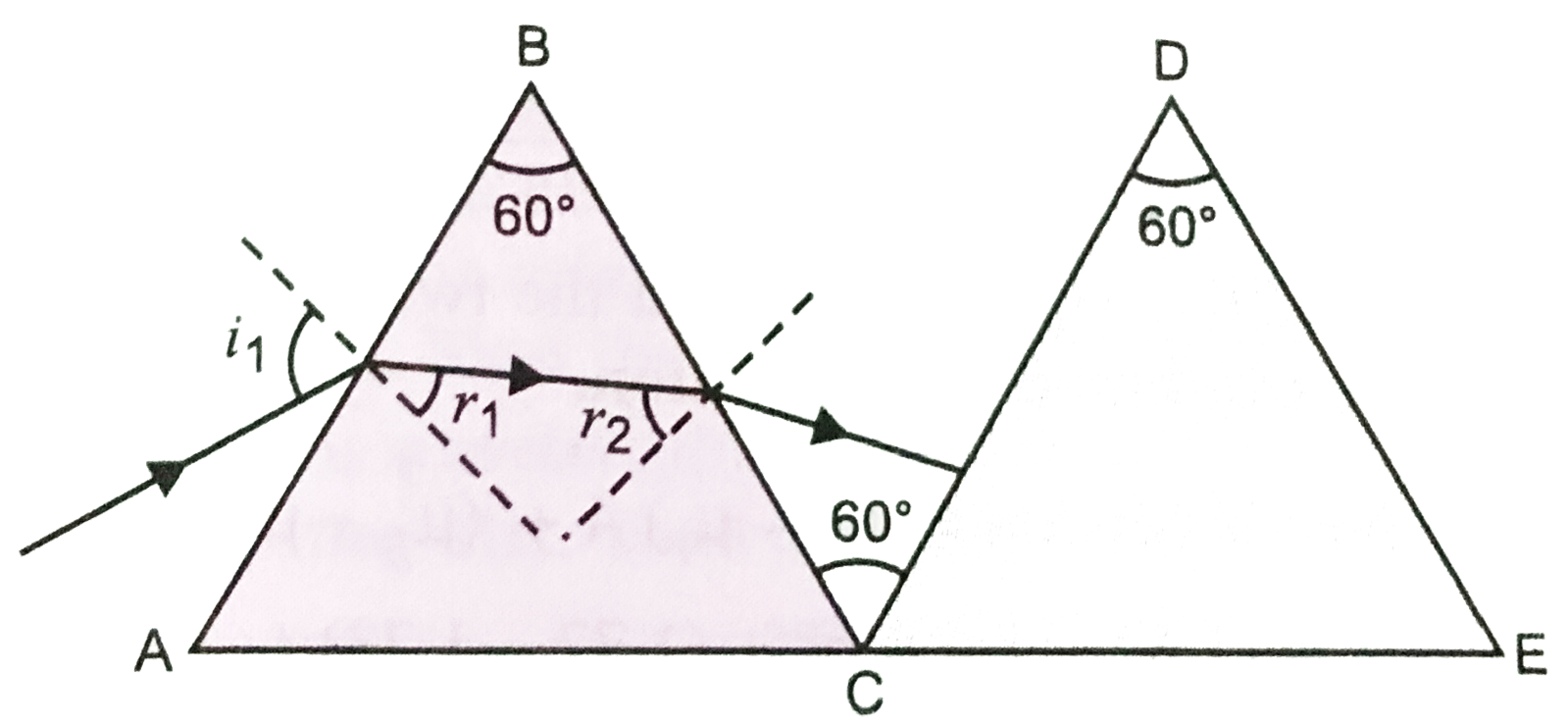
View Solution
