When three identical bulbs are connected in series. The consumed power is 10W. If they are now connected in pa rallel then the consumed power will be:-
30W
29W
10/3W
270W
The correct Answer is:B
To solve the problem, we need to determine the power consumed by three identical bulbs when they are connected in parallel after knowing the power consumed when they are connected in series.
1. Understanding the Series Connection:
- When the three identical bulbs are connected in series, the total resistance Rtotal is the sum of the individual resistances. If each bulb has a resistance R, then:
Rtotal=R+R+R=3R
- The power consumed in the series connection is given as P=10W.
2. Using the Power Formula:
- The power consumed in a circuit can be calculated using the formula:
P=V2Rtotal
- Substituting the total resistance for the series connection:
10=V23R
- Rearranging this gives us:
V2=30R(1)
3. Understanding the Parallel Connection:
- When the bulbs are connected in parallel, the total resistance R′ is given by:
1R′=1R+1R+1R=3R⟹R′=R3
4. Calculating Power in Parallel Connection:
- The power consumed in the parallel connection can be calculated using the same power formula:
P′=V2R′
- Substituting R′:
P′=V2R3=3⋅V2R
- From equation (1), we know that V2R=30:
P′=3⋅30=90W
5. Conclusion:
- The power consumed when the three identical bulbs are connected in parallel is 90W.
Final Answer:
The consumed power when the bulbs are connected in parallel is 90 W.
- When the three identical bulbs are connected in series, the total resistance Rtotal is the sum of the individual resistances. If each bulb has a resistance R, then:
Rtotal=R+R+R=3R
- The power consumed in the series connection is given as P=10W.
- The power consumed in a circuit can be calculated using the formula:
P=V2Rtotal
- Substituting the total resistance for the series connection:
10=V23R
- Rearranging this gives us:
V2=30R(1)
- When the bulbs are connected in parallel, the total resistance R′ is given by:
1R′=1R+1R+1R=3R⟹R′=R3
- The power consumed in the parallel connection can be calculated using the same power formula:
P′=V2R′
- Substituting R′:
P′=V2R3=3⋅V2R
- From equation (1), we know that V2R=30:
P′=3⋅30=90W
- The power consumed when the three identical bulbs are connected in parallel is 90W.
The consumed power when the bulbs are connected in parallel is 90 W.
Similar Questions
When two resistances R1andR2 are connected in series , they consume 12 W powers . When they are connected in parallel , they consume 50 W powers . What is the ratio of the powers of R1andR2 ?
View Solution3 identical bulbs are connected in series and these together dissipate a power P . If now the bulbs are connected in parallel, then the power dissipated will be
View SolutionTwo equal resistances when connected in series to a battery ,consume electric power of 60 W .If these resitances are now connected in parealled combination to the same battery ,the electric power cansumed will be :
View SolutionWhen three identical bulbs of 60W,200V rating are connected in series to a 200V supply, the power drawn by them will be
View SolutionThree equal resistances connected is series across a source of e.m.f consume 20 watt. If the same resistor are connected in parallel across the same source of e.m.f., what would be the power dissipated ?
View SolutionThree identical bulb are connected as shown in figure . When switch S is closed, the power consumed in bulb B is P . What will be the power consumed by the same bulb when switch S is opened ?
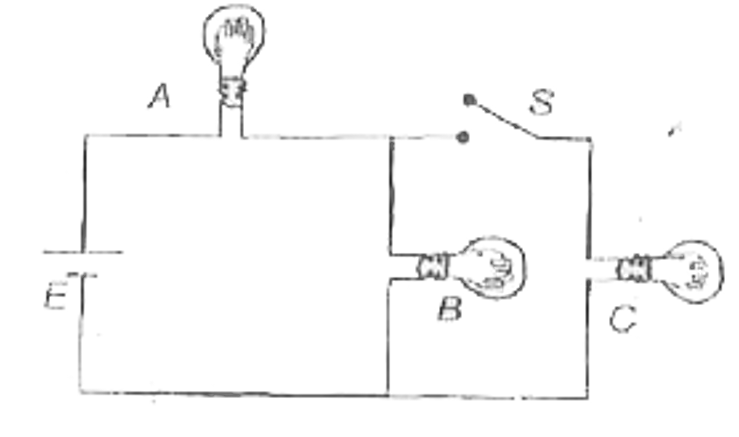
View SolutionThree identical resistors are connected in series . When a certain potential difference is applied across the combination , the total power would be dissipated is 27W. How many times the power would be dissipated if the three resistors were connected in parallel across the same potential difference ?
View SolutionTen 60 W, 220 V bulbs are connected in series to 220 V supply . Power consumed in the circuit is
View SolutionAssertion : Two identical bulbs when connected across a battery, produce a total power P. When they are connected across the same battery in series total power consumed will be P4.
Reason : In parallel, P=P1+P2 and in series P=P1P2P1+P2
View SolutionTwo bulbs when connected in parallel to a source take 60 W each, the power consumed, when they are connected in series with the same source is
View Solution