In a regular hexagon ABCDEF, −−→AE
To find the vector →AE in a regular hexagon ABCDEF, we can follow these steps:
Step 1: Draw the Regular Hexagon
First, we need to visualize the regular hexagon ABCDEF. A regular hexagon has all sides equal and can be inscribed in a circle. The vertices are equidistant from the center.
Step 2: Identify the Vectors
We need to express the vector →AE in terms of other vectors. We can move from point A to point E by traversing through the other vertices.
Step 3: Break Down the Path
We can express →AE as:
→AE=→AB+→BC+→CD+→DE
This means we can reach point E starting from point A by moving to B, then to C, then to D, and finally to E.
Step 4: Combine Vectors
Next, we can combine some of these vectors. Notice that:
→AB+→BC=→AC
Thus, we can rewrite →AE as:
→AE=→AC+→CD+→DE
Step 5: Recognize Parallel Vectors
In a regular hexagon, opposite sides are equal and parallel. Therefore, we have:
→CD=→AF
This allows us to express →AE as:
→AE=→AC+→AF+→DE
Step 6: Express →DE
Notice that the vector →DE can be expressed in terms of →AB because →DE is in the opposite direction of →AB:
→DE=−→AB
Step 7: Final Expression
Now we can substitute →DE back into our equation:
→AE=→AC+→AF−→AB
Conclusion
Thus, the vector →AE can be expressed as:
→AE=→AC+→AF−→AB
First, we need to visualize the regular hexagon ABCDEF. A regular hexagon has all sides equal and can be inscribed in a circle. The vertices are equidistant from the center.
We need to express the vector →AE in terms of other vectors. We can move from point A to point E by traversing through the other vertices.
We can express →AE as:
→AE=→AB+→BC+→CD+→DE
This means we can reach point E starting from point A by moving to B, then to C, then to D, and finally to E.
Next, we can combine some of these vectors. Notice that:
→AB+→BC=→AC
Thus, we can rewrite →AE as:
→AE=→AC+→CD+→DE
In a regular hexagon, opposite sides are equal and parallel. Therefore, we have:
→CD=→AF
This allows us to express →AE as:
→AE=→AC+→AF+→DE
Notice that the vector →DE can be expressed in terms of →AB because →DE is in the opposite direction of →AB:
→DE=−→AB
Now we can substitute →DE back into our equation:
→AE=→AC+→AF−→AB
Thus, the vector →AE can be expressed as:
→AE=→AC+→AF−→AB
Topper's Solved these Questions
- VECTOR ALGEBRABOOK - ARIHANT MATHS ENGLISHCHAPTER - VECTOR ALGEBRAEXERCISE - Exercise For Session 3
11 Videos
- VECTOR ALGEBRABOOK - ARIHANT MATHS ENGLISHCHAPTER - VECTOR ALGEBRAEXERCISE - VECTOR ALGEBRA EXERCISES 1: Single Option Correct Type Questions
1 Videos
- VECTOR ALGEBRABOOK - ARIHANT MATHS ENGLISHCHAPTER - VECTOR ALGEBRAEXERCISE - Exercise For Session 1
7 Videos
- TRIGONOMETRIC FUNCTIONS AND IDENTITIESBOOK - ARIHANT MATHS ENGLISHCHAPTER - TRIGONOMETRIC FUNCTIONS AND IDENTITIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
19 Videos
Similar Questions
In a regular hexagon ABCDEF,¯¯¯¯¯¯AB+¯¯¯¯¯¯AC+¯¯¯¯¯¯AD+¯¯¯¯¯¯AE+¯¯¯¯¯¯AF=k¯¯¯¯¯¯AD then k is equal to
View SolutionIn a regular hexagon ABCDEF, prove that −−→AB+−−→AC+−−→AD+−−→AE+−−→AF=3−−→AD
View SolutionIn a regular hexagon ABCDEF, AB||ED|| x-axis. What are the slopes of its sides?
View SolutionIn a regular hexagon ABCDEF, A→B=a, B→C=→b and →CD=c Then\ →AE=
a.→a+→b+→c b. 2→a+→b+→c c. →b+→c d. →a+2→b+2→c
View SolutionThe coordinates of two consecutive vertices A and B of a regular hexagon ABCDEF are (1,0) and (2,0) respectively. The equation of the diagonal CE is:
View Solutiona and b form the consecutive sides of a regular hexagon ABCDEF.
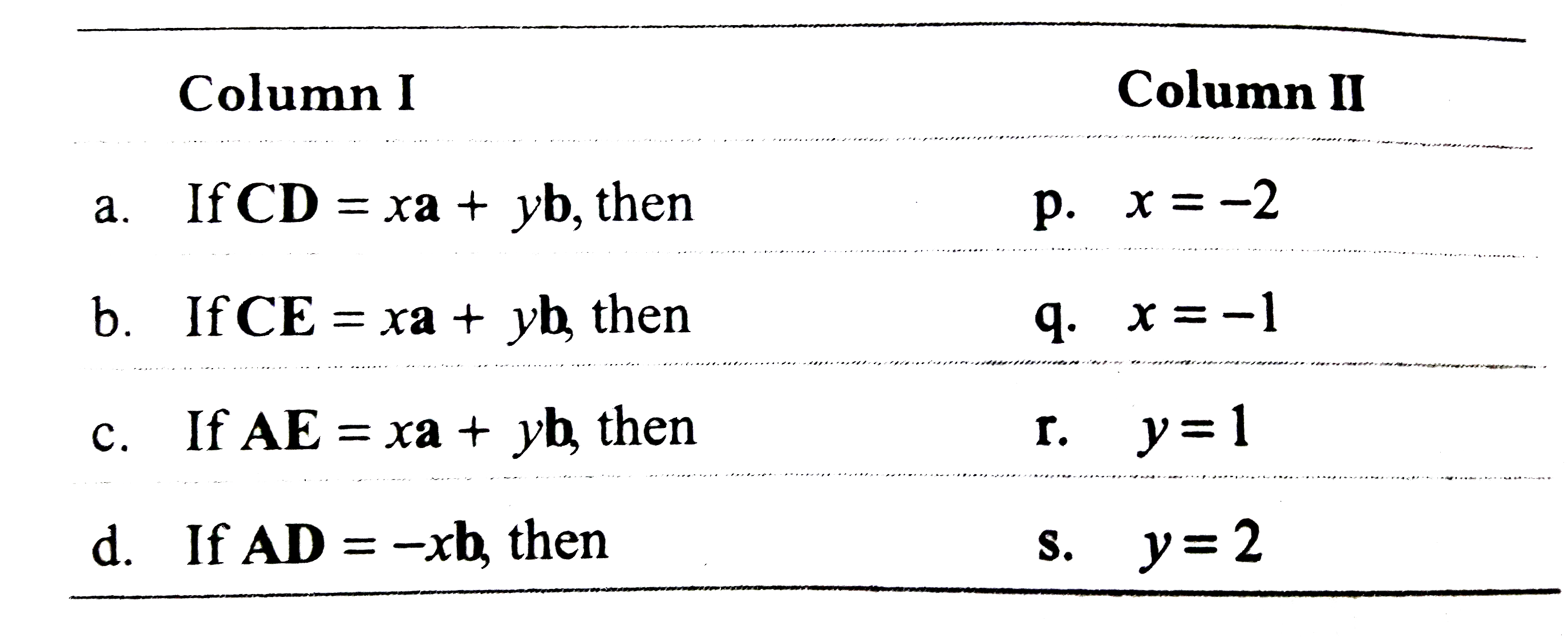
View SolutionIf →aand→b are the vectors determined by two adjacent sides of a regular hexagon ABCDEF, find the vector determined by the ther sides taken in order. Also find −−→ADand−−→CE in terms of →aand→b.
View SolutionA particle moves with constant speed v along a regular hexagon ABCDEF in the same order. Then the magnitude of the avergae velocity for its motion form A to
View SolutionIf →a, →b are the vectors forming consecutive sides of a regular of a regular hexagon ABCDEF, then the vecrtor representing side CD is
a.→a+→b b. →a−→b c. →b−→a d. −(→a+→b)
View SolutionLet O be the centre of the regular hexagon ABCDEF then find −−→OA+−−→OB+−−→OD+−−→OC+−−→OE+−−→OF
View Solution
