Consider the lines L1:r=a+λbandL2:r=b+μa, where a and b are non zero and non collinear vectors.
Statement-I L1andL2 are coplanar and the plane containing these lines passes through origin.
Statement-II (a−b)⋅(a×b)=0 and the plane containing L1andL2 is [r a b]=0 which passe through origin.
Statement-I is true, Statement II is also true, Statement-II is the correct explanation of Statement-I.
Statement-I is true, Statement-II is also true, Statement-II is not the correct explanation of Statement-I.
Statement-I is true, Statement-II is false.
Statement-I is false, Statement -II is true.
The correct Answer is:(a)
To solve the problem, we need to analyze the given lines L1 and L2 and the statements provided.
Step 1: Understand the lines
The lines are given as:
- L1:r=a+λb
- L2:r=b+μa
Here, a and b are non-zero and non-collinear vectors. This means they do not lie on the same line.
Hint: Identify the direction vectors of the lines and the points they pass through.
Step 2: Check for coplanarity
To check if the lines L1 and L2 are coplanar, we can use the condition involving the scalar triple product. The lines are coplanar if:
(a−b)⋅(a×b)=0
Hint: Recall that the scalar triple product gives a volume; if it equals zero, the vectors are coplanar.
Step 3: Analyze the plane containing the lines
The normal vector to the plane containing the lines can be found using the cross product:
n=a×b
The equation of the plane can be expressed as:
n⋅(r−r0)=0
where r0 is a point on the plane. We can choose r0=a or b since both lines pass through these points.
Hint: Remember that the plane equation can be derived from the normal vector and a point on the plane.
Step 4: Substitute to find the plane equation
Substituting r0=a into the plane equation gives:
(a×b)⋅(r−a)=0
This simplifies to:
(a×b)⋅r=(a×b)⋅a
Since a×a=0, we can conclude that the plane indeed passes through the origin if we set r=0.
Hint: The condition for the plane to pass through the origin is that the right-hand side of the equation equals zero.
Step 5: Conclusion about the statements
From the analysis:
- Statement I: L1 and L2 are coplanar, and the plane containing these lines passes through the origin. This is true.
- Statement II: The condition (a−b)⋅(a×b)=0 is indeed a condition for coplanarity and confirms that the plane passes through the origin. This is also true.
Thus, both statements are true.
Final Answer: Both Statement I and Statement II are true.
The lines are given as:
- L1:r=a+λb
- L2:r=b+μa
To check if the lines L1 and L2 are coplanar, we can use the condition involving the scalar triple product. The lines are coplanar if:
The normal vector to the plane containing the lines can be found using the cross product:
Substituting r0=a into the plane equation gives:
From the analysis:
- Statement I: L1 and L2 are coplanar, and the plane containing these lines passes through the origin. This is true.
- Statement II: The condition (a−b)⋅(a×b)=0 is indeed a condition for coplanarity and confirms that the plane passes through the origin. This is also true.
Topper's Solved these Questions
- THREE DIMENSIONAL COORDINATE SYSTEMBOOK - ARIHANT MATHS ENGLISHCHAPTER - THREE DIMENSIONAL COORDINATE SYSTEMEXERCISE - Exercise (Passage Based Questions)
33 Videos
- THREE DIMENSIONAL COORDINATE SYSTEMBOOK - ARIHANT MATHS ENGLISHCHAPTER - THREE DIMENSIONAL COORDINATE SYSTEMEXERCISE - Three Dimensional Coordinate System Exercise 9 : Match Type Questions
7 Videos
- THREE DIMENSIONAL COORDINATE SYSTEMBOOK - ARIHANT MATHS ENGLISHCHAPTER - THREE DIMENSIONAL COORDINATE SYSTEMEXERCISE - Exercise (More Than One Correct Option Type Questions)
28 Videos
- THEORY OF EQUATIONSBOOK - ARIHANT MATHS ENGLISHCHAPTER - THEORY OF EQUATIONSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
35 Videos
- TRIGONOMETRIC EQUATIONS AND INEQUATIONSBOOK - ARIHANT MATHS ENGLISHCHAPTER - TRIGONOMETRIC EQUATIONS AND INEQUATIONSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
12 Videos
Similar Questions
If L1 is the line of intersection of the planes 2x−2y+3z−2=0x−y+z+1=0 and L2 is the line of the intersection of the planes x+2y−z−3=0 3x−y+2z−1=0then the distance of the origin from the plane containing the lines L1 and L2 is
View Solution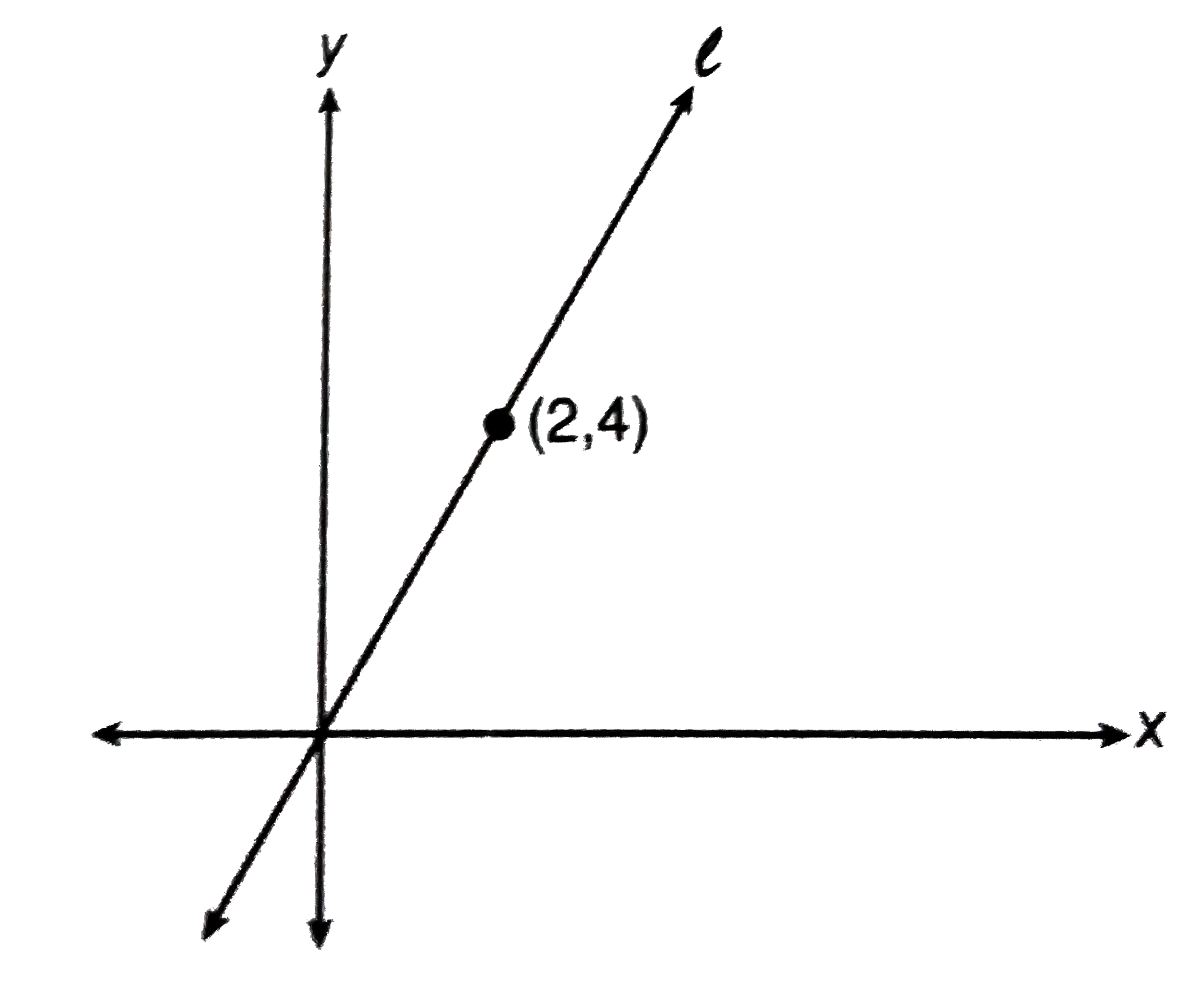
In the accompanying figure. Line l passes through the origin and the point (2, 4). Line m (not shown) is perpendicular to line l at (2, 4). Line m intersects the x-axis at which point?
View Solution
Knowledge Check
Line L passes through the coordinate points (−72,3)and(−32,5). What is the slope of line L?
A−1B−25C25D1
Statement -1 : The lines (a + b) x + 2(a - b) y = 2a are concurrent at the point (1, 1/2) .
Statement-2 : L1+λL2=0 represents the equation of family of lines passing through the intersection of the lines L1=0 and L2=0 for all non - zero finite value of λ
View SolutionConsider the lines L1≡3x−4y+2=0 and L2≡3y−4x−5=0. Now, choose the correct statement(s).
(a)The line x+y=0 bisects the acute angle between L1and L2 containing the origin.
(b)The line x-y+1=0 bisects the obtuse angle between L1 and L2 not containing the origin.
(c)The line x+y+3=0 bisects the obtuse angle between L1 and L2 containing the origin.
(d)The line x-y+1=0 bisects the acute angle between L1 and L2 not containing the origin.
View Solutionlines L1:ax+by+c=0 and L2:lx+my+n=0 intersect at the point P and make a angle θ between each other. find the equation of a line Ldifferent from L2 which passes through P and makes the same angle θ with L1
View SolutionThe area (in square units ) of the triangle formed by y-axis , the straight line L passing through (1,1) and (2,0) and the straight line perpendicular to the line L and passing through (1/2, 0) , is
View SolutionLet a given line L1 intersect the X and Y axes at P and Q respectively. Let another line L2 perpendicular to L1 cut the X and Y-axes at Rand S, respectively. Show that the locus of the point of intersection of the line PS and QR is a circle passing through the origin
View SolutionConsider a plane x+y−z=1 and point A(1,2,−3). A line L has the equation x=1+3r,y=2−randz=3+4r. The equation of the plane containing line L and point A has the equation
View SolutionPoints (3, 0) and (-1,0) are invariant points under reflection in the line L1 points (0, -3) and (0, 1) are invariant points on reflection in line L2
Name or write equations for the lines L1 and L2
View Solution
