In ΔABC,ifAC=8,BC=7 and D lies between A and B such that AD=2,BD=4, then the length CD equals
√46
√48
√51
√75
The correct Answer is:A
To solve the problem, we will follow these steps systematically:
Step 1: Understand the given information
We have triangle ABC with the following dimensions:
- AC = 8
- BC = 7
- D lies between A and B such that AD = 2 and BD = 4.
Step 2: Determine the length of AB
Since D lies between A and B, we can find the length of AB:
AB=AD+BD=2+4=6
Step 3: Apply the Cosine Law to find CD
We will use the Cosine Law in triangle ACD to find the length of CD. The Cosine Law states:
c2=a2+b2−2ab⋅cos(C)
Here, we will denote:
- AC = b = 8
- AD = a = 2
- CD = c (which we need to find)
- Angle CAB = C
Step 4: Find cos(C) using the Cosine Law in triangle ABC
To find cos(C), we can use the Cosine Law in triangle ABC:
BC2=AB2+AC2−2⋅AB⋅AC⋅cos(C)
Substituting the known values:
72=62+82−2⋅6⋅8⋅cos(C)
Calculating:
49=36+64−96⋅cos(C)
49=100−96⋅cos(C)
96⋅cos(C)=100−49
96⋅cos(C)=51
cos(C)=5196
Step 5: Substitute values into the Cosine Law for triangle ACD
Now we substitute back into the Cosine Law for triangle ACD:
CD2=AC2+AD2−2⋅AC⋅AD⋅cos(C)
Substituting the values:
CD2=82+22−2⋅8⋅2⋅5196
Calculating:
CD2=64+4−2⋅8⋅2⋅5196
=64+4−81696
=68−8.5
=59.5
Step 6: Find CD
Now we take the square root to find CD:
CD=√59.5
Final Answer
Thus, the length of CD is:
CD=√59.5
We have triangle ABC with the following dimensions:
- AC = 8
- BC = 7
- D lies between A and B such that AD = 2 and BD = 4.
Since D lies between A and B, we can find the length of AB:
AB=AD+BD=2+4=6
We will use the Cosine Law in triangle ACD to find the length of CD. The Cosine Law states:
c2=a2+b2−2ab⋅cos(C)
Here, we will denote:
- AC = b = 8
- AD = a = 2
- CD = c (which we need to find)
- Angle CAB = C
To find cos(C), we can use the Cosine Law in triangle ABC:
BC2=AB2+AC2−2⋅AB⋅AC⋅cos(C)
Substituting the known values:
72=62+82−2⋅6⋅8⋅cos(C)
49=36+64−96⋅cos(C)
49=100−96⋅cos(C)
96⋅cos(C)=100−49
96⋅cos(C)=51
cos(C)=5196
Now we substitute back into the Cosine Law for triangle ACD:
CD2=AC2+AD2−2⋅AC⋅AD⋅cos(C)
Substituting the values:
CD2=82+22−2⋅8⋅2⋅5196
Calculating:
CD2=64+4−2⋅8⋅2⋅5196
=64+4−81696
=68−8.5
=59.5
Now we take the square root to find CD:
CD=√59.5
Thus, the length of CD is:
CD=√59.5
Topper's Solved these Questions
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHS ENGLISHCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - SOLVED EXAMPLES
1 Videos
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHS ENGLISHCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise For Sesssion 1
20 Videos
- PRODUCT OF VECTORSBOOK - ARIHANT MATHS ENGLISHCHAPTER - PRODUCT OF VECTORSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
51 Videos
- SEQUENCES AND SERIESBOOK - ARIHANT MATHS ENGLISHCHAPTER - SEQUENCES AND SERIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
38 Videos
Similar Questions
In the ΔABC,AB=a,AC=candBC=b, then
View SolutionIn ΔABC,AB=ACandD is a point an side AC such that AD = BD = BC . Show that : ∠ADB=108∘
View SolutionIn ΔABC,AB=AC and D is a point in BC so that BD=CD. Prove that AD bisects ∠BAC.
View Solutionin a figure DE||BC AD=3cm, BD =4cm and BC=14cm, then DE equals
View SolutionIn ΔABC,∠ABC=∠DAC, AB = 8 cm, AC = 4 cm and AD = 5 cm.
(ii) Find the length of BC and CD.
View SolutionIn a ΔABC if a = 7 , b = 8 and c = 9 , then the length of the line joining B to the mid-points of AC is
View SolutionIn a ΔABC,B=90∘,AC=h and the length of perpendicular from B to AC is p such that h=4p. If AB<BC, then measure ∠C.
View Solution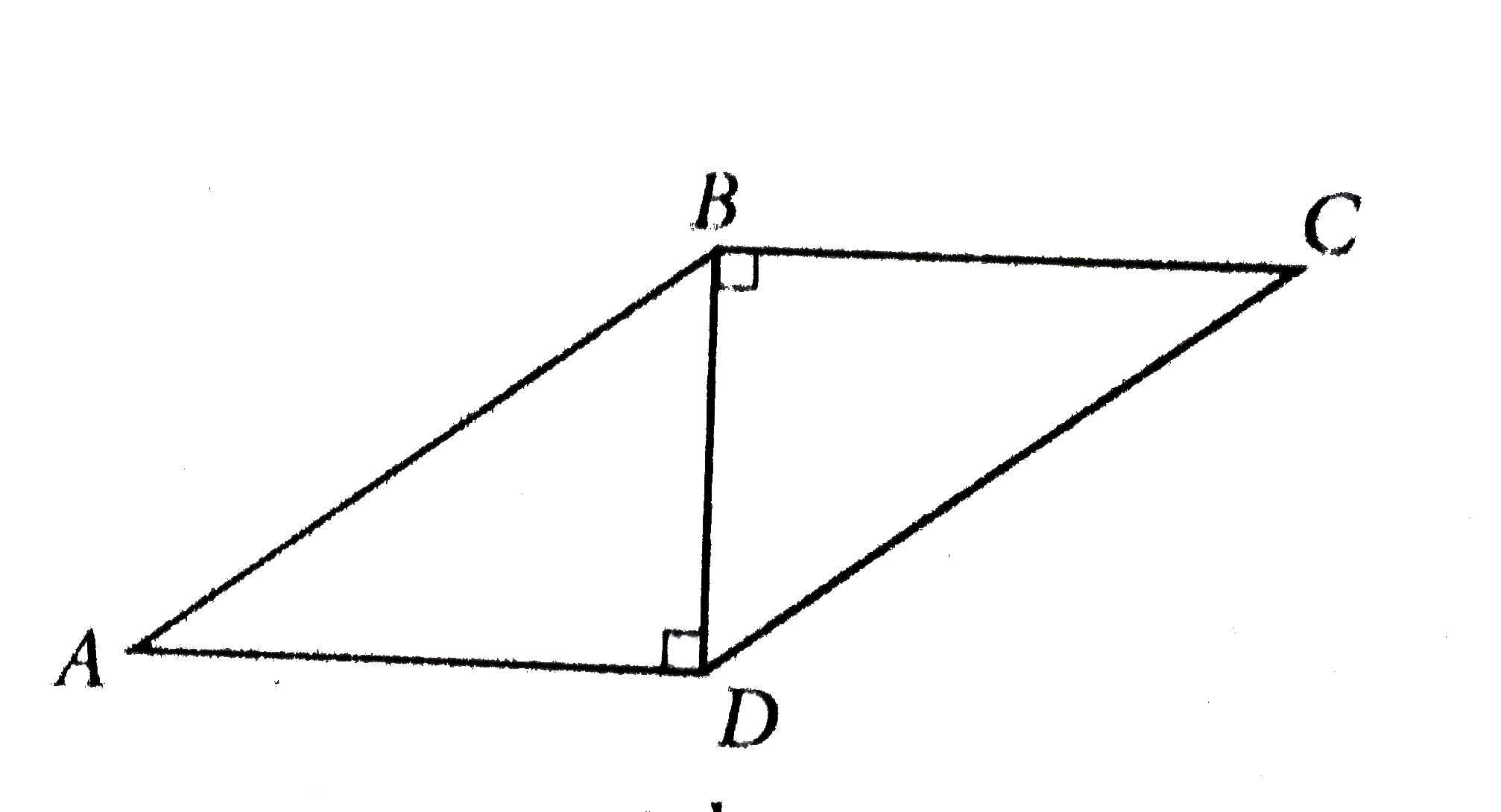
If AD=2CD,andDB=BC=6, what is the length of side AB?
View SolutionIn ΔABC,AB=AC and the bisectors of ∠B and ∠C meet AC and AB at point D and E respectively. Prove that BD=CE.
View SolutionIn ΔABC,∠B=90∘ and BD⊥AC.
If AC = 18 cm and AD = 6 cm, find BD.
View Solution
