Question

Find the coefficient of $x^7$ in the expansion of ${(1+3x-2x^3)}^{10}$.

Explanation

Coefficient of ` x^(7)` in the expansion of `(1 + 3x - 2x^(3))^(10)` is
`=sum (10!)/(alpha ! beta ! gamma !)(1)^(alpha) (3)^(beta)(-2)^(gamma)`
where , ` alpha + beta + gamma = 10 and beta + 3gamma = 7`
The possible values of ` alpha , beta " and" gamma ` are given below

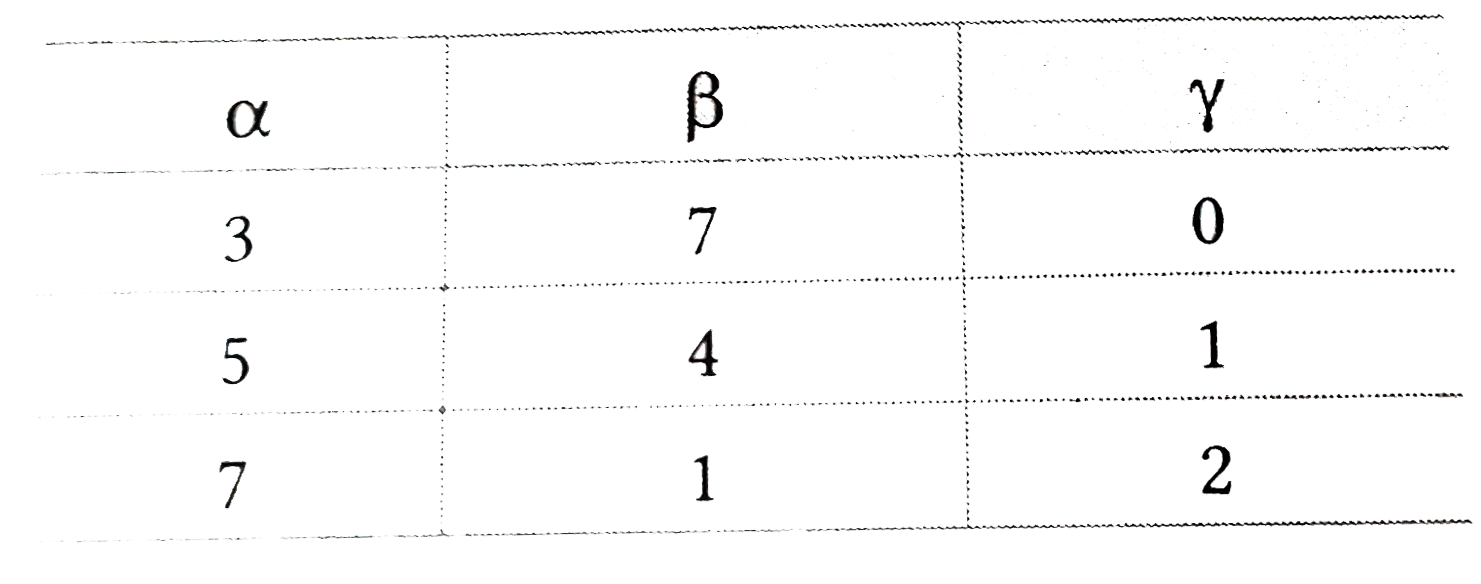
`therefore ` Coefficient of `x^(7)`
`= (10!)/(3!7!0!) (1)^(3) (3)^(7) (-2)^(0) + (10!)/(5! 4!1!) (1)^(5) (3)^(4) (-2)^(1) + (10!)/(7!1!2!) (1)^(7) (3)^(1)-(-2)^(2)`
` = 262440 - 204120 + 4320 = 62640` .