The vertices of ΔABC are A(0,6), B(8,12) and C(8,0). The cordinates of the incentre are:
To find the coordinates of the incenter of triangle ABC with vertices A(0, 6), B(8, 12), and C(8, 0), we will follow these steps:
Step 1: Calculate the lengths of the sides of the triangle
We will use the distance formula to find the lengths of the sides opposite to each vertex.
- Length of side a (opposite vertex A):
a=BC=√(xB−xC)2+(yB−yC)2=√(8−8)2+(12−0)2=√0+144=12
- Length of side b (opposite vertex B):
b=AC=√(xA−xC)2+(yA−yC)2=√(0−8)2+(6−0)2=√64+36=√100=10
- Length of side c (opposite vertex C):
c=AB=√(xA−xB)2+(yA−yB)2=√(0−8)2+(6−12)2=√64+36=√100=10
Step 2: Use the incenter formula
The coordinates of the incenter (I) can be calculated using the formula:
Ix=a⋅xA+b⋅xB+c⋅xCa+b+c
Iy=a⋅yA+b⋅yB+c⋅yCa+b+c
Step 3: Substitute the values into the formula
- For the x-coordinate:
Ix=12⋅0+10⋅8+10⋅812+10+10=0+80+8032=16032=5
- For the y-coordinate:
Iy=12⋅6+10⋅12+10⋅012+10+10=72+120+032=19232=6
Step 4: Final coordinates of the incenter
Thus, the coordinates of the incenter I are:
I(5,6)
We will use the distance formula to find the lengths of the sides opposite to each vertex.
a=BC=√(xB−xC)2+(yB−yC)2=√(8−8)2+(12−0)2=√0+144=12
b=AC=√(xA−xC)2+(yA−yC)2=√(0−8)2+(6−0)2=√64+36=√100=10
c=AB=√(xA−xB)2+(yA−yB)2=√(0−8)2+(6−12)2=√64+36=√100=10
The coordinates of the incenter (I) can be calculated using the formula:
Ix=a⋅xA+b⋅xB+c⋅xCa+b+c
Iy=a⋅yA+b⋅yB+c⋅yCa+b+c
- For the x-coordinate:
Ix=12⋅0+10⋅8+10⋅812+10+10=0+80+8032=16032=5
Iy=12⋅6+10⋅12+10⋅012+10+10=72+120+032=19232=6
Thus, the coordinates of the incenter I are:
I(5,6)
Topper's Solved these Questions
- THE STRAIGHT LINESBOOK - ARIHANT MATHS ENGLISHCHAPTER - THE STRAIGHT LINESEXERCISE - Example
3 Videos
- THE STRAIGHT LINESBOOK - ARIHANT MATHS ENGLISHCHAPTER - THE STRAIGHT LINESEXERCISE - JEE Tyep Solved Examples : (Paragraph Based Questions)
12 Videos
- SETS, RELATIONS AND FUNCTIONS BOOK - ARIHANT MATHS ENGLISHCHAPTER - SETS, RELATIONS AND FUNCTIONS EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
12 Videos
- THEORY OF EQUATIONSBOOK - ARIHANT MATHS ENGLISHCHAPTER - THEORY OF EQUATIONSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
35 Videos
Similar Questions
Knowledge Check
If the vertices of a triangle are (u,0),(v,8), and (0,0) then the area of the triiangle is
A4|u|B2|v|C|uv|D2|uv|
The co-ordinates of the vertices of a ΔABC are A(1,0),B(3,6)andC(3,2). Find the length of its medians.
View SolutionThree vertices of a parallelogram are (0, 0, 0), (2, -1, 2) and (5, 6, 8) Find the coordinates of
the Fourth vertex.
View SolutionThree vertices of a parallelogram ABCD are B (6,7) , C (8,3) and D (0,−1) . Find the co-ordinates of vertex A .
View SolutionThe vertices of a ΔABC are A(3, 8), B(-1, 2) and C(6, 6). Find :
(i) Slope of BC.
(ii) Equation of a line perpendicular to BC and passing through A.
View SolutionThe vertices of a ΔABC are A(3,0),B(0,6)andC(6,9). A straight line DE divides AB and AC in the ration 1:2 at D and E respectively, prove that ΔABCΔADE=9
View SolutionConsider the triangle whose vertices are (0,0),(5,12) and (16,12) Find coordinates of (i) Centroid (ii) Incentre (iii) Circumcentre
View SolutionThe vertices of a triangle are A(−10,8),B(14,8) and C(−10,26). Let G, I, H, O be the centroid, incentre, orthocentre, circumcentre respectively of ΔABC.
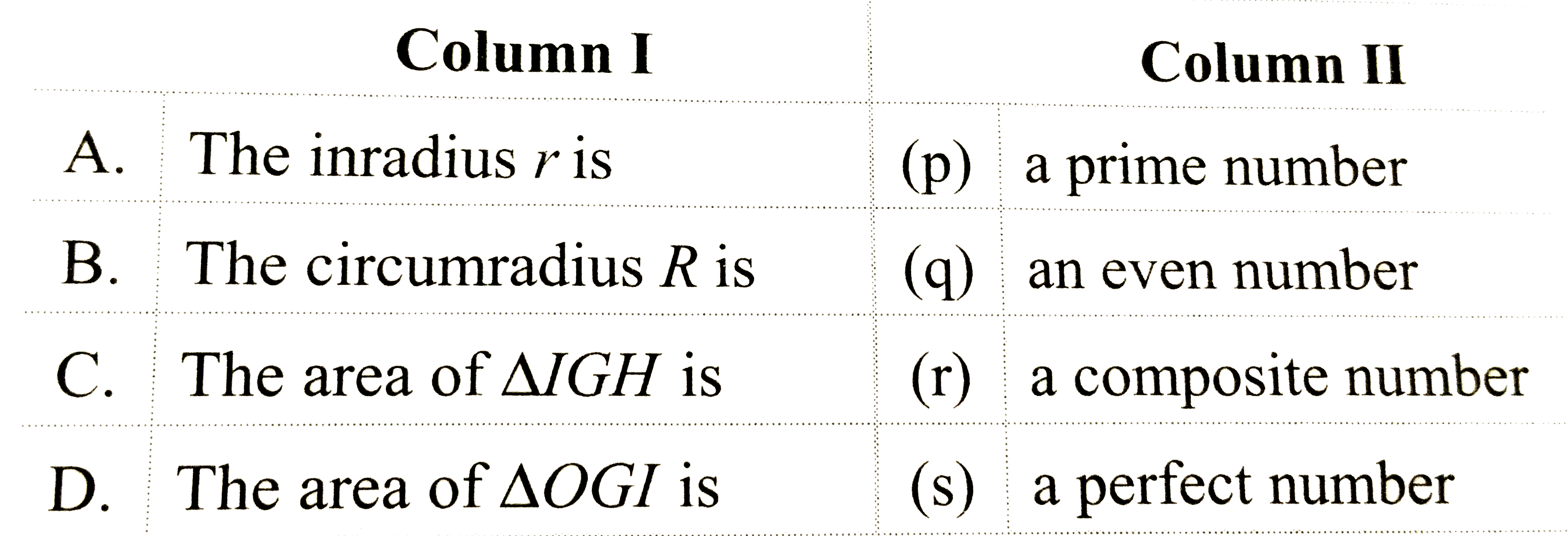
View Solution
