Let the vectors PQ,OR,RS,ST,TU and UP represent the sides of a regular hexagon.
Statement I:
Statement II:
Both statement I and statement II are correct and statement II is the correct explanation of statement I
both statement I and statement II are correct but statement II is not the correct explanation of statement I
Statement I is correct but statement II is incorrect
Statement II is correct but statement I is incorrect
The correct Answer is:C
To solve the problem, we need to analyze the two statements given regarding the vectors representing the sides of a regular hexagon.
1. Understanding the Geometry of a Regular Hexagon:
- A regular hexagon has equal sides and angles. Let's denote the vertices of the hexagon as P,Q,R,S,T,U.
- The vectors representing the sides of the hexagon are →PQ,→QR,→RS,→ST,→TU,→UP.
2. Analyzing Statement I:
- Statement I states: →PQ×(→RS+→ST)≠0.
- We can rewrite this using the distributive property of the cross product:
→PQ×→RS+→PQ×→ST≠0
3. Understanding the Cross Products:
- Since →PQ and →ST are parallel (as they are opposite sides of the hexagon), we have:
→PQ×→ST=0
- However, →PQ and →RS are not parallel; they form an angle θ (where 0<θ<180∘). Thus:
→PQ×→RS≠0
4. Conclusion for Statement I:
- Since →PQ×→RS is a non-zero vector and →PQ×→ST=0, we can conclude:
→PQ×(→RS+→ST)=→PQ×→RS+0=→PQ×→RS≠0
- Therefore, Statement I is True.
5. Analyzing Statement II:
- Statement II states: →PQ×→RS=0 and →PQ×→ST≠0.
- From our previous analysis, we know:
- →PQ×→RS≠0 (this is false).
- →PQ×→ST=0 (this is true).
6. Conclusion for Statement II:
- Since one part of Statement II is false, we conclude that Statement II is False.
Final Answer:
- Statement I is True, and Statement II is False.
- A regular hexagon has equal sides and angles. Let's denote the vertices of the hexagon as P,Q,R,S,T,U.
- The vectors representing the sides of the hexagon are →PQ,→QR,→RS,→ST,→TU,→UP.
- Statement I states: →PQ×(→RS+→ST)≠0.
- We can rewrite this using the distributive property of the cross product:
→PQ×→RS+→PQ×→ST≠0
- Since →PQ and →ST are parallel (as they are opposite sides of the hexagon), we have:
→PQ×→ST=0
- However, →PQ and →RS are not parallel; they form an angle θ (where 0<θ<180∘). Thus:
→PQ×→RS≠0
- Since →PQ×→RS is a non-zero vector and →PQ×→ST=0, we can conclude:
→PQ×(→RS+→ST)=→PQ×→RS+0=→PQ×→RS≠0
- Therefore, Statement I is True.
- Statement II states: →PQ×→RS=0 and →PQ×→ST≠0.
- From our previous analysis, we know:
- →PQ×→RS≠0 (this is false).
- →PQ×→ST=0 (this is true).
- Since one part of Statement II is false, we conclude that Statement II is False.
- Statement I is True, and Statement II is False.
Topper's Solved these Questions
- PRODUCT OF VECTORSBOOK - ARIHANT MATHS ENGLISHCHAPTER - PRODUCT OF VECTORSEXERCISE - JEE Type Solved Examples: Passage Based Type Questions
3 Videos
- PRODUCT OF VECTORSBOOK - ARIHANT MATHS ENGLISHCHAPTER - PRODUCT OF VECTORSEXERCISE - Exercise For Session 1
12 Videos
- PROBABILITYBOOK - ARIHANT MATHS ENGLISHCHAPTER - PROBABILITYEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
54 Videos
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHS ENGLISHCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
21 Videos
Similar Questions
Let the vectors −−→PQ,−−→QR,−−→RS,−→ST,−−→TU and −−→UP represent the sides of a regular hexagon.
Statement I:−−→PQ×(−−→RS+−→ST)≠→0
Statement II: −−→PQ×−−→RS=→0 and −−→PQ×−−→RS=→0 and −−→PQ×−→ST≠→0
For the following question, choose the correct answer from the codes (A), (B) , (C) and (D) defined as follows:
View SolutionFor the following question, choose the correct answer from the codes (a), (b), (c) and (d) defined as follows: Statement I is true, Statement II is also true; Statement II is the correct explanation of Statement I. Statement I is true, Statement II is also true; Statement II is not the correct explanation of Statement I. Statement I is true; Statement II is false Statement I is false; Statement II is true. Let a,b,c,p,q be the real numbers. Suppose α,β are the roots of the equation x2+2px+q=0 and α,1β are the roots of the equation ax2+2bx+c=0, where β2∉{−1,0,1}. Statement I (p2−q)(b2−ac)≥0 and Statement II b∉pa or c∉qa.
View SolutionGiven : A circle, 2x2+2y2=5 and a parabola, y2=4√5x . Statement - I : An equation of a common tangent to these curves is y=x+√5 Statement - II : If the line, y=mx+√5m(m≠0) is their common tangent, then m satisfies m4−3m2+2=0. (1) Statement - I is True; Statement -II is true; Statement-II is not a correct explanation for Statement-I (2) Statement -I is True; Statement -II is False. (3) Statement -I is False; Statement -II is True (4) Statement -I is True; Statement -II is True; Statement-II is a correct explanation for Statement-I
View SolutionStatement I: If a is perpendicular to b and c, then a×(b×c)=0 Statement II: if a is perpendicular to b and c, then b×c=0
View SolutionStatement I The number of solution of the equation |sinx|=|x| is only one.
Statement II |sinx|≥0∀x∈R.
View Solution
Statement-1 : limx→0√1−cos2xx at (x = 0) does not exist.
Statement -2 :Right hand limit ≠ Left hand limit
i) Statement - 1 is True, Statement-2 is True, Statement-2 is a correct explanation for statement-1
ii)Statement-1 is True, Statement-2 is True, Statement-2 is Not a correct explanation for statement-1
iii)Statement-1 is True, Statement-2 is False
iv)Statement -1 is False, Statement-2 is True
View SolutionAssertion: Minimum number of non-equal Vectors in a plane required to give zero resultant is three.
Reason: If →A+→B+→C=→0, then they must lie in one plane
A. Statement-I is true, Statement-II is true, Statement-II is correct explanation for statement-I
B. Statement-I is true, Statement-II is true, Statement-II is NOT a correct explanation for statement-I
C. Statement-I is true, Statement-II is false.
D. Statement-I is false and Statement-II is true.
View SolutionStatement I. The four straight lines given by
6x2+5xy−6y2=0and6x2+5xy−6y2−x+5y−1=0 are the sides of a square .
Statement II . The lines represented by general equation of second degree ax2+2hxy+by2+2gx+2fy+c=0 are perpendicular if a+b=0 .
View SolutionLet A1A2A3A4A5A6A1 be a regular hexagon. Write the x-components of the vectors representeed by the six sides taken in order. Use the fact that the resultant of these six vectors is zero, to prove that cos0+cosπ3+cos(2π)3+cos(4π)3+cos(5π)3=0 Use the known cosine values of verify the result.
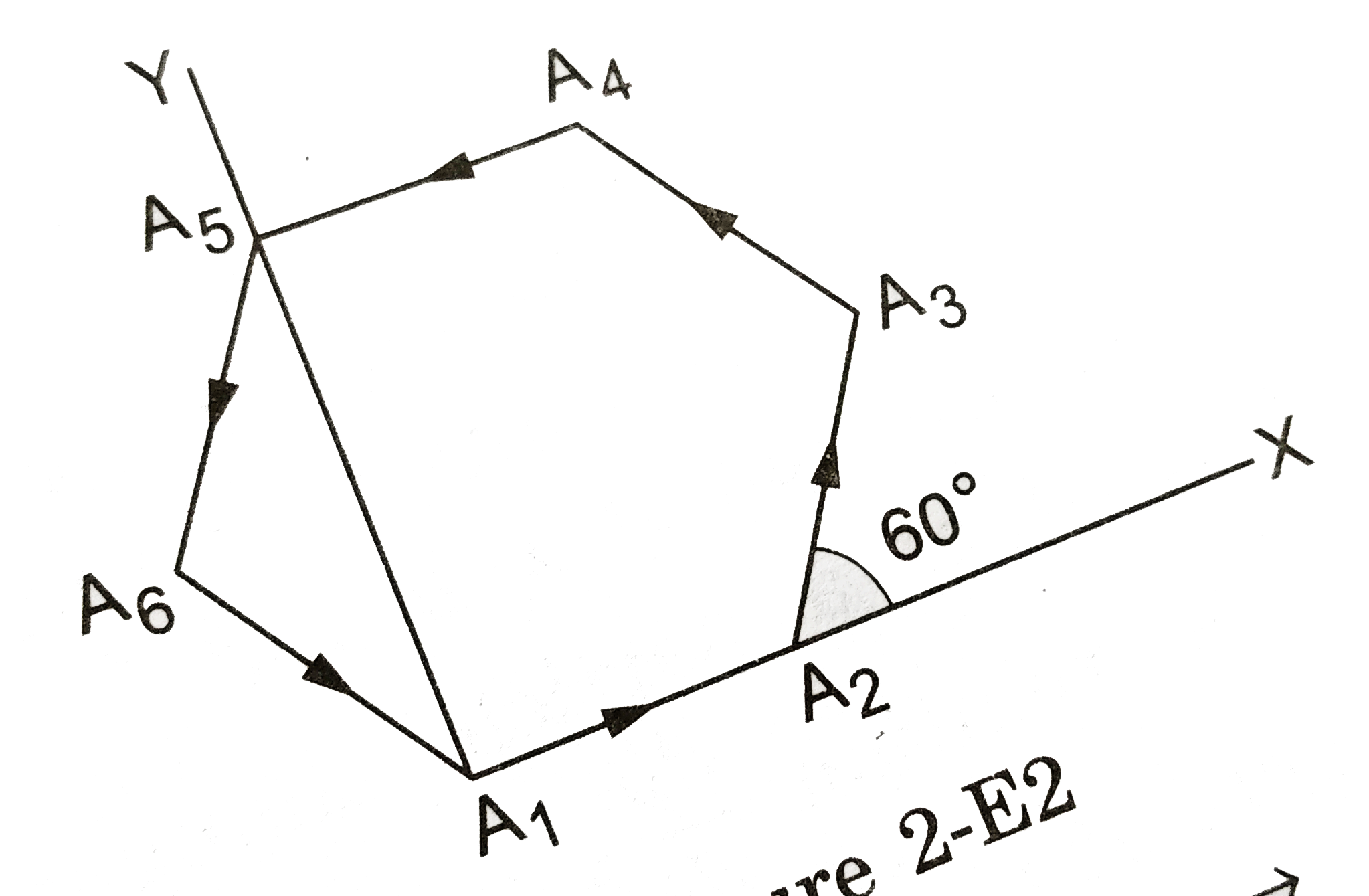 .
.
View Solutionstatement-I : 2A +X0 genotype represents turner syndrome in woman .
Statement-II : 2A +X0 genotype is normal male in grasshopper .
View Solution
