In a class of 75 students, 15 are above average, 45 are average and the rest below average achievers. The probability that an above average achieving student fails is 0.005, that an average achieving student fails is 0.05 and the probability of a below average achieving student failing is 0.15. If a student is known to have passed, what is the probability that he is a below average achiever?
The correct Answer is:0.181
To solve the problem step by step, we will use the concepts of probability and conditional probability.
Step 1: Define the events
Let:
- A1: Event that a student is above average.
- A2: Event that a student is average.
- A3: Event that a student is below average.
- E: Event that a student passes.
Step 2: Calculate the probabilities of each event
- Total number of students = 75
- Number of above average students = 15
- Number of average students = 45
- Number of below average students = 75−(15+45)=15
Now, we can calculate the probabilities:
- P(A1)=1575=15
- P(A2)=4575=35
- P(A3)=1575=15
Step 3: Calculate the probabilities of passing given each event
- Probability of passing for above average students:
P(E|A1)=1−P(Fail|A1)=1−0.005=0.995
- Probability of passing for average students:
P(E|A2)=1−P(Fail|A2)=1−0.05=0.95
- Probability of passing for below average students:
P(E|A3)=1−P(Fail|A3)=1−0.15=0.85
Step 4: Calculate the total probability of passing
Using the law of total probability:
P(E)=P(A1)⋅P(E|A1)+P(A2)⋅P(E|A2)+P(A3)⋅P(E|A3)
Substituting the values:
P(E)=(15⋅0.995)+(35⋅0.95)+(15⋅0.85)
Calculating each term:
- 15⋅0.995=0.199
- 35⋅0.95=0.57
- 15⋅0.85=0.17
Now summing them up:
P(E)=0.199+0.57+0.17=0.939
Step 5: Calculate the conditional probability that a student is below average given that they passed
We want to find P(A3|E):
P(A3|E)=P(A3)⋅P(E|A3)P(E)
Substituting the values:
P(A3|E)=(15⋅0.85)0.939
Calculating the numerator:
15⋅0.85=0.17
Now substituting back:
P(A3|E)=0.170.939≈0.181
Final Answer
The probability that a student is a below average achiever given that they passed is approximately 0.181.
Let:
- A1: Event that a student is above average.
- A2: Event that a student is average.
- A3: Event that a student is below average.
- E: Event that a student passes.
- Total number of students = 75
- Number of above average students = 15
- Number of average students = 45
- Number of below average students = 75−(15+45)=15
- P(A1)=1575=15
- P(A2)=4575=35
- P(A3)=1575=15
- Probability of passing for above average students:
P(E|A1)=1−P(Fail|A1)=1−0.005=0.995
- Probability of passing for average students:
P(E|A2)=1−P(Fail|A2)=1−0.05=0.95
- Probability of passing for below average students:
P(E|A3)=1−P(Fail|A3)=1−0.15=0.85
Using the law of total probability:
P(E)=P(A1)⋅P(E|A1)+P(A2)⋅P(E|A2)+P(A3)⋅P(E|A3)
Substituting the values:
P(E)=(15⋅0.995)+(35⋅0.95)+(15⋅0.85)
Calculating each term:
- 15⋅0.995=0.199
- 35⋅0.95=0.57
- 15⋅0.85=0.17
P(E)=0.199+0.57+0.17=0.939
We want to find P(A3|E):
P(A3|E)=P(A3)⋅P(E|A3)P(E)
Substituting the values:
P(A3|E)=(15⋅0.85)0.939
Calculating the numerator:
15⋅0.85=0.17
P(A3|E)=0.170.939≈0.181
The probability that a student is a below average achiever given that they passed is approximately 0.181.
Topper's Solved these Questions
- MATHEMATICS-2012Book:ICSEChapter:MATHEMATICS-2012Exercise:SECTION-C
Explore 6 Videos
- MATHEMATICS-2012Book:ICSEChapter:MATHEMATICS-2012Exercise:SECTION-C
Explore 6 Videos
- MATHEMATICS-2011Book:ICSEChapter:MATHEMATICS-2011Exercise:SECTION-C
Explore 6 Videos
- MATHEMATICS-2015Book:ICSEChapter:MATHEMATICS-2015Exercise:SECTION-C
Explore 5 Videos
Similar Questions
In a class of 75 students, 15 are above average, 45 are average and the rest below average achievers. The probability than an above average achieving student fails is 0.005, that an average achieving student fails is 0.05 and the probabilit of a below average achieving students failing is 0.15. If a student is known to have passed, what is the probability that he is a below average achiever?
View Solution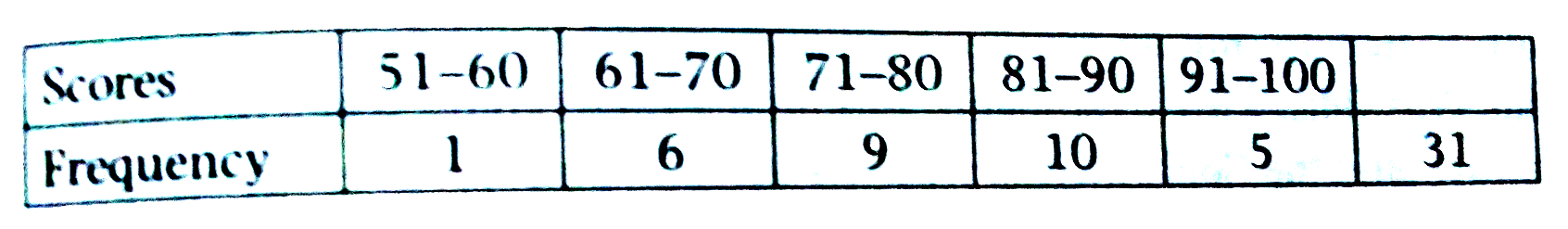
The frequency chart shows test scores for a certain class. The total number of student is 31. If a student in the class was picked at random , what is the probability that the student's score was below 71 ?
View SolutionIn a class of 100 students, there are 70 boys whose average marks in a subject is 75. If the average marks of the complete class is 72, then what is the average of the girls ?
View SolutionIn a college, 70% students pass in Physics, 75% pass in Mathematics and 10% students fail in both. One student is chosen at random. What is the probability that:
He passes in Physics and Mathematics.
View SolutionThe probability that a student will pass the final examination in both English and Hindi is 0.5 and the probability of passing neither is 0.1. If the probability of passing the English examination is 0.75. What is the probability of passing the Hindi examination?
View SolutionIn an entrance test that is graded on the basis of two examinations, the probability of a randomly chosen student passing the first examination is 0.8 and the probability of passing the second examination is 0.7. The probability of passing at least one of them is 0.95. What is the probability of passing both?
View SolutionIn an entrance test that is graded on the basis of two examinations, the probability of a randomly chosen student passing the first examination is 0.8 and the probability of passing the second examination is 0.7. The probability of passing at least one of them is 0.95. What is the probability of passing both?
View SolutionIn a class, 30% students fail in English, 20% students fail in Hindi and 10% students fail in both English and Hindi. A student is chosen at random, then what is the probability he fail in English, if he has failed in Hindi?
View SolutionIt is given that in a group of 3 students, the probability of 2 students not having the same birthday is 0.992. What is the probability that the 2 students have the same birthday?
View SolutionIt is given that in a group of 3 students, the probability of 2 students not having the same birthday is 0.992. What is the probability that the 2 students have the same birthday?
View Solution