The following particles are moving with the same velocity. Which particle has maximum momentum ?
β particle
proton
α particle
neutron
To determine which particle has the maximum momentum among a beta particle, proton, alpha particle, and neutron, we can follow these steps:
Step 1: Understand the formula for momentum
Momentum (p) is defined as the product of mass (m) and velocity (v):
p=m×v
Since all particles are moving with the same velocity, we can express their momenta in terms of their masses:
p∝m
This means that the momentum of each particle is directly proportional to its mass.
Step 2: Identify the masses of the particles
- Beta Particle (Electron): The mass of a beta particle (electron) is approximately 9.11×10−31 kg.
- Proton: The mass of a proton is approximately 1.67×10−27 kg.
- Neutron: The mass of a neutron is also approximately 1.67×10−27 kg (similar to the proton).
- Alpha Particle: An alpha particle consists of 2 protons and 2 neutrons. Therefore, its mass is approximately:
Mass of alpha particle=2×mass of proton+2×mass of neutron
≈2(1.67×10−27)+2(1.67×10−27)=4×1.67×10−27≈6.68×10−27 kg
Step 3: Compare the masses
Now we can compare the masses of the particles:
- Mass of beta particle: 9.11×10−31 kg
- Mass of proton: 1.67×10−27 kg
- Mass of neutron: 1.67×10−27 kg
- Mass of alpha particle: 6.68×10−27 kg
Step 4: Determine which particle has the maximum mass
From the comparison:
- The beta particle has the least mass.
- The proton and neutron have the same mass.
- The alpha particle has the greatest mass.
Step 5: Conclusion
Since momentum is proportional to mass and all particles have the same velocity, the particle with the maximum mass will have the maximum momentum. Therefore, the alpha particle has the maximum momentum.
Final Answer:
The alpha particle has the maximum momentum.
---
Momentum (p) is defined as the product of mass (m) and velocity (v):
p=m×v
Since all particles are moving with the same velocity, we can express their momenta in terms of their masses:
p∝m
This means that the momentum of each particle is directly proportional to its mass.
- Beta Particle (Electron): The mass of a beta particle (electron) is approximately 9.11×10−31 kg.
- Proton: The mass of a proton is approximately 1.67×10−27 kg.
- Neutron: The mass of a neutron is also approximately 1.67×10−27 kg (similar to the proton).
- Alpha Particle: An alpha particle consists of 2 protons and 2 neutrons. Therefore, its mass is approximately:
Mass of alpha particle=2×mass of proton+2×mass of neutron
≈2(1.67×10−27)+2(1.67×10−27)=4×1.67×10−27≈6.68×10−27 kg
Now we can compare the masses of the particles:
- Mass of beta particle: 9.11×10−31 kg
- Mass of proton: 1.67×10−27 kg
- Mass of neutron: 1.67×10−27 kg
- Mass of alpha particle: 6.68×10−27 kg
From the comparison:
- The beta particle has the least mass.
- The proton and neutron have the same mass.
- The alpha particle has the greatest mass.
Since momentum is proportional to mass and all particles have the same velocity, the particle with the maximum mass will have the maximum momentum. Therefore, the alpha particle has the maximum momentum.
The alpha particle has the maximum momentum.
Topper's Solved these Questions
- SELF ASSESSMENT PAPER 4BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 4EXERCISE - Section-A
7 Videos
- SELF ASSESSMENT PAPER 4BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 4EXERCISE - Section-B
13 Videos
- SELF ASSESSMENT PAPER 3BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 3EXERCISE - Section-D
6 Videos
- SELF ASSESSMENT PAPER 5BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 5EXERCISE - Section-D
6 Videos
Similar Questions
If particles are moving with same velocity , then maximum de - Broglie wavelength will be for
View SolutionAssertion: In successive time intervals if the average velocities of a particle are equal then the particle must be moving with constant velocity.
Reason: When a particle moves with uniform velocity, its displacement always increases with time.
View SolutionA particle moves on a straight line with a uniform velocity. It's angular momentum
View SolutionThe momentum of a particle of mass of m moving with a velocity v is p= mv. What is the rate of change of momentum ? Assume that m is constant .
View SolutionTwo particles are moving with velocities v1andv2 . Their relative velocity is the maximum, when the angle between their velocities is
View SolutionA particle of mass m1 is moving with a velocity v1 and another particle of mass m2 is moving with a velocity v2. Both of them have the same momentum but their different kinetic energies are E1 and E2 respectively. If m1>m2 then
View SolutionAssertion: In projectile motion horizontal velocity remains same but vertical velocity continously change Reason: particle strikes the ground with same vertical velocity with which the particle was thrwon in vertical direction.
View Solution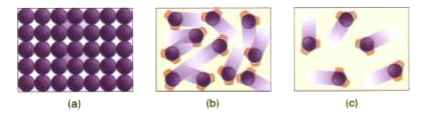
Which of the above state has particles with maximum kinetic energy?
View SolutionA small particle of mass m attached with a light inextensible thread of length L is moving in a verical circle. In the given case particle is moving in complete vertical circle and ratio of its maximum to minimum velocity is 2:1 .
Minimum velocity of the particle is
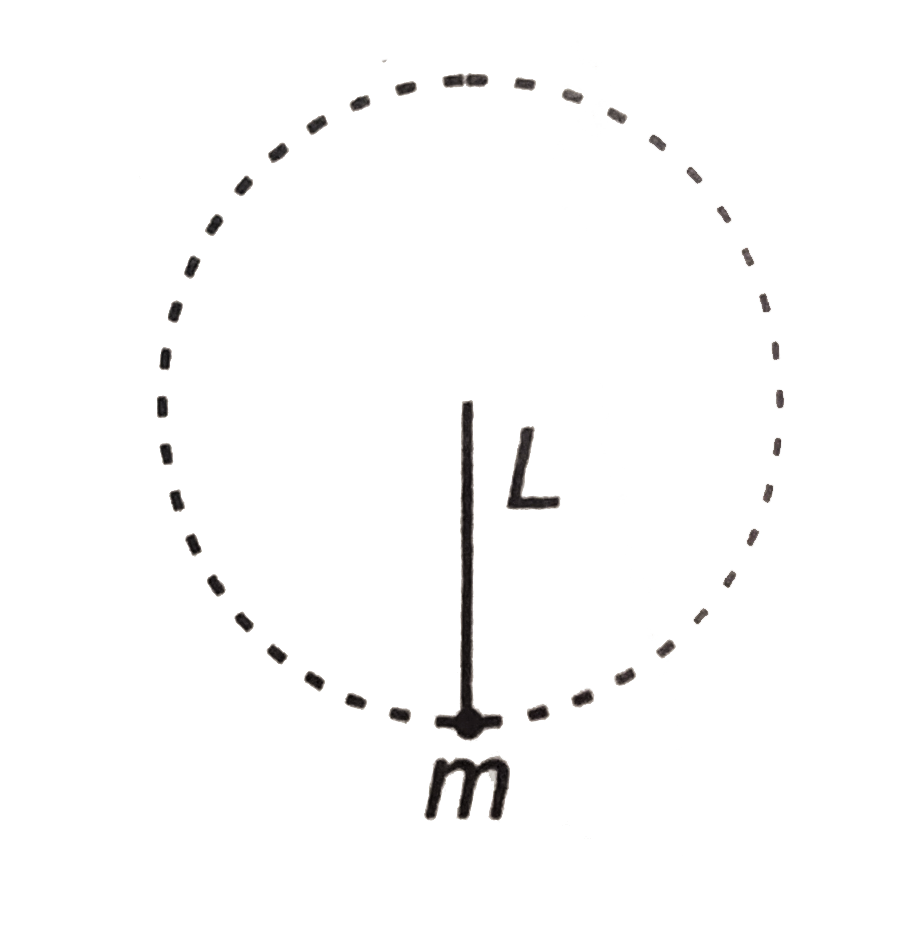
View SolutionAssertion: Two particles of different mass, projected with same velocity at same angles. The maximum height attained by both the particle will be same.
Reason: The maximum height of projetile is independent of particle mass.
View Solution
