Two particles are moving with velocities . Their relative velocity is the maximum, when the angle between their velocities is
zero
The correct Answer is:D
To solve the problem of finding the angle between the velocities v1 and v2 that maximizes their relative velocity, we can follow these steps:
1. Understanding Relative Velocity:
The relative velocity →vrel of one particle with respect to another is given by the vector difference of their velocities:
→vrel=→v1−→v2
2. Magnitude of Relative Velocity:
The magnitude of the relative velocity can be expressed using the law of cosines:
|→vrel|=|→v1−→v2|=√|→v1|2+|→v2|2−2|→v1||→v2|cosθ
where θ is the angle between the two velocity vectors.
3. Maximizing the Magnitude:
To find the maximum value of |→vrel|, we need to analyze the expression:
|→vrel|=√|→v1|2+|→v2|2−2|→v1||→v2|cosθ
The term −2|→v1||→v2|cosθ contributes negatively, so to maximize the magnitude, we need to minimize cosθ.
4. Finding the Angle:
The minimum value of cosθ occurs when θ=180∘ (or π radians), which corresponds to the two velocities being in opposite directions. In this case:
|→vrel|=|→v1|+|→v2|
5. Conclusion:
Therefore, the angle between the velocities v1 and v2 that maximizes their relative velocity is:
θ=180∘ or π radians
Final Answer:
The angle between their velocities is 180∘ or π radians.
---
The relative velocity →vrel of one particle with respect to another is given by the vector difference of their velocities:
→vrel=→v1−→v2
The magnitude of the relative velocity can be expressed using the law of cosines:
|→vrel|=|→v1−→v2|=√|→v1|2+|→v2|2−2|→v1||→v2|cosθ
where θ is the angle between the two velocity vectors.
To find the maximum value of |→vrel|, we need to analyze the expression:
|→vrel|=√|→v1|2+|→v2|2−2|→v1||→v2|cosθ
The term −2|→v1||→v2|cosθ contributes negatively, so to maximize the magnitude, we need to minimize cosθ.
The minimum value of cosθ occurs when θ=180∘ (or π radians), which corresponds to the two velocities being in opposite directions. In this case:
|→vrel|=|→v1|+|→v2|
Therefore, the angle between the velocities v1 and v2 that maximizes their relative velocity is:
θ=180∘ or π radians
The angle between their velocities is 180∘ or π radians.
Topper's Solved these Questions
- MOTION IN A STRAIGHT LINE & PLANE BOOK - VMC MODULES ENGLISHCHAPTER - MOTION IN A STRAIGHT LINE & PLANE EXERCISE - IMPECCABLE
52 Videos
- MOTION IN A STRAIGHT LINE & PLANE BOOK - VMC MODULES ENGLISHCHAPTER - MOTION IN A STRAIGHT LINE & PLANE EXERCISE - ENABLE
50 Videos
- MOCK TEST 9BOOK - VMC MODULES ENGLISHCHAPTER - MOCK TEST 9EXERCISE - PHYSICS (SECTION 2)
5 Videos
- Motion in Straight Line BOOK - VMC MODULES ENGLISHCHAPTER - Motion in Straight Line EXERCISE - IN-CHAPTER EXERCISE-J
10 Videos
Similar Questions
Statement-I : If two particles, moving with constant velocities are to meet, the relative velocity must be along the line joining the two particles.
Statement-II : Relative velocity means motion of one particle as viewed from the other.
View SolutionTwo particles A and B are moving with constant velocities V1=ˆj and v2=2ˆi respectively in XY plane. At time t=0, the particle A is at co-ordinates (0,0) and B is at (-4,0). The angular velocities of B with respect to A at t=2s is (all physical quantities are in SI units)
View SolutionTwo particles A and B moving in a plane have velocities as shown in figure .Relative velocity of A w.r.t B
View SolutionTwo particles 1and2 move with velocities →v1 and →v2 making the angles θ1andθ2 with the line joining them, respectively. Find angular velocity of relative→1.
 .
.
View SolutionTwo particles are moving with velocities v1=ˆi−tˆj+ˆk and v2=tˆi+tˆj+2ˆkm/s respectively. Time at which they are moving perpendicular to each other is.____________(second)
View SolutionTwo masses, m1 and m2 , are moving with velocities v1 and v2. Find their total kinetic energy in the reference frame of centre of mass.
View SolutionA particle moving with uniform acceleration from A to B along a straight line has velcities v1 and v2 at A and B respectively. If C is the mid-point between A and B then determine the velocity of the particle at C .
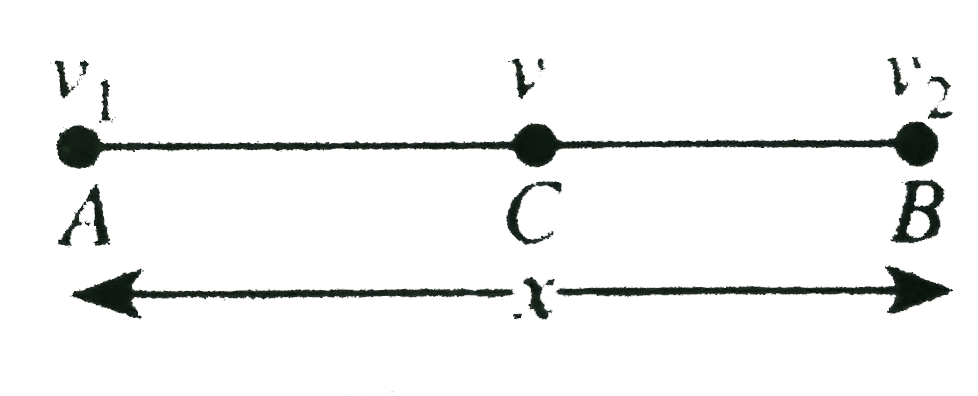 .
.
View SolutionA particle moves with initial velocity v0 and retardation αv, where v is velocity at any instant t. Then the particle
View SolutionA particle of mass m is projected upwards with velocity v=ve2, where ve is the escape velocity then at the maximum height the potential energy of the particle is : (R is radius of earth and M is mass of earth)
View SolutionTwo particles, 1 and 2, move with constant velocities v1 and v2. At the initial moment their radius vectors are equal to r1 and r2. How must these four vectors be interrelated for the particles to collide?
View Solution
