The moment of inertia of a flywheel is 4 kg-m2. What angular acceleration will be produced in it by applying a torque of 10 N-m on it ?
To find the angular acceleration produced in a flywheel when a torque is applied, we can use the relationship between torque, moment of inertia, and angular acceleration. The formula we will use is:
τ=I⋅α
Where:
- τ is the torque applied,
- I is the moment of inertia,
- α is the angular acceleration.
1. Identify the Given Values:
- Moment of inertia (I) = 4 kg·m²
- Torque (τ) = 10 N·m
2. Write the Formula for Angular Acceleration:
- From the torque equation, we can rearrange it to solve for angular acceleration (α):
α=τI
3. Substitute the Known Values into the Formula:
- Substitute τ=10N⋅m and I=4kg⋅m²:
α=10N⋅m4kg⋅m²
4. Calculate the Angular Acceleration:
- Perform the division:
α=2.5rad/s²
5. Final Answer:
- The angular acceleration produced in the flywheel is 2.5rad/s².
- τ is the torque applied,
- I is the moment of inertia,
- α is the angular acceleration.
- Moment of inertia (I) = 4 kg·m²
- Torque (τ) = 10 N·m
- From the torque equation, we can rearrange it to solve for angular acceleration (α):
α=τI
- Substitute τ=10N⋅m and I=4kg⋅m²:
α=10N⋅m4kg⋅m²
- Perform the division:
α=2.5rad/s²
- The angular acceleration produced in the flywheel is 2.5rad/s².
Topper's Solved these Questions
- BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 02EXERCISE - SECTION - C (Answer the following )8videos
SELF ASSESSMENT PAPER 02
- BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 02EXERCISE - SECTION-D (Answer the following )6videos
SELF ASSESSMENT PAPER 02
- BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 02EXERCISE - SECTION - A(Answer the following )7videos
SELF ASSESSMENT PAPER 02
- BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 01EXERCISE - SECION- D (Answer the following )6videos
SELF ASSESSMENT PAPER 01
- BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 3EXERCISE - Section-D6videos
SELF ASSESSMENT PAPER 3
Similar Questions
The product of moment of inertia (I) and angular acceleration (α) is called
View SolutionA flywheel having moment of inertia 20kgm2 rotates about its axis with an angular velocity of 100rads−1. If the moment of inertia of the flywheel is reduced to 10kgm2 without applying external torque, calculate the new angular velocity of the Flywheel.
View SolutionA flywheel having moment of inertia 20kgm2 rotates about its axis with an angular velocity of 100rads−1. If the moment of inertia of the flywheel is reduced to 10kgm2 without applying external torque, calculate the new angular velocity of the Flywheel.
View SolutionA wheel whose moment of inertia is 10kgm2 has an initial angular velocity of20rad/s. A constant torque of 20Nm acts on the wheel. The time in which wheel is acceleration to 100rad/s is
View SolutionA wheel of radius 0.4m can rotate freely about its axis as shown in the figure. A string is wrapped over its rim and a mass of 4kg is hung. An angular acceleration of 8rad/s2 is produced in it due to the torque. Then, the moment of inertia of the wheel is (g=10m/s2)
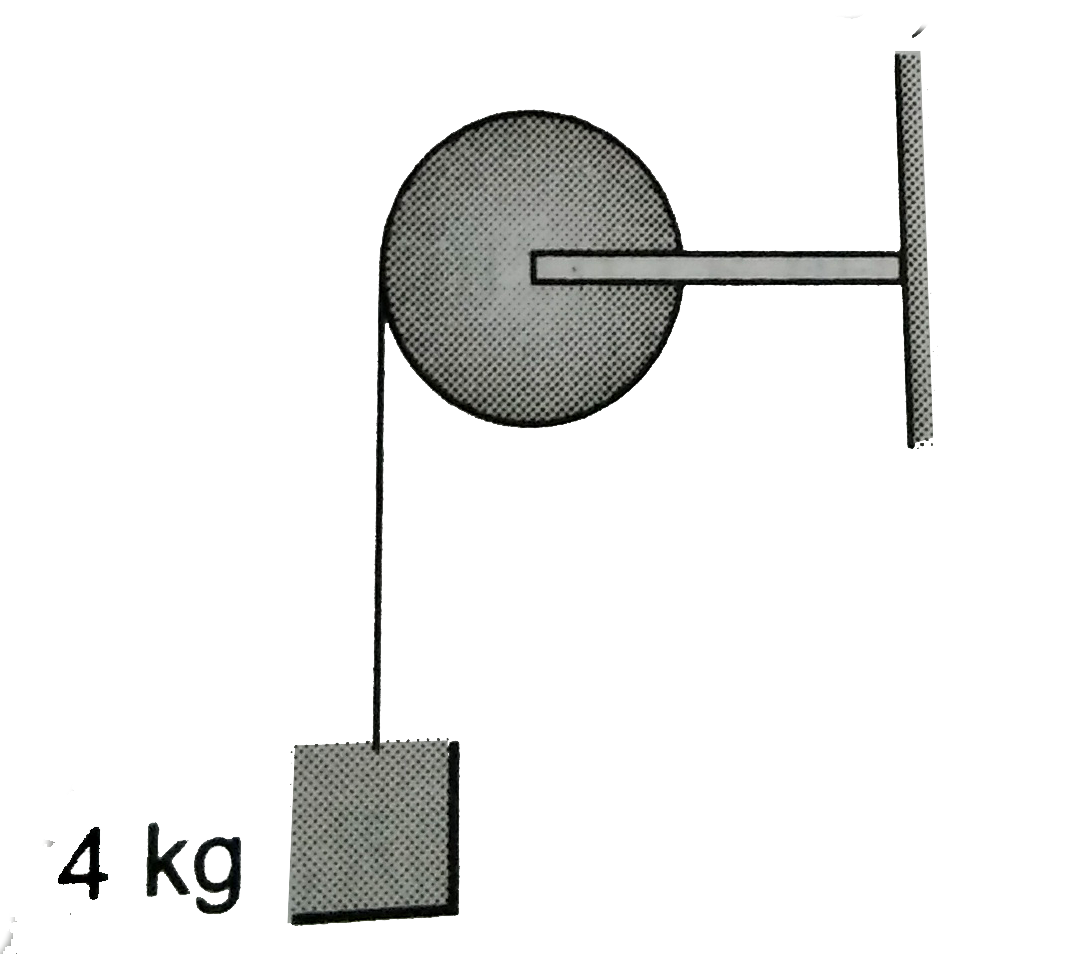 View Solution
View SolutionIf a tangential force mg is applied to a disc of mass m and radius r, the angular acceleration produced in it is?
View SolutionA 16 kg block is attached to a cord that is wrapped around the rim of a flywheel of diameter 0.4 m and hangs vertically, as shown in figure. The rotational inertia of the flywheel is 0.5 kg m2. When the block is released and the cord unwinds, the acceleration of the block is
 View Solution
View SolutionIn the following figure r1 and r2 are 5cm and 30cm repectively. If the moment of inertia of wheel is 5100kgm2 then its angular acceleration will be :−
 View Solution
View SolutionThe moment of inertia of a body about a given axis is 1.2kgm2. Initially, the body is at rest. In order to produce a rotational KE of 1500J, for how much duration, an acceleration of 25rads−2 must be applied about that axis ?
View SolutionThe moment of inertia of the body about an axis is 1.2 kg m2. Initially the body is at rest. In order to produce a rotational kinetic energy of 1500J, an angualr acceleration of 25 rads2 must be applied about the axis for the duration of
View Solution

