The internal energy of a compressed gas is less than that of a rarefied gas at the same temperature. Why ?
To understand why the internal energy of a compressed gas is less than that of a rarefied gas at the same temperature, we can break down the explanation into several steps.
1. Understanding Internal Energy:
- Internal energy (U) of a gas is the sum of the kinetic energy (KE) and potential energy (PE) of its molecules.
- Mathematically, it can be expressed as:
U=KE+PE
2. Kinetic Energy at Constant Temperature:
- The kinetic energy of gas molecules is directly related to the temperature of the gas.
- Since both the compressed gas and the rarefied gas are at the same temperature, their average kinetic energy is the same.
- Therefore, we can say:
KEcompressed=KErarefied
3. Potential Energy in Compressed Gas:
- When a gas is compressed, the molecules are brought closer together.
- This results in stronger intermolecular forces and interactions, which increases the potential energy.
- However, it is important to note that potential energy in this context is often considered negative because it represents the energy required to separate the molecules against these attractive forces.
4. Comparing Potential Energies:
- In a rarefied gas, the molecules are farther apart, resulting in weaker interactions and, consequently, a less negative potential energy.
- Thus, we can conclude:
PEcompressed<PErarefied (more negative)
5. Calculating Internal Energy:
- Since the kinetic energy remains constant for both states, the internal energy can be expressed as:
Ucompressed=KE+PEcompressed
Urarefied=KE+PErarefied
- Given that PEcompressed is more negative than PErarefied, it follows that:
Ucompressed<Urarefied
6. Conclusion:
- Therefore, the internal energy of a compressed gas is less than that of a rarefied gas at the same temperature due to the differences in potential energy arising from the distances between the molecules.
- Internal energy (U) of a gas is the sum of the kinetic energy (KE) and potential energy (PE) of its molecules.
- Mathematically, it can be expressed as:
U=KE+PE
- The kinetic energy of gas molecules is directly related to the temperature of the gas.
- Since both the compressed gas and the rarefied gas are at the same temperature, their average kinetic energy is the same.
- Therefore, we can say:
KEcompressed=KErarefied
- When a gas is compressed, the molecules are brought closer together.
- This results in stronger intermolecular forces and interactions, which increases the potential energy.
- However, it is important to note that potential energy in this context is often considered negative because it represents the energy required to separate the molecules against these attractive forces.
- In a rarefied gas, the molecules are farther apart, resulting in weaker interactions and, consequently, a less negative potential energy.
- Thus, we can conclude:
PEcompressed<PErarefied (more negative)
- Since the kinetic energy remains constant for both states, the internal energy can be expressed as:
Ucompressed=KE+PEcompressed
Urarefied=KE+PErarefied
- Given that PEcompressed is more negative than PErarefied, it follows that:
Ucompressed<Urarefied
- Therefore, the internal energy of a compressed gas is less than that of a rarefied gas at the same temperature due to the differences in potential energy arising from the distances between the molecules.
Topper's Solved these Questions
- SELF ASSESSMENT PAPER 02BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 02EXERCISE - SECTION - C (Answer the following )
8 Videos
- SELF ASSESSMENT PAPER 02BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 02EXERCISE - SECTION-D (Answer the following )
6 Videos
- SELF ASSESSMENT PAPER 02BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 02EXERCISE - SECTION - A(Answer the following )
7 Videos
- SELF ASSESSMENT PAPER 01BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 01EXERCISE - SECION- D (Answer the following )
6 Videos
- SELF ASSESSMENT PAPER 3BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 3EXERCISE - Section-D
6 Videos
Similar Questions
Knowledge Check
The compressibility of a gas is less than unity at STP. Therefore,
AVm>22.4LBVm<22.4LCVm=22.4LDVm=44.8L
The internal energy of non-ideal gas depends on
View SolutionThe internal energy of one mole mono-atomic gas is
View SolutionThe volume of gas A is twice than that of gas B. The compressibility factor of gas A is thrice than that of gas B at same temperature. The pressures of the gases for equal number of moles are:
View SolutionThe pressure of real gas is less than the pressure of an ideal gas because of
View SolutionIn the given graph, an ideal gas changes its state from A to C by two paths ABC and AC.
(a) Find the path along which work done is less.
(b) The internal energy of gas at A is 10J and the amount of heat supplied in path AC is 200J. Calculate the internal energy of gas at C.
(c) The internal energy of gas at state B is 20J. Find the amount of heat supplied to the gas to go from A to B.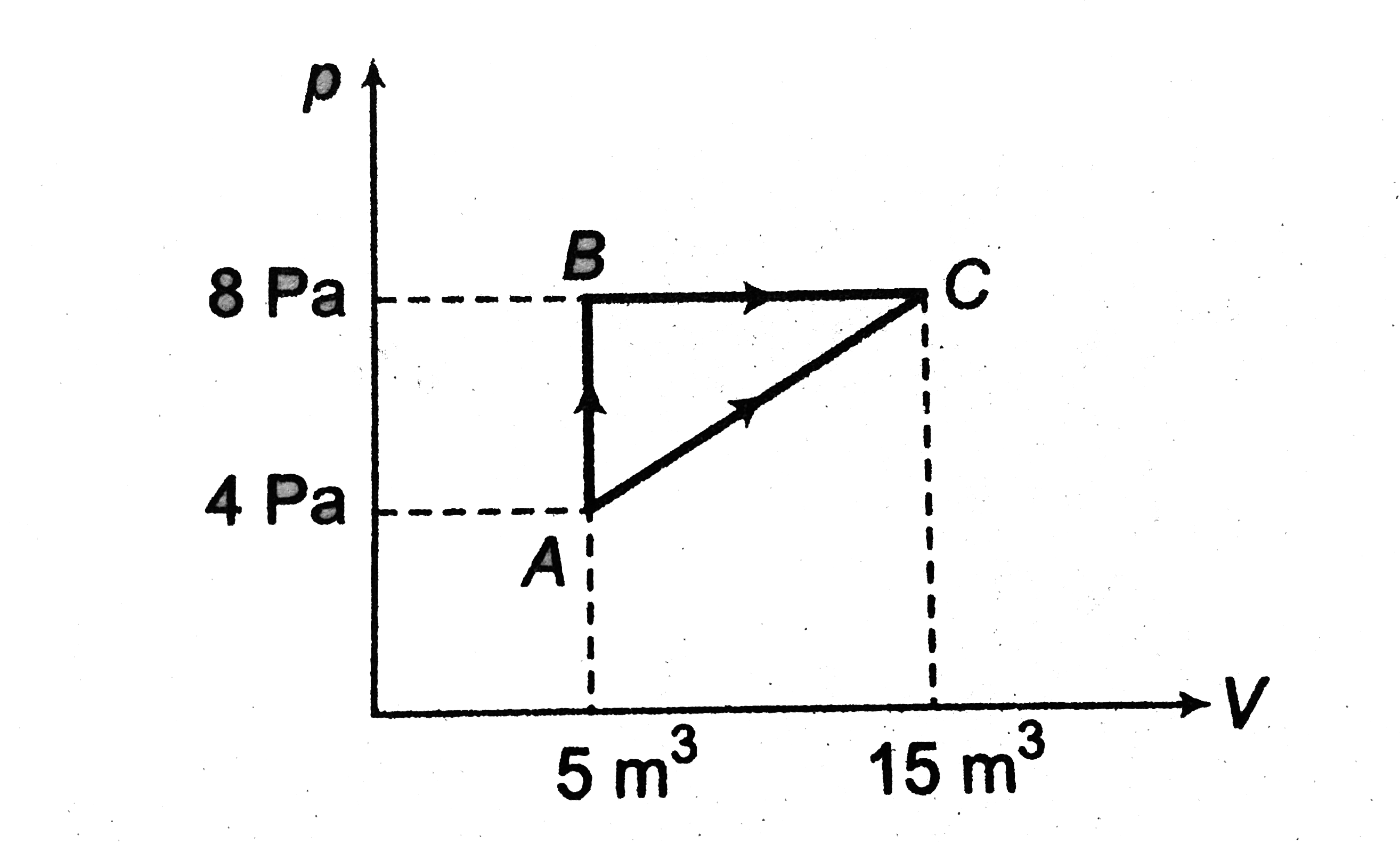
View SolutionThe kinetic energy of a molecule of a gas is directly proportional to the absolute temperature of the gas.
View SolutionIs the internal energy of a gas a function of the pressure? Explain.
View Solution
