A solid 'S'floats in liquid 'L'. The density of 'S' is ……………. than liquid 'L'.
To solve the question, we need to analyze the relationship between the densities of the solid 'S' and the liquid 'L' based on the fact that the solid is floating in the liquid.
1. Understanding the Concept of Density:
- Density is defined as mass per unit volume. An object will float in a fluid if its density is less than the density of the fluid.
2. Identifying the Scenario:
- The question states that a solid 'S' is floating in a liquid 'L'. This indicates that the solid is not sinking but rather remaining on the surface of the liquid.
3. Applying the Principle of Buoyancy:
- According to Archimedes' principle, an object will float if the buoyant force acting on it is equal to its weight. For this to happen, the density of the object must be less than that of the fluid.
4. Comparing Densities:
- Since solid 'S' is floating in liquid 'L', we conclude that the density of solid 'S' must be less than the density of liquid 'L'.
5. Filling in the Blank:
- Therefore, the correct statement to fill in the blank is: "The density of 'S' is less than liquid 'L'".
Final Answer:
The density of 'S' is less than liquid 'L'.
---
- Density is defined as mass per unit volume. An object will float in a fluid if its density is less than the density of the fluid.
- The question states that a solid 'S' is floating in a liquid 'L'. This indicates that the solid is not sinking but rather remaining on the surface of the liquid.
- According to Archimedes' principle, an object will float if the buoyant force acting on it is equal to its weight. For this to happen, the density of the object must be less than that of the fluid.
- Since solid 'S' is floating in liquid 'L', we conclude that the density of solid 'S' must be less than the density of liquid 'L'.
- Therefore, the correct statement to fill in the blank is: "The density of 'S' is less than liquid 'L'".
The density of 'S' is less than liquid 'L'.
Similar Questions
A solid floats in a liquid at 20∘C with 75 % of it immersed. When the liquid is heated to 100∘C, the same solid floats with 80% of it immersed in the liquid. Calculate the coefficient of expansion of the liquid. Assume the volume of the solid to be constant.
View SolutionAssertion : A solid floats in a liquid so that it is just submerged . When the liquid is heated the solid sinks to the bottom.
Reason : Weight of the solid increases with the rise in temperature .
View Solution
Knowledge Check
A body is just floating on the surface of a liquid. The density of the body is the same as that of the liquid. The body is slightly pushed down. What will happen to the body?
Acome back slowly to its earlier positionBremain submerged where it is leftCsink in liquidDcome out vigourslyA piece of solid weighs 120 g in air ,80 g in water and 60 kg in a liquid . The relative density of the solid and that of the liquid are respectively
A2,12B2,32C3,12D3,32
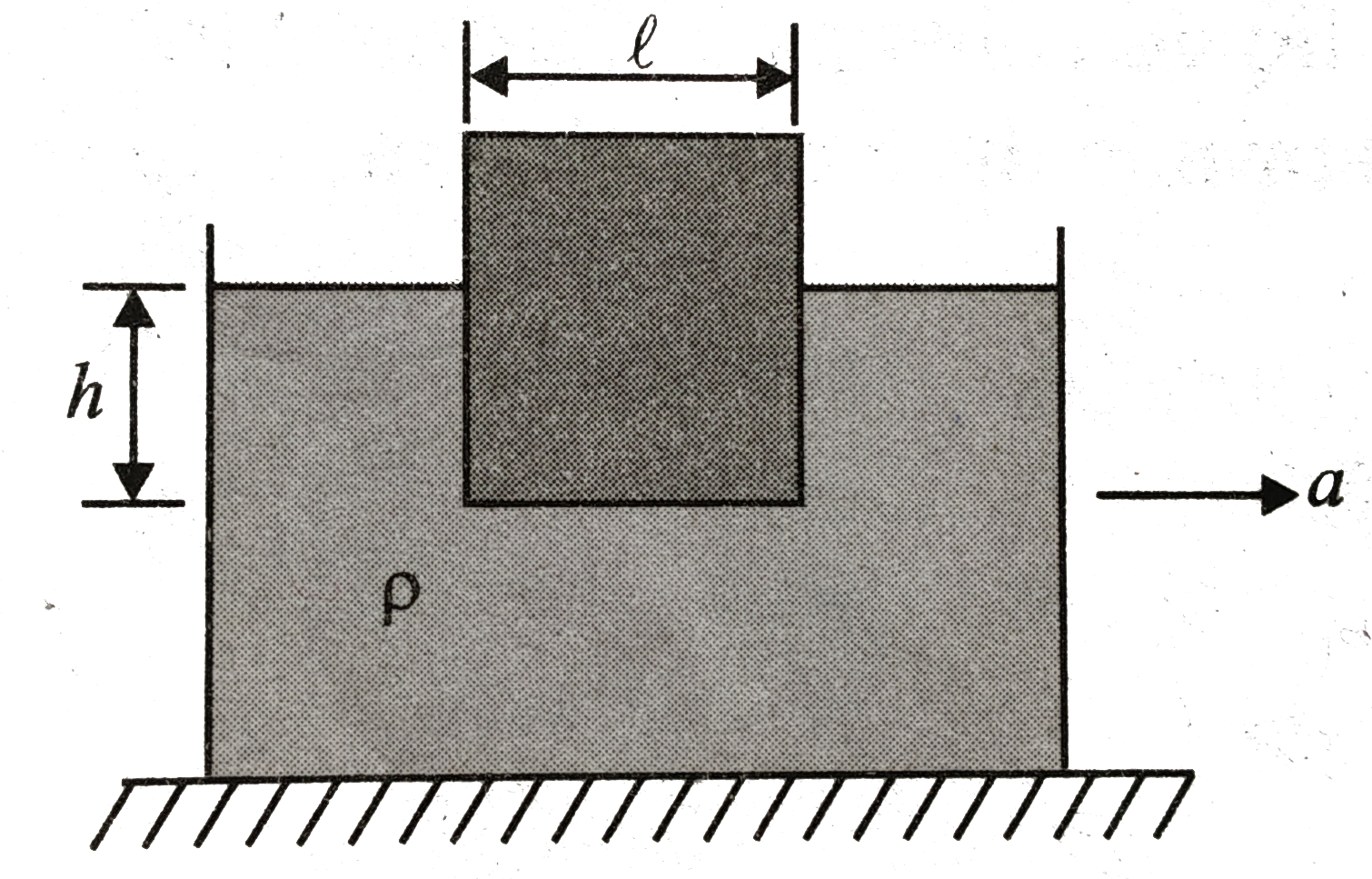
A wooden cube of length l and density a floats in a liquid of density p(>a). If the liquid moves with an acceleration in steady state, find the a. net hydrostatic force acting on the cube b. angle of inclination of the cub with horizontal.
View SolutionA solid floats in a liquid in a partially dipped position.
View SolutionAssertion : A solid is floating in a liquid of density ρ1. When the solid melts its density becomes ρ2 in liquid state. If ρ1>ρ2 level of liquids will increase after melting.
Reason : In liquid state volume always increases after a solid melts.
View SolutionA Solid sphere of density ρ=ρ0(1−r2R2),0<r≤R just floats in a liquid then density of liquid is– (r is distance from centre of sphere)
View SolutionA body floats in water with its one-third volume above the surface. The same body floats in a liquid with one-third volume immersed. The density of the liquid is
View SolutionA solid cube floats in a liquid of density ρ with half of its volume submerged as shown. The side length of the cube is a, which is very small compared to the size of the container. Now a liquid of density ρ3 (immiscible with the liquid already in the container) is poured slowly into the container. The column of the liquid of density ρ3 that must be poured so that the cube is fully submerged (with its top surface coincident with the surface of the poured liquid) is :

View Solution
