Question

A truck travels 25 km north and then 50 km in a direction ${60}^{\circ }$ west of north .Find the magnitude and direction of the truuck's resultant displacement .

Explanation

To solve the problem of finding the magnitude and direction of the truck's resultant displacement, we can follow these steps:

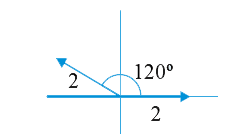
Step 1: Visualize the Problem
The truck travels 25 km north and then 50 km in a direction 60° west of north. We can represent this graphically on a coordinate system where north is the positive y-axis and east is the positive x-axis.

Step 2: Break Down the Movements into Components
1. The first movement is straightforward:
- Northward displacement (D1): \( D1 = 25 \, \text{km} \) (along the y-axis)
- Components: \( D1_x = 0 \, \text{km}, D1_y = 25 \, \text{km} \)

2. The second movement (D2) is 50 km at 60° west of north:
- We can break this down into x and y components using trigonometry:
- \( D2_x = -50 \sin(60^\circ) \) (negative because it is west)
- \( D2_y = 50 \cos(60^\circ) \)

Using the values of sine and cosine:
- \( \sin(60^\circ) = \frac{\sqrt{3}}{2} \)
- \( \cos(60^\circ) = \frac{1}{2} \)

Therefore:
- \( D2_x = -50 \cdot \frac{\sqrt{3}}{2} = -25\sqrt{3} \, \text{km} \)
- \( D2_y = 50 \cdot \frac{1}{2} = 25 \, \text{km} \)

Step 3: Calculate the Resultant Displacement Components
Now, we can find the total displacement components by adding the components from both movements:
- Total x-component:
\( R_x = D1_x + D2_x = 0 - 25\sqrt{3} \, \text{km} = -25\sqrt{3} \, \text{km} \)
- Total y-component:
\( R_y = D1_y + D2_y = 25 + 25 \, \text{km} = 50 \, \text{km} \)

Step 4: Calculate the Magnitude of the Resultant Displacement
Using the Pythagorean theorem, the magnitude of the resultant displacement \( R \) can be calculated as follows:
\( R = \sqrt{R_x^2 + R_y^2} = \sqrt{(-25\sqrt{3})^2 + (50)^2} \)
Calculating:
\( R = \sqrt{(625 \cdot 3) + 2500} = \sqrt{1875 + 2500} = \sqrt{4375} \approx 66.1 \, \text{km} \)

Step 5: Calculate the Direction of the Resultant Displacement
To find the direction (angle \( \alpha \)) of the resultant displacement from the north (y-axis), we use the tangent function:
\( \tan(\alpha) = \frac{R_x}{R_y} = \frac{-25\sqrt{3}}{50} \)
Calculating:
\( \tan(\alpha) = -\frac{\sqrt{3}}{2} \)
To find \( \alpha \):
\( \alpha = \tan^{-1}\left(-\frac{\sqrt{3}}{2}\right) \)
This angle is in the second quadrant since \( R_x \) is negative and \( R_y \) is positive. Thus, we can find the angle with respect to the north:
\( \alpha \approx 120^\circ \text{ (from the positive y-axis)} \)

Final Answer
- Magnitude of the resultant displacement: \( \approx 66.1 \, \text{km} \)
- Direction of the resultant displacement: \( \approx 120^\circ \) from the north.