Find the magnitude of the sum of $\to A and \to B$ and its direction with respect to the X - axis .

Text Solution

Verified by Experts

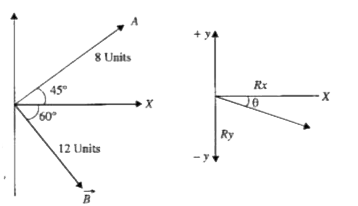
Resolving $\to A$ into two components $A_{x}$ along X - axis and $A_{y}$ along + Y - axis
$A_{x} = A cos 45 = 8 \times 0.707 = 5.656$ units
$A_{y} = A sin 45 = 8 \times 0.707 = 5.656$ units
The components of B along + X axis is $B_{x}$ and that along - Y axis is $B_{y}$
$B_{x} = B {cos 60}^{\circ} = 12 \times \frac{1}{2} = 6$ units
$B - (y) b sin 60 = 12 \times 0.086 = 10.392$ units
Let the resultant be $\to R \to A + \to B$
$∣ ∣ \to R ∣ ∣ = \sqrt{R_{x}^{2} + R_{y}^{2}}$
$R_{x} = A_{x} + B_{x} = 5.656 + 6 = 11.7$ units
$R_{y} = A_{y} - B_{y} = 5.656 - 10.392 = - 4.7$ units
Magnitude of resultant = $\sqrt{R_{x}^{2} + R_{y}^{2}}$
$= \sqrt{{11.7}^{2} + {(- 4.7)}^{2}} = 12.6$ units
$tan θ = \frac{R_{y}}{R_{x}} = \frac{- 4.7}{11.7} = - 0.4$
$θ = {21.8}^{\circ}$ .

Updated on:21/07/2023

|

Class 11 PHYSICS VECTOR

Topper's Solved these Questions

Similar Questions

Knowledge Check

The magnitude of x and y components of $\to A$ are 7 and 6 respectively . Also , the magnitudes of x and y components of $\to A + \to B$ are 11 and 9 respectively . Calculate the magnitude of vector $\to B$
A10
B5
C6
D3

ICSE-VECTORS SCALARS ELEMENTARY CALCULUS -ADDITIONAL SOLVED PROBLEMS

Home
Ask Doubt
Profile