What are the values of the following
→A×→A
The correct Answer is:0
To solve the problem of finding the value of →A×→A, we will utilize the properties of the cross product of vectors.
1. Understanding the Cross Product:
The cross product of two vectors →A and →B is given by the formula:
→A×→B=|→A||→B|sin(θ)^n
where θ is the angle between the two vectors, and ^n is the unit vector perpendicular to the plane formed by →A and →B.
2. Identifying the Vectors:
In this case, we are finding →A×→A. Here, both vectors are the same, meaning:
→A and →A are parallel.
3. Determining the Angle:
The angle θ between →A and →A is 0∘ because they are in the same direction.
4. Applying the Cross Product Formula:
Substituting θ=0∘ into the cross product formula:
→A×→A=|→A||→A|sin(0∘)^n
5. Calculating the Sine of Zero:
We know that:
sin(0∘)=0
Therefore:
→A×→A=|→A||→A|⋅0⋅^n=0
6. Conclusion:
Thus, the value of →A×→A is:
→A×→A=→0
Final Answer:
→A×→A=→0
The cross product of two vectors →A and →B is given by the formula:
→A×→B=|→A||→B|sin(θ)^n
where θ is the angle between the two vectors, and ^n is the unit vector perpendicular to the plane formed by →A and →B.
In this case, we are finding →A×→A. Here, both vectors are the same, meaning:
→A and →A are parallel.
The angle θ between →A and →A is 0∘ because they are in the same direction.
Substituting θ=0∘ into the cross product formula:
→A×→A=|→A||→A|sin(0∘)^n
We know that:
sin(0∘)=0
Therefore:
→A×→A=|→A||→A|⋅0⋅^n=0
Thus, the value of →A×→A is:
→A×→A=→0
→A×→A=→0
Topper's Solved these Questions
- VECTORS SCALARS ELEMENTARY CALCULUS BOOK - ICSECHAPTER - VECTORS SCALARS ELEMENTARY CALCULUS EXERCISE - UNSOLVED PROBLEMS
79 Videos
- VECTORS SCALARS ELEMENTARY CALCULUS BOOK - ICSECHAPTER - VECTORS SCALARS ELEMENTARY CALCULUS EXERCISE - FROM UNIT VECTORS COMPONENTS OF A VECTOR
6 Videos
- UNITSBOOK - ICSECHAPTER - UNITSEXERCISE - MODULE 3 (SELECTED PROBLEMS)
38 Videos
- WAVESBOOK - ICSECHAPTER - WAVESEXERCISE - From Musical Sound
10 Videos
Similar Questions
Knowledge Check
If →a and →b are unit vectors, then while of the following values of →a. →b is not possible ?
A1√2B−12C√32D√3
For any three vectors →a, →b and →c, write the value of the following: →a ×(→b+→c)+→b ×(→c+→a)+→c ×(→a+→b)
View SolutionFind the value of the following
∣∣→a.→b∣∣2+∣∣→a×→b∣∣2
View SolutionWhat is the value of (→A+→B).(→A×→B) ?
View SolutionFind the value of the following
∣∣→a.→b∣∣2−∣∣→a×→b∣∣2
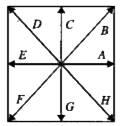
View SolutionA number of vectors are as shown in .They can be combined in various ways , like →A+→C=→B . What is the value of (a) →E+→C (b) →A+→F (c ) →A+→D (d) →E+→A (e ) →A−→B .
View SolutionIf →a,→b are two vectors, then write the truth value of the following statements: →a=−→b|→a|=∣∣→b∣∣ |→a|=∣∣→b∣∣→a=±→b |→a|=∣∣→b∣∣→a=→b
View SolutionIf →a,→b,→c are the position vectors of vertices A, B, C of a triangle ABC, Show that the area of the triangle is 12∣∣→b×→c+→c×→a+→a×→b∣∣
View Solution
