A car is running on a straight road . The distance travelled and time taken are connected by the formula =t2−2t where t is measured in hours and s in km. When the odometer reading is 15, what is the speedometer reading ? [ Odometer measures s and the speedmeter measures velocity]
To solve the problem step by step, we will follow the logic presented in the video transcript.
Step 1: Set up the equation
We are given the relationship between distance (s) and time (t) as:
s=t2−2t
Step 2: Substitute the odometer reading
We need to find the time (t) when the odometer reading (s) is 15 km. So we set:
15=t2−2t
Step 3: Rearrange the equation
Rearranging the equation gives us:
t2−2t−15=0
Step 4: Factor the quadratic equation
Now we factor the quadratic equation:
(t−5)(t+3)=0
Step 5: Solve for t
Setting each factor to zero gives us:
1. t−5=0 → t=5
2. t+3=0 → t=−3
Since time cannot be negative, we discard t=−3 and take:
t=5 hours
Step 6: Find the velocity
Next, we need to find the velocity (v) when t=5. The velocity is defined as the derivative of displacement with respect to time:
v=dsdt
Step 7: Differentiate the displacement function
We differentiate s=t2−2t:
dsdt=2t−2
Step 8: Substitute t into the velocity equation
Now we substitute t=5 into the velocity equation:
v=2(5)−2=10−2=8 km/h
Final Answer
Thus, when the odometer reading is 15 km, the speedometer reading is:
Speedometer reading=8 km/h
---
We are given the relationship between distance (s) and time (t) as:
s=t2−2t
We need to find the time (t) when the odometer reading (s) is 15 km. So we set:
15=t2−2t
Rearranging the equation gives us:
t2−2t−15=0
Now we factor the quadratic equation:
(t−5)(t+3)=0
Setting each factor to zero gives us:
1. t−5=0 → t=5
2. t+3=0 → t=−3
t=5 hours
Next, we need to find the velocity (v) when t=5. The velocity is defined as the derivative of displacement with respect to time:
v=dsdt
We differentiate s=t2−2t:
dsdt=2t−2
Now we substitute t=5 into the velocity equation:
v=2(5)−2=10−2=8 km/h
Thus, when the odometer reading is 15 km, the speedometer reading is:
Speedometer reading=8 km/h
Topper's Solved these Questions
- VECTORS SCALARS ELEMENTARY CALCULUS BOOK - ICSECHAPTER - VECTORS SCALARS ELEMENTARY CALCULUS EXERCISE - FROM SCALAR PRODUCT AND VECTOR PRODUCT
20 Videos
- UNITSBOOK - ICSECHAPTER - UNITSEXERCISE - MODULE 3 (SELECTED PROBLEMS)
38 Videos
- WAVESBOOK - ICSECHAPTER - WAVESEXERCISE - From Musical Sound
10 Videos
Similar Questions
The position of a particle is given by the equation f(t)=t3−6t2+9t where t is measured in second and s in meter. Find the acceleration at time t. What is the acceleration at 4 s?
View SolutionIn S=a+bt+ct2. S is measured in metres and t in seconds. The unit of c is
View SolutionThe distance travelled by a particle in a straight line motion is directly proportional to t1/2, where t is the time elapsed.
View SolutionThe position of a particle is given by →r=(8tˆi+3t2+5ˆk) m where t is measured in second and →r in meter. Calculate,
the magnitude of velocity at t = 5 s,
View SolutionCheck the correctness of the formula S=ut+13at2 where S is the distance , u is velocity , a is acceleration and t is time.
View SolutionA particle is moving in a straight line such that its distance s at any time t is given by s=t44−2t3+4t2−7. Find when its velocity is maximum
View SolutionA car is moving on a straight road. The velocity of the car varies with time as shown in the figure. Initially (at t=0), the car was at x=0, where, x is the position of the car at any time 't'.
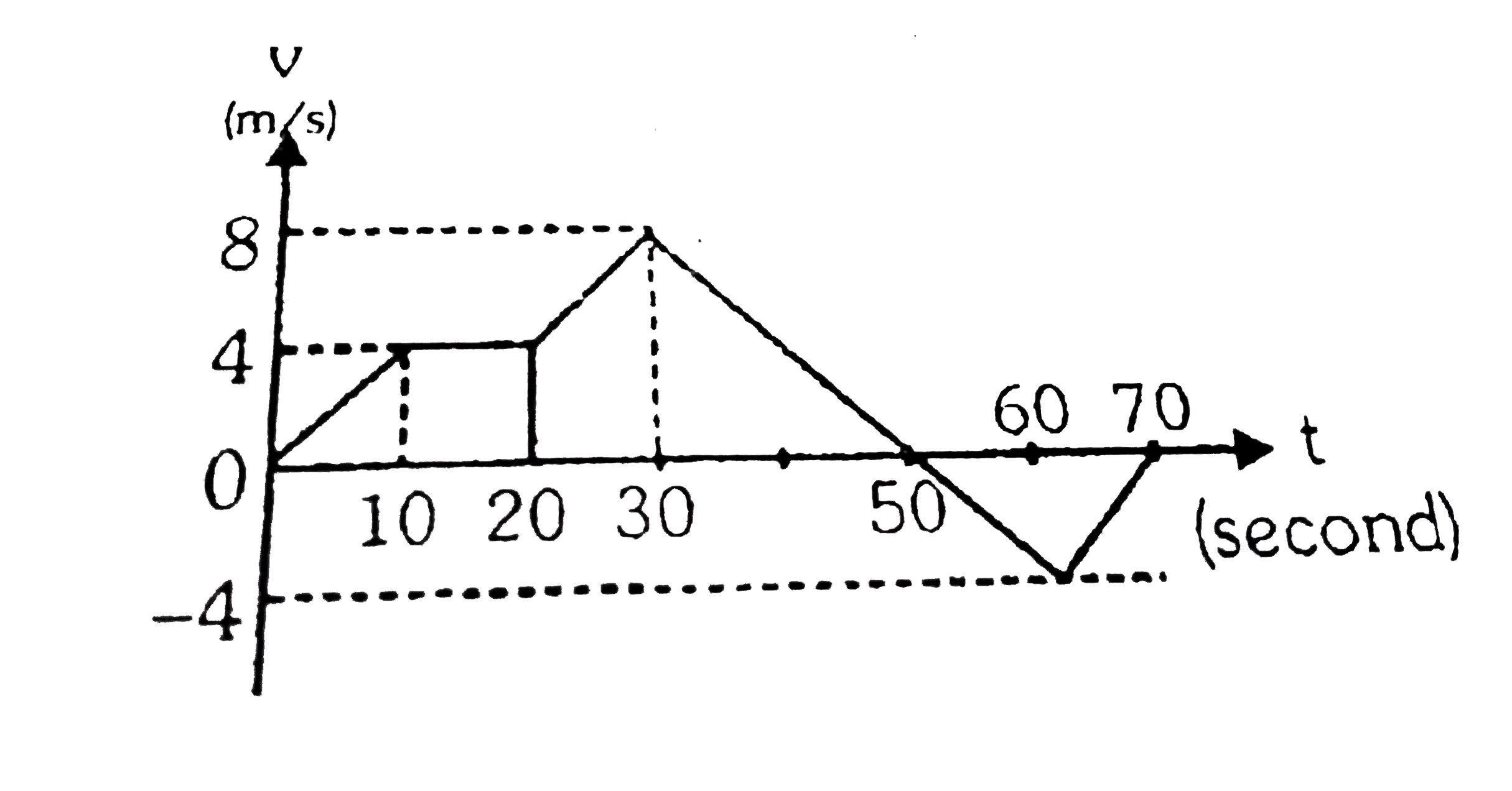
The time interval during which the car is retarding can be :-
View SolutionA particle moving in a straight line has its velocit varying with time according to relation v=t2−6t+8(m/s) where t is in seconds. The CORRECT statement(s) about motion of this particle is/are:-
View SolutionThe distance travelled by a motor car in t second after the brakes are applied is s feet, where s = 22t −12t2. The distance travelled by the car before it stops, is
View SolutionA particle moves along the positive branch of the curve y=x22 where x=t22,x and y are measured in metres and t in second. At t=2s, the velocity of the particle is
View Solution
